3.3.4: Characteristic curves in wings

Last updated
Save as PDF

Page ID: 78110

\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \) \( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)\(\newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\) \( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\) \( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\) \( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\) \( \newcommand{\Span}{\mathrm{span}}\) \(\newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\) \( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\) \( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\) \( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\) \( \newcommand{\Span}{\mathrm{span}}\)\(\newcommand{\AA}{\unicode[.8,0]{x212B}}\)

The curve of lift and the drag polar permit knowing the aerodynamic characteristics of the aircraft.

Lift curve

The coefficient of lift depends, in general, on the angle of attack, Mach and Reynolds number, and the aircraft configuration (flaps, see Section 3.4). The most general expression is:

\[C_L = f(\alpha , M, \text{Re}, configuration).\]

As in airfoils (under the same hypothesis of incompressible flow), in wings typically the lift curve presents a linear zone, which can be approximated by:

\[C_L (\alpha) = C_{L0} + C_{L\alpha} \alpha = C_{L \alpha} (\alpha - \alpha_0),\]

where \(C_{L \alpha} = d C_L/d \alpha\) is the slope of the lift curve, \(C_{L0}\) is the value of \(C_L\) for \(\alpha = 0\) and \(\alpha_0\) is the value of \(\alpha\) for \(C_L = 0\). There is a point at which the linear behavior does not hold anymore, whose angle is referred to as stall angle. At this angle the curve presents a maximum. Once this angle is past, lift decreases dramatically.

According to Prandtl theory of large wings, the slope of the curve is:

\[C_{L \alpha} = \dfrac{d C_L}{d\alpha} = \dfrac{c_{l\alpha} e}{1 + \tfrac{c_{l\alpha}}{\pi A} },\]

where \(e \le 1\) is an efficiency form factor of the wing, also referred to as Oswald factor. In elliptic plantform \(e = 1\).

Drag polar

The aircraft’s drag polar is the function relating the coefficient of drag with the coefficient of lift, as mentioned for airfoils.

The coefficient of drag depends, in general, on the coefficient of lift, Mach, and Reynolds number, and the aircraft configuration (flaps, see Section 3.4). The most general expression is:

\[C_D = f(C_L, M, \text{Re}, configuration).\]

The polar can be approximated to a parabolic curve of the form:

\[C_D (C_L) = C_{D_0} + C_{D_i} C_L^2,\]

where \(C_{D_0}\) is the parasite drag coefficient (the one that exists when \(C_L = 0\)) due to friction

and pressure effects in the wing, fuselage, etc., and \(C_{D_i} = \tfrac{1}{\pi Ae}\) is the induced coefficient (drag induced by lift) fundamentally due to the induced velocity and the whirlwind trail. This curve is referred to as parabolic drag polar. The typical values of \(C_{D_0}\) depend on the aircraft but are approximately 0.015 - 0.030 and the parameter of aerodynamic efficiency e can be approximately 0.75 - 0.85.

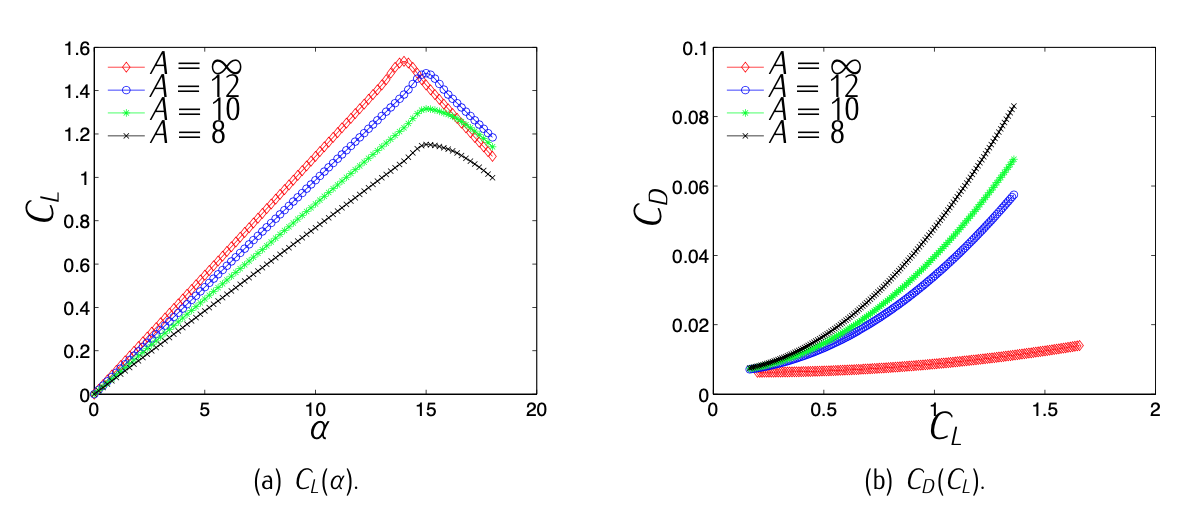
截屏2022-01-15 下午3.30.02.png
Figure 3.25: Characteristic curves in wings.

The lift curve (\(C_L - \alpha\)) and the drag ploar (\(C_D - C_L\)) are represented in Figure 3.25 for a wing with four different enlargements. Both the slope and the maximum value of the lift curves increase when the enlargement increases. For the polar case, it can be observed how drag reduces as the enlargement increases.