6.4: Real Gases
- Page ID
- 7506
In reality, no gas behaves ideally. Therefore, the ideal EOS is not useful for practical applications, although it is important as the basis of our understanding of gas behavior. Even though the ideal model is not reliable for real engineering applications, we have to keep in mind that the ideal gas EOS is the starting point of all modern approaches.
If we look back at Figure 6.3.2 and recall our discussions about P-v behavior of pure substances, something should catch your attention. Figure 6.4.3 shows us the typical P-v behavior of a pure substance to facilitate our discussion.
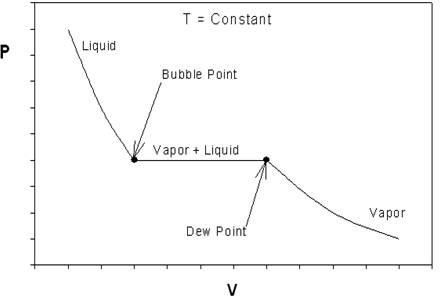
What can we conclude about the ideal EOS while contrasting Figure 6.3.2 to 6.4.3? The curve in Figure 6.3.2 is continuous; Figure 6.4.3 has an obvious discontinuity (at the vapor+liquid transition). Hence, one thing we can already say is that the ideal EOS is at least qualitatively wrong. For a real substance, as pressure increases, there must be a point of discontinuity that represents the phase change. Ideal gas will not condense, no matter what pressure it is subjected to, regardless of the temperature of the system. In other words, we cannot hope to reproduce the P-v behavior of Figure 6.4.3 using the ideal equation (\(6.3.3\)) since no discontinuity is to be found. However, the real P-v isotherm can be approximated by ideal behavior at low pressures, as we can see from the plots.
We can also establish some quantitative differences between ideal and real PVT behavior. For example, for most conditions of interest at a given volume and temperature, the ideal gas model over-predicts the pressure of the system:
\[ P_{Ideal\,Gas} > P_{Real\,Gas} \]
We can explain this difference by recalling that a real gas does have interaction forces between molecules. Secondly, we recall that the concept of “pressure” of a gas is a consequence of the number of molecular collisions per unit area against the wall of the container. Such number of collisions is, in turn, a measure of the freedom of the molecules to travel within the gas. The ideal gas is a state of complete molecular freedom where molecules do not even know the existence of the others. Hence, a hypothetical ideal gas will exert a higher pressure than a real gas at any given volume and temperature. When molecules come together (real gas), it reduces the available free space for the molecules and pressure is reduced.
Additionally, the ideal model assumes that the physical space that the molecules themselves occupy is negligible. In reality molecules are physical particles and they do occupy space. Once we find a way of accounting for the space that the molecules themselves occupy, we would be able to compute a “real” free volume available for the molecules to travel through the gas. In the ideal case, this free volume is equal to the volume of the container itself since molecular volume is not accounted for. In the real case, this free volume must be less than the volume of the container itself after we account for the physical space that molecules occupy. Therefore:
\[ V_{real, free} < V_{Ideal} = V_{Container} \]
\[ V_{real, free} < V_{Container} = V_{occupied\,by\,molecules}\]


