7.3: Purpose and Historical Development
- Page ID
- 450
We want to use EOS as the basis for generating data: volumetric data, thermophysical data, and to help us perform vapor/liquid equilibrium (VLE) calculations. Probably there has not been an area of thermodynamics to which so many hours of research have been devoted as that of the topic of EOS. Among the properties derived from an EOS include:
- Densities (vapor and liquid),
- Vapor pressures of pure components,
- Critical pressures and temperatures for the mixture,
- Vapor-Liquid equilibrium (VLE) information,
- Thermodynamic properties (ΔH, ΔS, ΔG, ΔA).
300 years of EOS Development
PERIOD 1: “Foundational work”
Before 1662, there was an incomplete understanding and qualitative representation of the volumetric behavior of gases.
1662: First breakthrough — Boyle’s Law. Boyle did not define an ideal behavior. When he proposed this law, he was convinced that it applied to all gases. Among the limitations that Boyle was working under: no high pressure measurements could have been taken using the equipment of his time, and his working fluid was air. Hence, it is no wonder that everything these pioneers did pertained to what we now recognize as the ideal state.
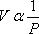
PV =constant
1787: Charles’ Law. It was one hundred plus years until a new, important development in the gas behavior field. Charles postulated that the volume of a gas is proportional to its temperature at isobaric conditions.
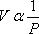
Combining, PV/T = constant = R, gas constant
1801: Dalton introduced the concept of partial pressures and recognized that the total pressure of a gas is the sum of the individual (partial) contributions of its constituents.
1802: Gay-Lussac. He helped to define the universal gas constant “R”. Dalton had looked at different gases and calculated the ratio PV/T to verify that it was constant. However, it was believed that each gas may have its own R. Gay-Lussac showed that a single constant applied to all gases, and calculated the “universal” gas constant.
1822: Cagniard de la Tour. He discovered the critical state (critical point) of a substance.
1834: Clapeyron. He was the first to suggest PV=R(T+273).
PERIOD 2: “Monumental Work”
Period of turning points and landmarks with quantitative developments.
1873: van der Waals. With van der Waals, a quantitative approach was taken
for the first time. He was an experimentalist and proposed the continuity of gases and liquid that won for him a Nobel Prize. He has provided the most important contribution to EOS development.
1875: Gibbs, an American mathematical physicist, made the most important contributions to the thermodynamics of equilibrium in what has been recognized as a monumental work.
1901: Onnes theoretically confirmed the critical state.
1902: Lewis defined the concept of fugacity.
1927: Ursell proposed a series solution (polynomial functional form) for EOS: P = 1 + b/V + c/V2 + d/V3 +… This is known as the virial EOS. Virial EOS has better theoretical foundation than any other. However, cubic EOS (as vdW’s) need only 2 parameters and have become more widespread in use.
PERIOD 3: “Incremental Improvement”
During this last and current period, a better quantitative description of volumetric behavior has been achieved at a rather low pace. What is striking, as we will study later, is that most of the tools of most critical use for us today are based on the works of van der Waals, Gibbs, and Lewis, and have been around for years.
1940: Benedit, Webb, & Rubbin proposed what can be called the “Cadillac” of EOS, i.e., the most sophisticated and most accurate for some systems. However, the price to pay is that it is complicated and not easy to use.
1949: Redlich & Kwong introduced a temperature dependency to the attraction parameter “a” of the vdW EOS.
1955: Pitzer introduced the idea of the “acentric factor” to quantify the non-sphericity of molecules and was able to relate it to vapor pressure data.
1972: Soave modified the RK EOS by introducing Pitzer’s acentric factor.
1976: Peng and Robinson proposed their EOS as a result of a study sponsored by the Canadian Gas Commission, in which the main goal was finding the EOS best applicable to natural gas systems.
Since then, there has not been any radical improvement to SRK and PR EOS, although a great deal of work is still underway.
Contributors and Attributions
Prof. Michael Adewumi (The Pennsylvania State University). Some or all of the content of this module was taken from Penn State's College of Earth and Mineral Sciences' OER Initiative.


