4.6.1: Stability
- Page ID
- 687
Figure 4.37 shows a body made of hollow balloon and a heavy sphere connected by a thin and light rod. This arrangement has mass centroid close to the middle of the sphere. The buoyant center is below the middle of the balloon. If this arrangement is inserted into liquid and will be floating, the balloon will be on the top and sphere on the bottom. Tilting the body with a small angle from its resting position creates a shift in the forces direction (examine Figure 4.37b). These forces create a moment which wants to return the body to the resting (original) position. When the body is at the position shown in Figure 4.37c, the body is unstable and any tilt from the original position creates moment that will further continue to move the body from its original position. This analysis doesn't violate the second law of thermodynamics. Moving bodies from an unstable position is in essence like a potential.

Fig. 4.38. Schematic of floating cubic.
A wooden cubic (made of pine, for example) is inserted into water. Part of the block floats above water line. The cubic mass (gravity) centroid is in the middle of the cubic. However the buoyant center is the middle of the volume under the water (see Figure 4.38). This situation is similar to Fig. 4.37c. However, any experiment of this cubic wood shows that it is stable locally. Small amount of tilting of the cubic results in returning to the original position. When tilting a larger amount than \(\pi/4\), it results in a flipping into the next stable position. The cubic is stable in six positions (every cubic has six faces). In fact, in any of these six positions, the body is in situation like in 4.37c. The reason for this local stability of the cubic is that other positions are less stable. If one draws the stability (later about this criterion) as a function of the rotation angle will show a sinusoidal function with four picks in a whole rotation.
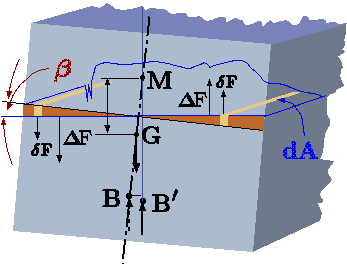
Fig. 4.39. Stability analysis of floating body.
So, the body stability must be based on the difference between the body's local positions rather than the ``absolute'' stability. That is, the body is ``stable'' in some points more than others in their vicinity. These points are raised from the buoyant force analysis. When the body is tilted at a small angle, \(\beta\), the immersed part of the body center changes to a new location, \(B'\) as shown in Figure. 4.39. The center of the mass (gravity) is still in the old location since the body did not change. The stability of the body is divided into three categories. If the new immerse volume creates a new center in such way that couple forces (gravity and buoyancy) try to return the body, the original state is referred as the stable body and vice versa. The third state is when the couple forces do have zero moment, it is referred to as the neutral stable. The body, shown in Figure 4.39, when given a tilted position, move to a new buoyant center, \(B'\). This deviation of the buoyant center from the old buoyant center location, \(B\), should to be calculated. This analysis is based on the difference of the displaced liquid. The right green area (volume) in Figure 4.39 is displaced by the same area (really the volume) on left since the weight of the body didn't change so the total immersed section is constant. For small angle, \(\beta\), the moment is calculated as the integration of the small force shown in the Fig. 4.39 as \(\delta F\). The displacement of the buoyant center can be calculated by examining the moment these forces creates. The body weight creates opposite moment to balance the moment of the displaced liquid volume. \[\overline{BB'}W = M\] Where \(M\) is the moment created by the displaced areas (volumes), \(\overline{BB'}\) is the distance between points \(B\) and point \(B'\), and \(W\) refers to the weight of the body. It can be noticed that the distance \(\overline{BB'}\) is an approximation for small angles (neglecting the vertical component). So the perpendicular distance, \(\overline{BB'}\), should be \[\overline{BB'} = \frac{M}{W}\] The moment \(M\) can be calculated as \[M = \int_{A} g\rho_{l}x\beta dA x = g \rho_{l} \beta \int_{A} x^{2} dA \] The integral in the right side of equation 36 is referred to as the area moment of inertia and was discussed in Chapter 3. The distance, \(\overline{BB'}\) can be written from equation 36 as \[\overline{BB'} = \frac{g\rho_{l}I_{xx}}{\rho_{s}V_{body}}\] The point where the gravity force direction is intersecting with the center line of the cross section is referred as metacentric point, \(M\). The location of the metacentric point can be obtained from the geometry as \[\overline{BM} = \frac{\overline{BB'}}{sin\beta}\] And combining equations 37 with 38 yields \[\overline{BM} = \frac{\not{g}\rho_{l}\beta I_{xx}}{\not{g}\rho_{s}sin\beta V_{body}} = \frac{\rho_{l} I_{xx}}{\rho_{s}V_{body}}\] For small angle \(\left(\beta \sim 0\right) \[lim_{\beta \rightarrow 0} \frac{sin \beta}{\beta} \sim 1 \] It is remarkable that the results is independent of the angle. Looking at Figure 4.39, the geometrical quantities can be related as \[\overline{GM} = \frac{\rho_{l} I_{xx}}{\rho_{s}V_{body}} - \overline{BG}\]
Example 4.28
A solid cone floats in a heavier liquid (that is \(\rho_l/\rho_c> 1\)). The ratio of the cone density to liquid density is \(\alpha\). For a very light cone \(\rho_{c}/\rho_{l} \sim 0\), the cone has zero depth. At this condition, the cone is unstable. For middle range, \(1 > \rho_{c}/\rho_{l} > 0\) there could be a range where the cone is stable. The angle of the cone is \(\theta\). Analyze this situation.
Solution 4.28
The floating cone volume is \(\dfrac{\pi\,d\,r^2}{3}\) and the center of gravity is D/4. The distance \(\overline{BG}\) depend on \(d\) as
\[
\label{coneStability:BG}
\overline{BG} = D/4 - d/4
\]
\[
\label{coneStability:d-r}
r = d\,\tan\theta
\]
\[
\label{coneStability:GMini}
\overline{GM} =
\dfrac{\rho_l\,\overbrace{\dfrac{\pi\,\left( d\,\tan\theta\right)^4}{64}} ^{I_{xx}}}{\rho_{s}
\underbrace{\dfrac{\pi\,d\,\left( d\,\tan\theta\right)^2}{3} }_{V_{body}} }
- \overbrace{\left(\dfrac{D}{4} - \dfrac{d}{4} \right)}^{\overline{BG}}
\]
Equation (44) can be simplified as
\[
\label{coneStability:GM}
\overline{GM} =
\dfrac{\rho_l\,d\, \tan^2\theta }{\rho_{s}\,192}
- \left(\dfrac{D}{4} - \dfrac{d}{4} \right)
\]
The relationship between \(D\) and \(d\) is determined by the density ratio (as displaced volume
\[
\label{coneStability:d-D}
\rho_l\,d^3 = \rho_c\, D^3 \Longrightarrow D = d \sqrt[3]{\dfrac{\rho_l}{\rho_c}}
\]
Substituting equation (46) into (??) yield the solution when \(\overline{GM} = 0 \)
\[
\label{coneStability:sol}
0 = \dfrac{\rho_l\,d\, \tan^2\theta }{\rho_{s}\,192}
- \left(\dfrac{d \sqrt[3]{\dfrac{\rho_l}{\rho_c}}}{4} - \dfrac{d}{4} \right) \Longrightarrow
\dfrac{\rho_l\, \tan^2\theta} {\rho_{s}\,48} = \sqrt[3]{\dfrac{\rho_l}{\rho_c} - 1 }
\]
Since \(\rho_l > \rho_c\) this never happened.
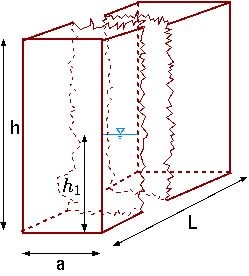
Fig. 4.40. Cubic body dimensions for stability analysis.
To understand these principles consider the following examples.
Example 4.29
A solid block of wood of uniform density, \(ρ_s = α\, ρ_l\) where (\(0\leα\le1\)) is floating in a liquid. Construct a graph that shows the relationship of the \(\overline{GM}\) as a function of ratio height to width. Show that the block's length, \(L\), is insignificant for this analysis.
Solution 4.29
Equation (41) requires that several quantities should be expressed. The moment of inertia for a block is given in Table ?? and is \(I_{xx}= \dfrac{La^3}{12}\). Where \(L\) is the length into the page. The distance \(\overline{BG}\) is obtained from Archimedes' theorem and can be expressed as
\begin{align*}
W = \rho_s \,\overbrace{a\,h\,L}^{V} =
\rho_l \,\overbrace{a\,h_1\,L}^{\text{immersed<br>volume} }
\Longrightarrow h_1 = \dfrac{\rho_s}{\rho_l} h
\end{align*}
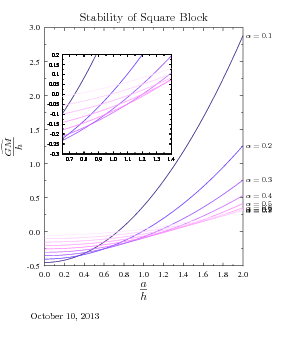
Fig. 4.41 Stability of cubic body infinity long.
Thus, the distance \(\overline{BG}\) is (see Figure 4.38)
\begin{align*}
\overline{BG} = \dfrac{h}{2} -
\overbrace{\dfrac{\rho_s}{\rho_l}\, h}^{h_1}\,\dfrac{1}{2}
= \dfrac{h}{2} \left(1 - \dfrac{\rho_s}{\rho_l} \right)
\label{static:eq:BGbar}
\end{align*}
\begin{align*}
GM = \dfrac{\cancel{g}\,\rho_l\,
\overbrace{\dfrac{\cancel{L}\,a^3}{12}}^{I_{xx}} }
{\cancel{g}\,\rho_s\,\underbrace{a\,h\,\cancel{L}}_V}
- \dfrac{h}{2} \left(1 - \dfrac{\rho_s}{\rho_l} \right)
\end{align*}
Simplifying the above equation provides
\begin{align*}
\dfrac{\overline{GM}}{h} = \dfrac{1}{12\,\alpha}
\left(\dfrac{a}{h}\right)^2
- \dfrac{1}{2} \left( 1 - \alpha \right)
\end{align*}
<a name='sub' ></a> </tt>
Notice that \(\overline{GM}/{h}\) isn't a function of the depth, \(L\). This equation leads to the condition where the maximum height above which the body is not stable anymore as
\begin{align*}
\dfrac{a}{h} \ge \sqrt
Callstack:
at (Bookshelves/Civil_Engineering/Book:_Fluid_Mechanics_(Bar-Meir)/04:_Fluids_Statics/4.6:_Buoyancy_and_Stability/4.6.1:_Stability), /content/body/div[4]/p[5]/span, line 1, column 2
\label{static:eq:stabilityCritieriaCubic}
\end{align*}
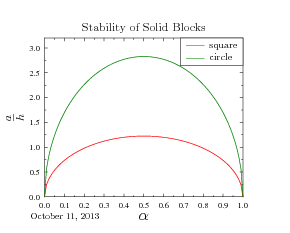
Fig. 4.42. The maximum height reverse as a function of density ratio.
One of the interesting point for the above analysis is that there is a point above where the ratio of the height to the body width is not stable anymore. In cylindrical shape equivalent to equation __?__ can be expressed. For cylinder (circle) the moment of inertia is \(I_{xx} = \pi b^{4} / 64\). The distance \(\overline{BG}\) is the same as for the square shape (cubic) (see above). Thus, the equation is \[\frac{\overline{GM}}{h} = \frac{g}{64\alpha}\left(\frac{b}{h}\right)^{2} - \frac{1}{2}\left(1 - \alpha\right)\] And the condition for maximum height for stability is \[\frac{b}{h} \ge \sqrt{32\left(1-\alpha\right)\alpha}\] This kind of analysis can be carried for different shapes and the results are shown for these two shapes in Figure 4.42. It can be noticed that the square body is more stable than the circular body shape.
Principle Main Axises
Any body has infinite number of different axes around which moment of inertia can be calculated. For each of these axes, there is a different moment of inertia. With the exception of the circular shape, every geometrical shape has an axis in which the moment of inertia is without the product of inertia. This axis is where the main rotation of the body will occur. Some analysis of floating bodies are done by breaking the rotation of arbitrary axis to rotate around the two main axes. For stability analysis, it is enough to find if the body is stable around the smallest moment of inertia. For example, a square shape body has larger moment of inertia around diagonal. The difference between the previous calculation and the moment of inertia around the diagonal is \[\delta I_{xx} = \frac{\sqrt{2}a\left(\frac{\sqrt{3}a}{2}\right)^{3}}{6} - \frac{a^{4}}{12} \sim 0.07a^{4}\] Which show that if the body is stable at main axes, it must be stable at the ``diagonal'' axis. Thus, this problem is reduced to find the stability for principle axis.
Unstable Bodies
What happen when one increases the height ratio above the maximum height ratio? The body will flip into the side and turn to the next stable point (angle). This is not a hypothetical question, but rather practical. This happens when a ship is overloaded with containers above the maximum height. In commercial ships, the fuel is stored at the bottom of the ship and thus the mass center (point \(G\) ) is changing during the voyage. So, the ship that was stable (positive \(\overline{GM}\) ) leaving the initial port might became unstable (negative \(\overline{GM}\) ) before reaching the destination port.
Fig. 4.43 Stability of two triangles put tougher.
Contributors and Attributions
Dr. Genick Bar-Meir. Permission is granted to copy, distribute and/or modify this document under the terms of the GNU Free Documentation License, Version 1.2 or later or Potto license.


