5.7: More Examples for Mass Conservation
- Page ID
- 708
Typical question about the relative velocity that appeared in many fluid mechanics exams is the following.
Example 5.14
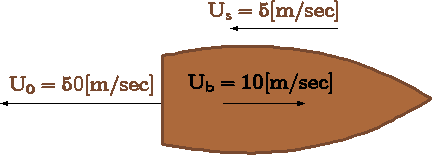
Fig. 5.12 Schematic of the boat for example .
The inboard engine uses a pump to suck in water at the front \(A_{in} = 0.2\, m^2\) and eject it through the back of the boat with exist area of \(A_{out}=0.05\,m^2\). The water absolute (relative to the ground) velocity leaving the back is 50\(m/sec\), what are the relative velocities entering and leaving the boat and the pumping rate?
Solution 5.14
The boat is assumed (implicitly is stated) to be steady state and the density is constant. However, the calculations have to be made in the frame of reference moving with the boat. The relative jet discharge velocity is
\begin{align*}
U_{r_{out}} = 50 + 10 = 60[m/sec]
\end{align*}
The volume flow rate is then
\begin{align*}
Q_{out} = A_{out} \,U_{r_{out}} = 60\times 0.05 = 3 m^3/sec
\end{align*}
The flow rate at entrance is the same as the exit thus,
\begin{align*}
U_{r_{in}} = \dfrac{A_{out}}{A_{in}} \,U_{r_{out} } = \dfrac{0.05}{0.2}{60} = 15.0 m/sec
\end{align*}
In this case (the way the question is phrased), the velocity of the river has no relavence.
Example 5.15
The boat from Example 5.14 travels downstream with the same relative exit jet speed (60\(m/s\)). Calculate the boat absolute velocity (to the ground) in this case, assume that the areas to the pump did not change. Is the relative velocity of the boat (to river) the same as before? If not, calculate the boat reletive velocity to the river. Assume that the river velocity is the same as in the previous example.
Solution 5.15
The relative exit velocity of the jet is 15\(m/sec\) hence the flow rate is
\begin{align*}
Q_{out} = A_{out} \,U_{r_{out}} = 60\times 0.05 = 3 m^3/sec
\end{align*}
The relative (to the boat) velocity into boat is same as before (15\(m/sec\)). The absolute velocity of boat (relative to the ground) is
\begin{align*}
U_{boat} = 15+ 5 = 20 m/sec
\end{align*}
The relative velocity of boat to the river is
\begin{align*}
U_{\text{relative to river} } = 20-5 = 15 m/sec
\end{align*}
The relative exit jet velocity to the ground
\begin{align*}
U_{in} = 60 - 15 = 45 m/sec
\end{align*}
The boat relative velocities are different and depends on the directions.
Example 5.16
Liquid A enters a mixing device depicted in at 0.1 [\(kg/s\)]. In same time liquid B enter the mixing device with a different specific density at 0.05 [\(kg/s\)]. The density of liquid A is 1000[\(kg/m^3\)] and liquid B is 800[\(kg/m^3\)]. The results of the mixing is a homogeneous mixture. Assume incompressible process. Find the average leaving velocity and density of the mixture leaving through the 2O [\(cm\)] diameter pipe. If the mixing device volume is decreasing (as a piston pushing into the chamber) at rate of .002 [\(m^3/s\)], what is the exit velocity? State your assumptions.
Solution 5.16
In the first scenario, the flow is steady state and equation (??) is applicable
\[
\label{mixChamber:gov}
\dot{m}_{A} + \dot{m}_B = Q_{mix}\,\rho_{mix} \Longrightarrow = 0.1 + 0.05 = 0.15 [m]
\]
\begin{align*}
\nonumber
\dot{Q}_A + \dot{Q}_B = \dot{Q}_{mix}
\Longrightarrow = \dfrac{\dot{m}_{A}}{\rho_A} + \dfrac{\dot{m}_{A}}{\rho_A} =
\dfrac{0.10}{1000} + \dfrac{0.05}{800}
\end{align*}
Thus the mixture density is
\[
\label{mixChamber:rhoMix}
\rho_{mix} = \dfrac{\dot{m}_{A} + \dot{m}_B }{ \dfrac{\dot{m}_{A}}{\rho_A} + \dfrac{\dot{m}_{B}}{\rho_B}}
= 923.07 [kg/m^3]
\]
The averaged velocity is then
\[
\label{mixChamber:Umix}
U_{mix} = \dfrac{Q_{mix}}{A_{out}} =
\dfrac{ \dfrac{\dot{m}_{A}}{\rho_A} + \dfrac{\dot{m}_{B}} {\rho_B} } {\pi\,0.01^2}
= \dfrac{1.625}{\pi } [m/s]
\]
In the case that a piston is pushing the exit density could be changed and fluctuated depending on the location of the piston. However, if the assumption of well mixed is still holding the exit density should not affected. The term that should be added to the governing equation the change of the volume. So governing equation is (??).
\[
\label{mixChamber:deformable}
\overbrace{U_{bn}\,A\,\rho_{b}}^{-Q_b\,\rho_{mix}}
= \overbrace{\dot{m}_A + \dot{m}_B}^{in} - \overbrace{\dot{m}_{mix}}^{out}
\]
That is the mixture device is with an uniform density
\[
\label{mixChamber:defCV}
- 0.002 [m^/sec]\,923.7[kg/m^3] = 0.1 + 0.05 - m_{exit}
\]
\[
m_{exit} = 1.9974 [kg/s]
\]
Example 5.17
A syringe apparatus is being use to withdrawn blood . If the piston is withdrawn at 0.01 [\(m/s\)]. At that stage air leaks in around the piston at the rate 0.000001 [\(m^3/s\)]. What is the average velocity of blood into syringe (at the tip)? The syringe radios is 0.005[m] and the tip radius is 0.0003 [m].
Solution 5.17
The situation is unsteady state (in the instinctive c.v. and coordinates) since the mass in the control volume (the syringe volume is not constant). The chose of the control volume and coordinate system determine the amount of work. This part of the solution is art. There are several possible control volumes that can be used to solve the problem. The two "instinctive control volumes'' are the blood with the air and the the whole volume between the tip and syringe plunger (piston). The first choice seem reasonable since it provides relationship of the total to specific material. In that case, control volume is the volume syringe tip to the edge of the blood. The second part of the control volume is the air. For this case, the equation (??) is applicable and can be written
\[
\label{syringe:blood}
U_{tip}\,A_{tip} \, \cancel{\rho_b} = U_b\,A_{s}\,\cancel{\rho_b}
\]
\[
\label{syringe:airS}
\overbrace{U_{plunger} \,{A_s} \,\rho_a - U_b\,{A_{s}}\,\rho_b}^
{\text{moving b.c.}} = \overbrace{\rho_a \dot{Q}_{in}}^{\text{ in/out}}
\]
In the case, the choice is coordinates moving with the plunger, the relative plunger velocity is zero while the blood edge boundary velocity is \(U_{plunger} - U_b\). The air governing equation is
\[
\label{syringe:airP}
\overbrace{\left(U_{plunger}- U_b\right)}^{\text{blood b. velocity}}
\,{A_{s}}\,\rho_b
= \overbrace{\rho_a \dot{Q}_{in}}^{\text{in/out}}
\]
In the case of coordinates are attached to the blood edge similar equation is obtained. At this stage, there are two unknowns, \(U_b\) and \(U_{tip}\), and two equations. Using equations (??) and (??) results in
\[
\begin{array}{rcl}
\label{syringe:Ub}
U_b = U_{plunger} - \dfrac{\rho_a\,Q_{in}}{A_s\,\rho_b} \
U_{tip} = \dfrac{U_b\,A_s}{A_{tip}} =
\dfrac{\left( U_{plunger} - \dfrac{\rho_a\,Q_{in}}{A_s\,\rho_b}\right) \, A_s}{A_{tip}}
\end{array}
\]
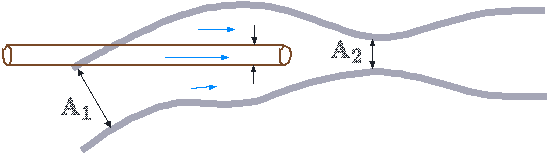
Fig. 5.13 Water jet Pump.
Example 5.18
The apparatus depicted in Figure 5.13 is referred in the literature sometime as the water–jet pump. In this device, the water (or another liquid) is pumped throw the inner pipe at high velocity. The outside pipe is lower pressure which suck the water (other liquid) into device. Later the two stream are mixed. In this question the what is the mixed stream averaged velocity with \(U_1= 4.0[m/s]\) and \(U_2= 0.5[m/s]\). The cross section inside and outside radii ratio is \(r_{1}/r_{2}=0.2\). Calculate the mixing averaged velocity.
Solution 5.18
The situation is steady state and which density of the liquid is irrelevant (because it is the same at the inside and outside).
\[
\label{jetPumpeq:gov1}
U_1\,A_1 + U_2\,A_2 = U_3\, A_3
\]
\[
\label{jetPumpeq:gov}
U_3 = \dfrac{ U_1\,A_1 + U_2\,A_2 } { A_3}
= U_1 \dfrac{A_1}{A_3} + U_2 \left( 1 - \dfrac{ A_1}{A_3\dfrac{}{}} \right)
\]
Contributors and Attributions
Dr. Genick Bar-Meir. Permission is granted to copy, distribute and/or modify this document under the terms of the GNU Free Documentation License, Version 1.2 or later or Potto license.


