4.5: Cutting Rock Hyperbaric

Last updated
Save as PDF

Page ID: 29440

\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \) \( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)\(\newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\) \( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\) \( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\) \( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\) \( \newcommand{\Span}{\mathrm{span}}\) \(\newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\) \( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\) \( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\) \( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\) \( \newcommand{\Span}{\mathrm{span}}\)\(\newcommand{\AA}{\unicode[.8,0]{x212B}}\)

In the case of hyperbaric rock cutting, the pore pressures cannot be neglected anymore. Gravity and inertial forces can still be neglected. Usually rock has no adhesion. When the hydrostatic pressure is larger than or approaching the UCS value of the rock, the rock tends to fail in a semi-ductile manner, named cataclastic failure. It is almost like the hydrostatic pressure can be added to the tensile strength of the rock.

Rock cutting under hyperbaric conditions (deep sea mining) is dominated by the internal shear strength, the pore vacuum pressure forces and by the internal and external friction angles. The main cutting mechanism is the Crushed Type, cataclastic semi-ductile cutting. This is covered in Chapter 9: Rock Cutting: Hyperbaric Conditions.

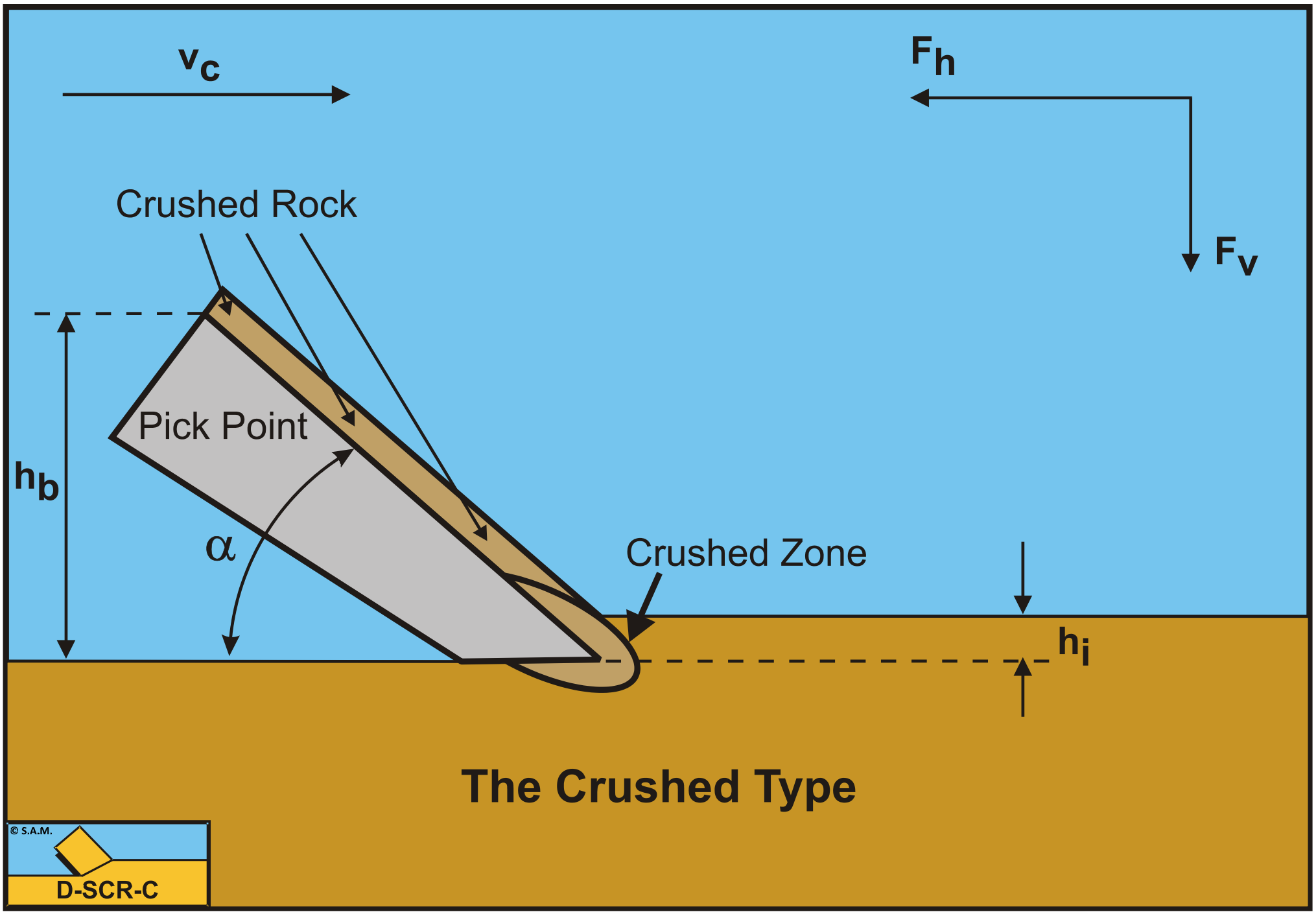
Screen Shot 2020-08-17 at 12.04.50 PM.png — Figure 4-8: The Crushed Type in hyperbaric rock cutting.

The forces K₁ and K₂ on the blade, chisel or pick point are now:

\[\ \begin{array}{left} \mathrm{K}_{1}=& \frac{\mathrm{W}_{2} \cdot \sin (\delta)+\mathrm{W}_{1} \cdot \sin (\alpha+\beta+\delta)+\mathrm{G} \cdot \sin (\alpha+\delta)}{\sin (\alpha+\beta+\delta+\varphi)} \\ &+\frac{-\mathrm{I} \cdot \cos (\alpha+\beta+\delta)-\mathrm{C} \cdot \cos (\alpha+\beta+\delta)+\mathrm{A} \cdot \cos (\delta)}{\sin (\alpha+\beta+\delta+\varphi)} \end{array}\tag{4-25}\]

\[\ \begin{array}{left} \mathrm{K}_{2}=& \frac{\mathrm{W}_{2} \cdot \sin (\alpha+\beta+\varphi)+\mathrm{W}_{1} \cdot \sin (\varphi)+\mathrm{G} \cdot \sin (\beta+\varphi)}{\sin (\alpha+\beta+\delta+\varphi)} \\ &+\frac{+\mathrm{I} \cdot \cos (\varphi)+\mathrm{C} \cdot \cos (\varphi)-\mathrm{A} \cdot \cos (\alpha+\beta+\varphi)}{\sin (\alpha+\beta+\delta+\varphi)} \end{array}\tag{4-26}\]

The normal forces N₁ on the shear plane and N₂ on the blade are:

\[\ \mathrm{N}_{1}=\mathrm{K}_{1} \cdot \cos (\varphi) \quad\text{ and }\quad \mathrm{N}_{2}=\mathrm{K}_{2} \cdot \cos (\delta)\tag{4-27}\]

The horizontal and vertical forces on the blade, chisel or pick point are:

\[\ \mathrm{F}_{\mathrm{h}}=-\mathrm{W}_{2} \cdot \sin (\alpha)+\mathrm{K}_{2} \cdot \sin (\alpha+\delta)+\mathrm{A} \cdot \cos (\alpha)\tag{4-28}\]

\[\ \mathrm{F}_{\mathrm{v}}=-\mathrm{W}_{2} \cdot \cos (\alpha)+\mathrm{K}_{2} \cdot \cos (\alpha+\delta)-\mathrm{A} \cdot \sin (\alpha)\tag{4-29}\]

The equilibrium of moments around the blade tip is:

\[\ \left(\mathrm{N}_{1}-\mathrm{W}_{1}\right) \cdot \mathrm{R}_{1}-\mathrm{G} \cdot \mathrm{R}_{3}=\left(\mathrm{N}_{2}-\mathrm{W}_{2}\right) \cdot \mathrm{R}_{2}\tag{4-30}\]

Analyzing these equations results in the following conclusions:

Since the argument in the cosine of the cohesive term in the force K₁ is always greater than 90 degrees, the cosine is negative and the term as a whole is positive. This results in positive forces on the blade, chisel or pick point and also positive normal forces. The minimum normal stress however equals the normal stress on the shear plane, minus about the radius of the Mohr circle. The result will always be a positive minimum normal stress because of the influence of the large pore under pressure forces.
On the blade the Curling Type may occur based on the equilibrium of moments, because the pore pressure force depends on the length of the blade. When cutting a very thin layer of rock, compared to the height of the blade, as in oil drilling, this will occur. On the shear plane, both the pore pressure force and the cohesive force depend on the length of the shear plane, which may result in brittle tensile failure, the Tear Type, here named the Chip Type. Usually this is suppressed by the very large pore under pressures in relation to the strength of the rock.
When the argument of the sine in the denominator gets close to 180 degrees, the forces become very large. If the argument is greater than 180 degrees, the forces would become negative. Since both conditions will not happen in nature, nature will find another cutting mechanism, the wedge mechanism.