6.1: The hydrogen atom

Hydrogen is the simplest element. There are just two components: an electron and a positively charged nucleus comprised of a single proton.

The electron experiences the attractive potential of the nucleus. The nuclear potential is spherically symmetric and given by the Coulomb potential

\[ V(r) = -\frac{Zq^{2}}{4\pi \epsilon_{0}r} \nonumber \]

where r is the radial separation of the electron and the nucleus \(\epsilon_{0}\) is the dielectric constant and Z is the number of positive charges at the nucleus. For hydrogen there is one proton, and Z = 1.

Recall that a general expression for the kinetic energy operator in three dimensions is:

\[ \hat{T} = - \frac{\hbar^{2}}{2m_{e}}\nabla^{2} \nonumber \]

where \(\nabla^{2}\) is the Laplacian operator.

In rectilinear coordinates (x,y,z)

\[ \nabla^{2} = \frac{d^{2}}{dx^{2}}+\frac{d^{2}}{dy^{2}}+\frac{d^{2}}{dz^{2}} \nonumber \]

In spherical coordinates \((r, \theta, \phi)\)

\[ \nabla^{2} = \frac{1}{r}\frac{d^{2}}{dr^{2}}+\frac{1}{r^{2}}\frac{1}{sin^{2}\theta}\frac{d^{2}}{d\phi^{2}}+\frac{1}{r^{2}}\frac{1}{sin\theta}\frac{d}{d\theta}sin\theta \frac{d}{d\theta} \nonumber \]

Thus, the Hamiltonian for the hydrogen atom is

\[ \hat{H} = -\frac{\hbar^{2}}{2m} \nabla^{2} - \frac{Zq^{2}}{4\pi\epsilon_{0}r} \nonumber \]

\( = -\frac{\hbar^{2}}{2m} \left( \frac{1}{r}\frac{d^{2}}{dr^{2}}r +\frac{1}{r^{2}}\frac{1}{sin^{2}\theta}\frac{d^{2}}{d\phi^{2}}+\frac{1}{r^{2}}\frac{1}{sin \theta}\frac{d}{d\theta}sin\theta \frac{d}{d\theta} \right) - \frac{Zq^{2}}{4\pi\epsilon_{0}r} \)

This takes a bit of algebra to solve for the atomic orbitals and associated energies. An approximate solution (assuming a box potential rather than the correct Coulomb potential) is contained in Appendix 2.

The lowest energy solutions are plotted in Figure 6.2.1, below

Figure \(\PageIndex{1}\): The first five orbitals of the hydrogen atom together with their radial profiles.

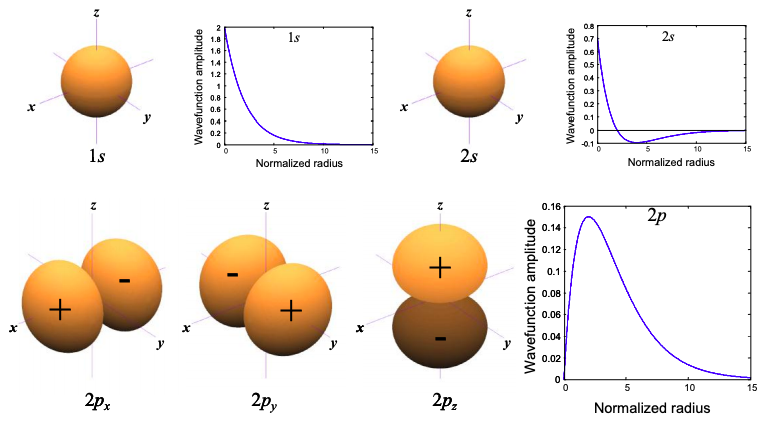
Each of the solutions shown in Figure 6.2.1 is labeled either s or p. These letters describe the angular symmetry of solution. They are the index for the orbital angular momentum of the electron. "s" orbitals exhibit even symmetry about the origin in every dimension. Orbitals that exhibit odd symmetry about the origin in one dimension are labeled "p". We show in Appendix 1 that the eigenfunctions of an electron restricted to the surface of a sphere are characterized by quantized angular momentum. We are only showing the s and p solutions but there are an infinite set of solutions, e.g. s, p, d, f… corresponding to orbital angular momenta of 0, 1, 2, 3…

The energy of each atomic orbital is also labeled by an integer known as the principal quantum number. Thus, the 1s orbital is the lowest energy s orbital, 2p and 2s orbitals are degenerate first excited states.

Knowledge of the exact atomic orbitals is not necessary for our purposes. Rather, we will use the orbitals as symbolic building blocks in the construction of molecular orbitals: electron wavefunctions in molecules.