1.6: Continuous Time Impulse Function

Introduction

In engineering, we often deal with the idea of an action occurring at a point. Whether it be a force at a point in space or some other signal at a point in time, it becomes worth while to develop some way of quantitatively defining this. This leads us to the idea of a unit impulse, probably the second most important function, next to the complex exponential, in this systems and signals course.

Dirac Delta Function

The Dirac delta function, often referred to as the unit impulse or delta function, is the function that defines the idea of a unit impulse in continuous-time. Informally, this function is one that is infinitesimally narrow, infinitely tall, yet integrates to one. Perhaps the simplest way to visualize this is as a rectangular pulse from \(a-\frac{\varepsilon}{2}\) to \(a+\frac{\varepsilon}{2}\) with a height of \(\frac{1}{\varepsilon}\). As we take the limit of this setup as \(\varepsilon\) approaches 0, we see that the width tends to zero and the height tends to infinity as the total area remains constant at one. The impulse function is often written as \(\delta(t)\).

\[\int_{-\infty}^{\infty} \delta(t) \mathrm{d} t=1 \nonumber \]

Figure \(\PageIndex{1}\): This is one way to visualize the Dirac Delta Function.

Figure \(\PageIndex{2}\): Since it is quite difficult to draw something that is infinitely tall, we represent the Dirac with an arrow centered at the point it is applied. If we wish to scale it, we may write the value it is scaled by next to the point of the arrow. This is a unit impulse (no scaling).

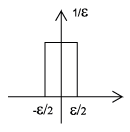
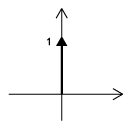
Below is a brief list a few important properties of the unit impulse without going into detail of their proofs.

Unit Impulse Properties

• \(\delta(\alpha t)=\frac{1}{|\alpha|} \delta(t)\)
• \(\delta(t)=\delta(-t)\)
• \(\delta(t)=\frac{d}{dt} u(t)\), where \(u(t)\) is the unit step.
• \(f(t) \delta(t)=f(0) \delta(t)\)

The last of these is especially important as it gives rise to the sifting property of the dirac delta function, which selects the value of a function at a specific time and is especially important in studying the relationship of an operation called convolution to time domain analysis of linear time invariant systems. The sifting property is shown and derived below.

\[\int_{-\infty}^{\infty} f(t) \delta(t) d t=\int_{-\infty}^{\infty} f(0) \delta(t) d t=f(0) \int_{-\infty}^{\infty} \delta(t) d t=f(0) \nonumber \]

Unit Impulse Limiting Demonstration

Figure \(\PageIndex{3}\): Click on the above thumbnail image (when online) to download an interactive Mathematica Player demonstrating the Continuous Time Impulse Function.

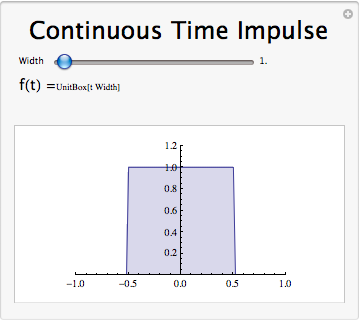
Continuous Time Unit Impulse Summary

The continuous time unit impulse function, also known as the Dirac delta function, is of great importance to the study of signals and systems. Informally, it is a function with infinite height ant infinitesimal width that integrates to one, which can be viewed as the limiting behavior of a unit area rectangle as it narrows while preserving area. It has several important properties that will appear again when studying systems.