3.4: Mixture Composition
- Page ID
- 81486
Frequently it is necessary to describe the composition of a system that contains a mixture. The composition of a mixture can be described on either a mass or a molar basis. Before proceeding we should review the relationship between the fundamental dimensions of mass and amount of substance (moles).
Molar Mass and the Amount of Substance
The quantity of material inside the system is usually specified in one of two ways: (1) the mass or (2) the amount of substance (the number of molecules or atoms) inside the system. The amount of substance unlike the mass of a substance specifies a unique number of particles. These particles can be atoms, molecules, electrons, etc.; thus, it is necessary to indicate exactly what particles are being counted. By definition, a mole is an amount of substance that contains the same number of particles as there are atoms in \(0.012\) kilograms of carbon-12 (\(6.022 \times 10^{23}\) particles). The mass of one mole of substance is called its molar (or molecular) mass, \(M\). If we use the symbol \(n\) for the number of moles, then we have the following relationship between the mass, moles, and molecular mass of a substance: \[ m = nM \nonumber \]
In physics and chemistry, most calculations involving the amount of substance always made use of the gram-mole (\(\mathrm{mol}\)) or just mole for short. In engineering calculations we will have need of many different moles. To understand how these are related, consider the molar mass of diatomic oxygen \(\mathrm{O}_{2}\):
\[M_{\mathrm{O}_{2}} = \underbrace{32.0 \ \frac{\mathrm{g}}{\text {mol }}}_{\begin{array}{l} \text {Form commonly} \\ \text {used in physics} \\ \text {and chemistry} \end{array}} = \underbrace{32.0 \ \frac{\mathrm{kg}}{\mathrm{kmol}}=0.0320 \ \frac{\mathrm{kg}}{\mathrm{mol}}}_{\begin{array}{c} \text {Other forms commonly} \\ \text {in the Si system} \end{array}} = \underbrace{32.0 \ \frac{\mathrm{lbm}}{\mathrm{lbmol}} = 32.0 \ \frac{\mathrm{slug}}{\mathrm{slugmol}}}_{\text {Forms used in the AES system}} \nonumber \]
In addition to the gram-mole (\(\mathrm{mol}\)), the molar mass can be described in terms of the kilogram-mole (\(\mathrm{kmol}\)), the pound-mole (\(mathrm{lbmol}\)), and the slug-mole (\(\mathrm{slugmol}\)). We will predominately use the kilogram-mole and the pound-mole for our calculations; however, anytime we use the word "mole" or refer to a "molar basis", the actual mole unit could be any of the units mentioned above.
The key to using these different quantities is to recognize that each one of them is a way of specifying a definite number of molecules or atoms. Consider the example below to test your understanding of this concept.
How many moles of diatomic oxygen \( \left( \mathrm{O}_2 \right)\) have the same number of particles as one pound-mole of \(\mathrm{O}_2\)?
Solution
Starting with the molar mass of diatomic oxygen, we have \(32.00 \ \mathrm{lbm} / \mathrm{lbmol}=32.00 \ \mathrm{~g} / \mathrm{mol}\). If we divide both sides through by \(32.00\) we discover that \(1 \ \mathrm{lbm} / \mathrm{lbmol}=1 \ \mathrm{~g} / \mathrm{mol}\). Starting with this result
\[ \begin{align*} 1 \ \frac{\mathrm{lbm}}{\mathrm{lbmol}}=1 \ \frac{\mathrm{g}}{\mathrm{mol}} \quad \rightarrow \quad 1 \mathrm{lbmol} &=\left(\frac{\mathrm{lbm}}{\mathrm{g} / \mathrm{mol}}\right)=\left(\frac{\mathrm{lbm}}{\mathrm{g}}\right) \times(\mathrm{mol}) \times \underbrace{ \left( 0.4536 \ \frac{\mathrm{kg}}{\mathrm{lbm}} \right) }_{\mathrm{=} 1} \times \underbrace{\left(1000 \ \frac{\mathrm{g}}{\mathrm{kg}}\right)}_{\mathrm{\equiv} 1} \\ &=453.6 \ \mathrm{mol} \end{align*} \nonumber \]
Similarly,
\[ \begin{align*} 1 \ \mathrm{kmol}&=1000 \ \mathrm{~mol} \\ 1 \ \mathrm{slugmol} &=32.174 \ \mathrm{lbmol}=14,594 \ \mathrm{~mol}=14.594 \ \mathrm{kmol} \end{align*} \nonumber \]
What is going on here? Would these results hold for other substances? How are the number of particles in each "mole" related?
A tank contains \(50 \ \mathrm{kg}\) of methane gas. How many moles of methane \(\left(\mathrm{CH}_{4}\right)\) are in the tank, in \(\mathrm{kmol}\)?
Starting with the basic equation relating moles, mass and molar mass, we have \(m=n M\). The molar mass for methane is \(M_{\text {methane}}=16.04\). Rearranging the basic relation we have \[n = \frac{m}{M} = \frac{50 \ \mathrm{kg}}{16.04 \ \mathrm{kg} / \mathrm{kmol}}=3.12 \ \mathrm{kmol} \nonumber \]
How many pound-moles would you have? \[n = \frac{m}{M} = \frac{50 \ \mathrm{kg}}{16.04 \ \mathrm{lbm} / \mathrm{lbmol}} = (3.12 \ \mathrm{lbmol}) \times \left( \frac{\mathrm{kg}}{\mathrm{lbm}}\right) \times \underbrace{\left(\frac{\mathrm{lbm}}{0.4536 \mathrm{kg}}\right)}_{=1} = 6.88 \ \mathrm{lbmol} \nonumber \]
How many ton-mol if 1 ton \(=2000 \ \mathrm{lbm}\) ?
Now that we have reviewed the difference between mass and amount of substance, we can turn to mixture composition.
Composition on a Mass Basis
When mixture composition is specified on a mass basis, we are interested in knowing the composition in terms of the mass of the various components. Because of the direct relationship between the mass of mixture and its weight, you will sometimes see "analysis by weight" or "gravimetric analysis" used instead of mass basis.
To see how the mass basis is specified, consider a three component mixture formed from compounds \(A\), \(B\), and \(C\). The mass of the mixture is just the sum of the mass of each component: \[m_{mix} = m_{A} + m_{B} + m_{C} = \sum_{i=1}^{N} m_{i} \nonumber \]
If we now divide by the mass of mixture, we obtain \[ 1=\frac{m_{A}}{m_{mix}} + \frac{m_{B}}{m_{mix}} + \frac{m_{C}}{m_{mix}} = \sum_{i=1}^{N} \frac{m_{i}}{m_{mix}} \nonumber \]
The ratio of \(m_{i}\), the mass of the \(i\)-th component, to \(m_{mix}\), the mass of the mixture, is called the mass fraction \(m \mathcal{f}_{i}\) of the \(i\)-th component of the mixture: \[m \mathcal{f}_{i} \equiv \frac{m_{i}}{m_{mix}} \nonumber \]
Combining Eq. \(\PageIndex{3}\) and Eq. \(\PageIndex{4}\), we see that the mass fractions of a mixture sum to unity: \[\sum_{i=1}^{N} m \mathcal{f}_{i}=1 \nonumber \]
When the composition of mixture is specified on a mass basis, the mass fractions are specified for the components of the mixture.
Composition on a Molar Basis
When a molar basis is used, we are interested in knowing the composition in terms of the number of moles of the various components. Again consider our three-component mixture. The number of moles of the mixture equals the sum of the number of moles of each component: \[n_{mix}=n_A + n_B +n_C = \sum_{i=1}^{N} n_{i} \nonumber \]
If we now divide this result by the number of moles in the mixture, we obtain \[1 = \frac{n_{A}}{n_{mix}} + \frac{n_{B}}{n_{mix}} + \frac{n_{C}}{n_{mix}}=\sum_{i=1}^{N} \frac{n_{i}}{n_{mix}} \nonumber \] The ratio of \(n_{i}\), the number of moles of the \(i\)-th component, to \(n_{\text {mix }}\), the number of moles of the mixture, is called the mole fraction \(n \mathcal{f}_{i}\) of the \(i\) th component of the mixture:
\[n \mathcal{f}_{i} \equiv \frac{n_{i}}{n_{mix}} \nonumber \] As with the mass fractions, combining the results of Eq. \(\PageIndex{7}\) and Eq. \(\PageIndex{8}\) reveals that the mole fractions for all components of mixture sum to unity: \[\sum_{i=1}^{N} n \mathcal{f}_{i}=1 \nonumber \] When the composition of a mixture is specified on a molar basis, the mole fractions of each component are specified.
Mixture Molar Mass
In addition to knowing the composition of a mixture, it is often of interest to know the molar mass for a mixture. By definition the mixture molar mass (or apparent molar mass of a mixture) is the ratio of the mixture mass to the number of moles in the mixture: \[M_{mix} = \frac{m_{mix}}{n_{mix}} \nonumber \] The units for this quantity are the same as that for a pure quantity — \(\mathrm{g} / \mathrm{mol}\), \(\mathrm{kg} / \mathrm{kmol}\), or \(\mathrm{lbm} / \mathrm{lbmol}\).
Converting Mixture Composition Basis
Either basis can be used to specify the composition of a mixture. Experience reveals that gaseous mixtures are most commonly described on a molar basis and mixtures containing liquids and solids are described using a mass basis.
It often required to convert the mixture composition from a mole basis to an mass basis or vice versa. This is best done by taking the mixture (either the actual amount or a representative amount), setting up a table of data, and methodically working through to find the necessary information. The following examples demonstrate this technique.
A tank contains \(1500 \ \mathrm{kmol}\) of a gas. The gas is a mixture of oxygen \(\left(\mathrm{O}_{2}\right)\), nitrogen \(\left(\mathrm{N}_{2}\right)\), and carbon dioxide \(\left(\mathrm{CO}_{2}\right)\). The measured mole fractions for two of the components are as follows: \(\mathrm{O}_{2}-20 \%\) and \(\mathrm{N}_{2}-70 \%\).
Determine (a) the number of kilograms in the tank, (b) the mixture composition in mass fractions (weight percent) and (c) the mixture (apparent) molar mass.
Solution
Known: Tank contains a gas mixture.
Find: (a) mass of the gas, \(m_{\text {mix}}\) in \(\mathrm{kg}\); (b) mass fractions, \(m f_{i}\)'s; (c) mixture molar mass, \(M_{\text {mix}}\)
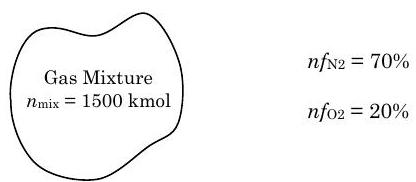
Figure \(\PageIndex{1}\): Given information about a gas mixture.
Strategy \(\rightarrow\) Try using a table format to simplify the calculations.
As a first step, we can complete the table for the mole fractions since the mole fraction of the mixture must sum to equal 100%. Thus \(n f_{\mathrm{CO}_2}=(100-70-20) \%=10 \%\).
Now set up a table including the molar masses of the three constituent gases
| \(n f_{j}\) | \(n_{j}=n f_{j} \cdot n_{\text {mix}}\) | \(M_{j}\) | \(m_{j}=n_{j} \cdot M_{j}\) | \(m f_{j}=m_{j} / m_{\text {mix}}\) | |
|---|---|---|---|---|---|
| \(\mathrm{O}_{2}\) | \(0.20\) | \(300 \ \mathrm{kmol}\) | \(32.00 \mathrm{~kg} / \mathrm{kmol}\) | \(9600 \mathrm{~kg}\) | \(0.210\) |
| \(\mathrm{N}_{2}\) | \(0.70\) | \(1050 \ \mathrm{kmol}\) | \(28.01 \mathrm{~kg} / \mathrm{kmol}\) | \(29410 \mathrm{~kg}\) | \(0.645\) |
| \(\mathrm{CO}_{2}\) | \(0.10\) | \(150 \ \mathrm{kmol}\) | \(44.01 \mathrm{~kg} / \mathrm{kmol}\) | \(6602 \mathrm{~kg}\) | \(0.145\) |
| \(1.00\) | \(1500 \ \mathrm{kmol} = n_{\text{mix}}\) | \(\ldots \ldots \ldots \ldots \ldots\) | \(45612 \mathrm{~kg} = m_{\text{mix}}\) | \(1.000\) |
The number of kilograms of gas in the tank is found in the fifth column of the table. The mass fractions of the mixture are found in the sixth column of the table.
To find the mixture molar mass, we use the definition of mixture molar mass \[m_{\text {mix}}=M_{\text {mix}} n_{\text {mix}} \quad \rightarrow \quad M_{\text {mix}}=\frac{m_{\text {mix}}}{n_{\text {mix}}}=\frac{45612 \mathrm{~kg}}{1500 \mathrm{kmol}}=30.41 \ \frac{\mathrm{kg}}{\mathrm{kmol}} \nonumber \]
Comments
(a) An alternate way to find the mixture molar mass \(M_{\text{mix}}\) uses the component mole fractions and molar masses directly: \[M_{\text {mix}}=\frac{m_{\text {mix}}}{n_{\text {mix}}} = \frac{\displaystyle\sum_{j=1}^{N} m_{j}}{n_{\text {mix}}} = \frac{\displaystyle\sum_{j=1}^{N}\left(n_{j} M_{j}\right)}{n_{\text {mix}}} = \sum_{j=1}^{N}\left(\frac{n_{j}}{n_{\text {mix}}} M_{j}\right) = \sum_{j=1}^{N}\left(n f_{j} M_{j}\right)=M_{\text {mix}} \nonumber \]
(b) Many times you are only given the compositions without any information about the actual amount of substance. Under these conditions, you can still use the table format. Simply assume an amount of substance, say \(100 \ \mathrm{kmol}\), and then work the problem for this size mixture.
(c) If you were given the composition in terms of mass fractions (or weight percents), you would follow the same process as shown in the table, only starting with mass fractions and the known or assumed mass of substance.
A stream of liquid enters a tank with a mass flow rate of \(400 \mathrm{~kg} / \mathrm{min}\). The weight percents (mass fractions) of the two-components in the stream are as follows: water \(\left(\mathrm{H}_{2} \mathrm{O}\right)-90 \%\) and ammonia \(\left(\mathrm{NH}_{3}\right)-10 \%\).
Determine the molar composition of the liquid (mole fractions) and determine the molar flow rate of the liquid in \(\mathrm{kmol} / \mathrm{min}\).
Solution
Known: Mass fractions for a flow stream.
Find: Mole fractions and molar flow rate.
Given: \(\quad \dot{m}=400 \mathrm{~kg} / \mathrm{min} ; \quad m f_{\mathrm{H}_{2} 0}=0.90 ; \quad m f_{\mathrm{NH}_{3}}=0.10\)
Analysis:
Strategy \(\rightarrow\) Try the table to convert from mass fractions to mole fractions.
| \(m f_{j}\) | \(\dot{m}_j = m f_j \cdot \dot{m}_{\text {mix}}\) | \(M_{\text {mix}}\) | \(\dot{n}_j = \dot{m}_j / M_{\text {mix}}\) | \(n f_j = \dot{n}_j / \dot{n}_{\text {mix}}\) | |
|---|---|---|---|---|---|
| \(\mathrm{H}_{2} \mathrm{O}\) | \(0.90\) | \(360.0 \mathrm{~kg} / \mathrm{min}\) | \(18.02 \mathrm{~kg} / \mathrm{kmol}\) | \(19.98 \ \mathrm{kmol} / \mathrm{min}\) | \(0.895\) |
| \(\mathrm{NH}_{3}\) | \(0.10\) | \(40.0 \mathrm{~kg} / \mathrm{min}\) | \(17.03 \mathrm{~kg} / \mathrm{kmol}\) | \(2.35 \ \mathrm{kmol} / \mathrm{min}\) | \(0.105\) |
| \(1.00\) |
\(400.0 \mathrm{~kg} / \mathrm{min}\) |
\(\ldots \ldots \) |
\(22.33 \ \mathrm{kmol} / \mathrm{min}\) |
\(1.000\) |


