Skip to main content
Engineering LibreTexts

6.4: Problems

  1. Last updated
  2. Save as PDF
  • Page ID
    81502

    • \( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \) \( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)\(\newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\) \( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\) \( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\) \( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\) \( \newcommand{\Span}{\mathrm{span}}\) \(\newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\) \( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\) \( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\) \( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\) \( \newcommand{\Span}{\mathrm{span}}\)\(\newcommand{\AA}{\unicode[.8,0]{x212B}}\)

    Problem \(6.1\)

    The bracket \(BCD\) is hinged at \(C\) and attached to a control cable at \(B\). For the loading shown, determine (a) the tension in the cable, (b) the reaction at \(C\). [Remember to explicitly select a system, apply the basic equations, and state your simplifying assumptions.]

    A bracket hinged-connected to a support at point C consists of a vertical arm ending at point B, 0.18 meters above C, and a horizontal arm ending at point D, 0.8 meters to the right of C. A cable connects point B to support A, which is 0.24 meters to the left of A. Two downwards loads of 240 Newtons are applied to the bracket: one halfway along CD and one at point D.

    Figure \(\PageIndex{1}\): A bracket is loaded with two point forces.

    Problem \(6.2\)

    (Adapted from Beer & Johnston, Dynamics, 6th ed., McGraw-Hill)

    Grain falls from a hopper onto a chute \(C B\) at the rate of \(240 \ \mathrm{lbm} / \mathrm{s}\). It hits the chute at \(A\) with a velocity of \(20 \ \mathrm{ft} / \mathrm{s}\) and leaves at \(B\) with a velocity of \(15 \ \mathrm{ft} / \mathrm{s}\), forming an angle of \(10^{\circ}\) with the horizontal. Knowing that the combined weight of the chute and of the grain it supports is \(600 \ \mathrm{lbf}\) and acts at \(G\), determine the reaction of the roller support \(B\) and the components of the reaction at the hinge \(C\).

    Side view of a curved chute CGB shows point C at the upper left, connected to a wall by a hinge support; point G somewhat below and 7 feet to the right of point C; and point B, connected to the floor with a roller, 6 feet below and 12 feet to the right of point C. Grain falls onto the chute at point A, 3 feet to the right of point C, and exits the chute at point B in a stream 10 degrees below the horizontal.

    Figure \(\PageIndex{2}\): Side view of grain falling onto a curved chute and exiting in an angled stream.

    [Be sure to sketch your system carefully so that you can see what is happening. Do not assume that you know any dimensions other than the ones given to you in the problem statement and figure. Also note that you can calculate the angular momentum about any point inside or on the boundary of the system. It is usually best to pick the point that minimizes the calculations or where the transports of angular momentum are easiest to see.]

    Problem \(6.3\)

    (Adapted from Pestel & Thomson, Statics, McGraw-Hill)

    For the clamping device shown in the figure, determine the forces \(\mathbf{F}_{1}\) and \(\mathbf{F}_{2}\).

    A horizontal bar 225 mm long has its left end resting on a 30-degree incline that slants down and to the right. A chain is attached to the bar 25 mm from the left endpoint and is pulled down and to the right with force F1, parallel to the incline. A second chain is attached to the bar 75 mm from the left endpoint and is pulled up and to the left with force F2, parallel to the incline. A load of 20 lbf hangs from the right endpoint of the bar.

    Figure \(\PageIndex{3}\): A clamping device experiences two unknown tension forces.

    Problem \(6.4\)

    (Adapted from Pestel & Thomson, Statics, McGraw-Hill)

    A monorail car with the dimensions shown in the figure is driven by only the front wheel. If the coefficient of static friction between the wheel and the rail is \(0.60\), determine the maximum acceleration possible for the car.

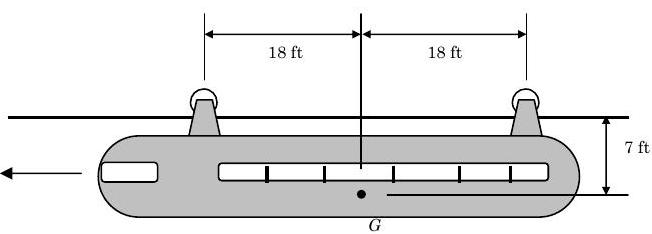    The body of a monorail car hangs below its track, while its wheels rest on top of the track. The car's center of gravity G is 7 feet below the track and 18 feet from each wheel. The car moves towards the left.

    Figure \(\PageIndex{4}\): A monorail car hangs below a track, supported by its wheels.

    Answer

    \(0.340 \mathrm{~g}\)

    Problem \(6.5\)

    The loaded trailer shown in the figure has a mass of \(900 \mathrm{~kg}\) with a center of mass at \(G\) and is attached at \(A\) to a rear-bumper hitch. Pertinent dimensions are given on the diagram.

    (a) Determine the vertical component of the hitch force acting on the trailer at \(A\) when the trailer is stationary. Give both the magnitude, in newtons, and the direction of the force.

    (b) If the car accelerates to the right at the rate of \(4.5 \mathrm{~m} / \mathrm{s}^{2}\), determine the vertical component of the hitch force acting on the trailer at \(A\). Neglect the small friction force exerted on the relatively light wheels. Give both the magnitude, in newtons, and the direction of the force.

    Side view of a single-axle trailer that has center of mass G at a height of 0.9 meters above the ground. The trailer wheel is directly below G and contacts the ground at point B. B is 1.2 meters to the left of point A, the auto hitch, which is located 0.5 meters above the ground.

    Figure \(\PageIndex{5}\): Side view of a loaded trailer.

    Problem \(6.6\)

    You have been challenged by your buddies to balance a broomstick in your hand. So that you don't embarrass yourself, you've decided to do some analysis before you put on a show.

    As a first approximation, you assume that the broomstick can only move in the plane of the paper. The broom has a mass \(m = 2.0 \mathrm{~lbm}\) and an overall length \(L = 5 \mathrm{~ft}\). The center of mass of the broom, including the bristles, is located a distance \(0.6 L\) from the end of the broom handle

    If you start to balance the broom when it leans an angle \(\theta=30^{\circ}\) from the vertical, your challenge is to move your hand horizontally so that the broom maintains this orientation, i.e. it undergoes linear translation. Since you cannot grab the broom, assume that your hand can only resist forces in the \(x\) - and \(y\)-directions.

    For these conditions, determine (a) the magnitude and direction of the reaction of your hand on the broom, and (b) the direction and magnitude of the horizontal acceleration of the broom.

    The end of a broomstick rests on a person's flat hand, which can move only to the right or the left. The broom is tilted at an angle theta from the vertical and has center of mass G along the stick.

    Figure \(\PageIndex{6}\): A broom is balanced on a hand via the end of its broomstick.

    Problem \(6.7\)

    A Pelton wheel turbine is used extract energy from a stream of flowing water. When it is operating at steady-state conditions, the water stream enters the rotating turbine wheel as shown with velocity \(V_{1}\) and leaves the turbine with velocity \(V_{2}\) at an angle \(\theta\). Under these conditions, the turbine wheel rotates about the axis through point \(O\). The black dot at \(O\) represents the turbine shaft. For steady-state operation, two reaction forces \(R_{\mathrm{x}}\) and \(R_{\mathrm{y}}\) and a torque (or moment) \(M_O\) must be applied to the shaft at point \(O\).

    If the mass flow rate is \(50 \mathrm{~kg} / \mathrm{s}\) and \(V_{1}=V_{2}=30 \mathrm{~m} / \mathrm{s}\), determine the two reaction forces and torque at \(O\) if \(\theta=\) (a) \(0^{\circ}\), (b) \(30^{\circ}\), (c) \(60^{\circ}\), (d) \(90^{\circ}\), (d) \(120^{\circ}\), (e) \(150^{\circ}\), and (f) \(180^{\circ}\).

    A wheel of diameter 1.0 m, with its center at point O, rotates clockwise. A stream of water moving to the right at velocity V1 impacts a section of the wheel at the upper left, runs along the wheel, and runs off at the upper right, moving at velocity V2 at an angle theta above the horizontal.

    Figure \(\PageIndex{8}\): Water runs along a section of a wheel turbine, turning it clockwise.

    Problem \(6.8\)

    (Adapted from Bedford & Wallace, Dynamics, 2nd ed., Addison-Wesley)

    The slender bar weighs \(10 \ \mathrm{lbf}\) and the disk weighs \(20 \ \mathrm{lbf}\). The coefficient of kinetic friction between the disk and the horizontal surface is \(0.1\). If the disk has an initial counterclockwise angular velocity of \(10 \ \mathrm{rad} / \mathrm{s}\), how long does it take for the disk to stop spinning.

    A 3-foot-long bar slants down and to the right at 30 degrees from the horizontal. Its left end is attached to a wall with a hinge support, and its right end is pinned to the center of a disk of diameter 1 foot that rests on the ground.

    Figure \(\PageIndex{9}\): An angled bar has one end pinned to a wall and the other pinned to a disk resting on the ground.

    Problem \(6.9\)

    Water flows out of a fire hydrant with a velocity of \(50 \ \mathrm{ft} / \mathrm{s}\) and a volumetric flow rate of \(1000 \ \mathrm{gpm}\). The water pressure at the inlet to the hydrant is \(200 \ \mathrm{psia}\) and the atmospheric pressure is \(14.7 \ \mathrm{psia}\). At the base of the hydrant the bolts must resist a normal force holding the hydrant down, a shear force parallel to the ground, and a couple trying to rotate the hydrant off its base. Calculate these reactions assuming steady-state conditions. Assume the density of water is \(62.4 \ \mathrm{lbm} / \mathrm{ft} ^{3}\).

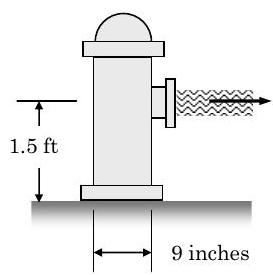
    Side view of a fire hydrant, 9 inches wide. Water exits the nozzle on the right side of the hydrant, 1.5 feet above the ground, moving horizontally.

    Figure \(\PageIndex{9}\): Side view of a fire hydrant with water exiting its nozzle in a horizontal stream.

    Problem \(6.10\)

    The frame shown supports part of a small building. Knowing that the tension in the cable is \(150 \ \mathrm{kN}\), determine the reaction at the fixed end \(E\) (forces and moment).

    6-meter-tall vertical beam DCE is built into the ground at point E. The right endpoint of 7.2-meter horizontal beam ABC is connected to the vertical beam at point C, 3.75 meters above the ground. Four downwards forces of magnitude 20 kN each are applied to the horizontal beam, equally spaced from each other. Point B, 1.8 meters to the right of beam ABC's left endpoint, is connected to point D, at the upper end of DCE, by a cable. Point D is also connected by a cable to point F, on the ground and 4.5 meters to the right of point A.

    Figure \(\PageIndex{10}\): A frame consisting of two beams and two support cables is loaded with several point loads.

    Problem \(6.11\)

    A high-speed jet of air issues from the nozzle \(A\), which has a diameter of \(40 \mathrm{~mm}\), with a velocity of \(240 \mathrm{~m} / \mathrm{s}\) and mass flow rate of \(0.36 \mathrm{~kg} / \mathrm{s}\) and impinges on the vane \(OB\), shown on its edge view. The vane and its right angle extension have negligible mass compared to the attached \(6 \mathrm{-kg}\) cylinder, and are freely pivoted about a horizontal axis through \(O\). The air density under the prevailing condition is \(1.206 \mathrm{~kg} / \mathrm{m}^3\).

    Determine:

    (a) the steady state angle \(\theta\) assumed by the vane with the horizontal, and

    (b) the reaction forces at \(O\).

    Nozzle A points to the left and issues a jet of air that travels horizontally until it strikes vane OB, slanted up and to the right at an angle theta below the horizontal, and travels downwards along the slant at an unchanged speed of 240 m/s. Point O is 120 mm above A, and is pinned to a wall. A 240-mm extension of the vane slants upwards and to the left from point O, and is attached to a 6-kg point mass at its upper end.

    Figure \(\PageIndex{11}\): A jet of air travels horizontally until it impacts a slanted surface, moving at constant speed throughout.

    Problem \(6.12\)

    A ball with mass \(m=5 \ \mathrm{lbm}\) is mounted on a horizontal rod that is free to rotate about a vertical shaft as shown in the figure. In the position shown (position \(A\) ), the rod rotates and the ball is held by a cord attached to the shaft. In this state, the speed of the ball is \(V_1=24 \ \mathrm{in} / \mathrm{s}\). The cord is suddenly cut and the ball moves to the position \(B\) as the rod continues to rotate. Neglecting the mass of the rod, determine the speed of the ball after it has reached the stop \(B\). Be careful to show all of your work.

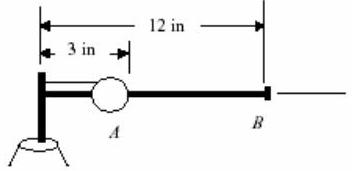
    A vertical shaft attached to a support at its bottom end supports a horizontal rod, which is able to rotate about it. In position A, the horizontal rod is straight to the right of the vertical shaft and is attached to a ball that is 3 inches away from the shaft. In position B, the ball is still straight to the right of the vertical shaft but 12 inches away from the shaft.

    Figure \(\PageIndex{12}\): Two instantaneous positions of a ball in relation to a rotating rod on a shaft.

    Problem \(6.13\)

    (From Dynamics by Beer and Johnson)

    Coal is being discharged from a horizontal conveyor belt at the rate of \(120 \mathrm{~kg} / \mathrm{s}\). It is received at \(A\) by a second belt which discharges it again at \(B\). Knowing that \(v_{1}= 3 \mathrm{~m} / \mathrm{s}\) and \(\mathrm{v}_{2}=4.25 \mathrm{~m} / \mathrm{s}\), and that the second belt assembly and the coal it supports have a total mass of \(472 \mathrm{~kg}\), determine horizontal and vertical components of the reactions at \(C\) and \(D\).

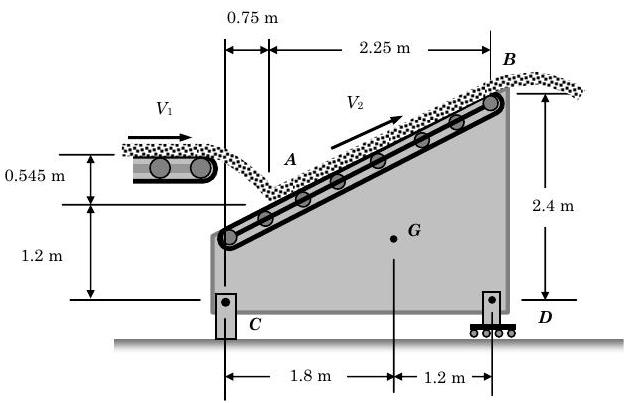    A conveyor belt moves up and to the right. It is supported on a base CD that is 3 meters wide, with C attached to the ground with a pin support and D on a roller. The rightmost end of the conveyor belt, B, is 2.4 meters above the ground. The center of mass G of the belt and support is located 1.8 meters to the right of C. Another horizontal conveyor belt, located to the left of the diagonal belt and 1.745 meters above the ground, moves to the right so that coal falls off its end onto point A on the diagonal belt. Point A is 1.2 meters above the ground and 0.75 meters to the right of C.

    Figure \(\PageIndex{13}\): Coal falls off a horizontal conveyor belt onto a diagonal one where it is again discharged.

    Problem \(6.14\)

    A conveyor system is fitted with vertical panels, and a \(300 \mathrm{~mm}\) rod \(AB\) of mass \(2.5 \mathrm{~kg}\) is lodged between the panel as shown. Assume all the surfaces are smooth. Knowing the acceleration of the panel and the rod is \(1.5 \mathrm{~m} / \mathrm{s}^{2}\) to the left, determine the reactions of the carrier on the rod at \(C\) and \(B\).

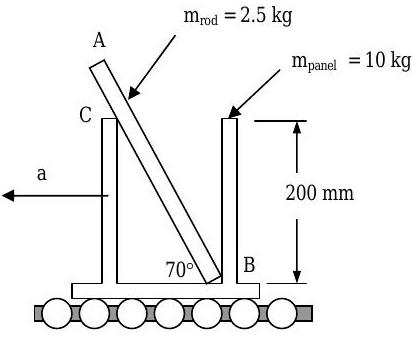
    A horizontal conveyor belt is moving to the left, bearing along two vertical panels of mass 10 kg and height 200 mm each. A long rod AB of mass 2.5 kg makes a 70-degree angle with the horizontal, contacting the panels at point B (the base of the right panel) and point C (the upper edge of the left panel).

    Figure \(\PageIndex{14}\): A rod rests against two vertical panels traveling along a conveyor belt.

    Problem \(6.15\)

    (Modified from Dynamics by Beer and Johnson)

    The forklift shown weighs \(2250 \ \mathrm{lbf}\) and is used to lift a crate of weight \(\mathrm{W}=2500 \ \mathrm{lbf}\). The coefficient of static friction between the crate and the fork lift is \(0.3\).

    Determine:

    (a) the maximum deceleration the forklift can have for the crate not to slip, and

    (b) the maximum deceleration the forklift can have for the forklift not to tip.

    (c) If the truck is moving to the left at a speed of \(10 \ \mathrm{ft} / \mathrm{s}\) when the brakes are applied, determine the smallest distance in which the truck can be brought to a stop if the crate is not to slide and if the truck is not to tip forward.

    Side view of a forklift facing to the left. The forklift wheel on the left side contacts the ground at point A, and the wheel on the right contacts the ground at point B. The forklift's center of gravity G is 3 feet above the ground, 4 feet to the right of A, and 3 feet to the left of B. The forklift is lifting a crate whose center of gravity W is 4 feet above the ground and 3 feet to the left of A.

    Figure \(\PageIndex{15}\): Side view of a two-axle forklift lifting a crate.

    Problem \(6.16\)

    Part (a) Three forces are applied to the L-shaped plate shown in the figure. All forces are applied in the plane of the paper.

    (i) Determine the moment of each force about Point \(O\) and the sum of the moments about Point \(O\), in \(\text{lbf-ft}\).

    (ii) Determine the moment of each force about Point \(P\) and the sum of the moments about Point \(P\), in \(\text{lbf-ft}\).

    (iii) Do any of the forces applied to the plate form a couple? If the answer is yes, which ones?

    [Note: Remember to indicate both the direction (label CW or CCW, or use arrows) and magnitude of all vector quantities.]

    An L-shaped plate consists of one rectangle 10 feet long by 3 feet high, with its top right edge adjoining a second rectangle 4 feet long by 3 feet high. Point O is the lower left corner of the whole plate, and point P is the lower left corner of the top rectangle. A rightwards force F1=200 lbf is applied to the corner directly above P, a leftwards force F2=200 lbf is applied to the corner directly above O, and a downwards force F3=500 lbf is applied to the lower right corner of the whole plate.

    Figure \(\PageIndex{16 \text{a}}\): Point forces are applied at different locations on an L-shaped plate.

    Part (b) Two forces act on the planar, rigid body shown in the figure.

    Determine the individual moment of each force about the point \(O\) and the sum of the moments about point \(O\) in \(\mathrm{N}-\mathrm{m}\). Remember to indicate the direction and magnitude of all vector quantities.

    Figure \(\PageIndex{16 \text{b}}\): Point forces are applied at different locations on an irregularly shaped plate.

    Problem \(6.17\)

    Consider the pulley-mass system shown in the figure. The diameters of the large and small pulley are \(D = 0.5 \mathrm{~m}\) and \(d = 0.25 \mathrm{~m}\), respectively. Both pulleys turn together around the same axle, point \(P\).

    (a) If the pulleys are locked by a brake and cannot turn, the weight of each block produces a moment about point \(P\). Determine the net moment about point \(P\) due to the stationary blocks, in \(\text{N-m}\).

    (b) If the pulleys turn together at the rate of \(2.0\) radians per second in the direction shown, i.e. \(\omega=2.0 \mathrm{rad} / \mathrm{s}\) in the direction shown, each of the blocks has angular momentum and linear momentum. Determine the following for each block:

    • the velocity, in \(\mathrm{m} / \mathrm{s}\),
    • the linear momentum, in \(\mathrm{kg-m} / \mathrm{s}\), and
    • the angular momentum of each mass with respect to axle point \(\mathrm{P}\), in \(\mathrm{kg-m}^{2} / \mathrm{s}\).

    Remember to indicate both the magnitude and direction of all vector quantities.

    A large and a small pulley are secured by a single axle. Block A, of mass 10 kg, hangs from the left side of the smaller pulley. Block B, of mass 5 kg, hangs from the right side of the larger pulley. The pulley system will turn clockwise.

    Figure \(\PageIndex{17}\): Two weights are suspended from two pulleys through which a single axle passes.

    Problem \(6.18\)

    A horizontal force \(\mathbf{P}\) acts on a cabinet that rests on a floor as shown. The cabinet weighs \(120 \ \mathrm{lbf}\). It is known that the coefficient of static friction is \(\mu_{\mathrm{s}}=0.30\) and the coefficient of kinetic friction is \(\mu_{\mathrm{k}}=0.24\).

    (a) If slipping impends, what is the magnitude of \(\mathbf{P}\) ?

    (b) If tipping impends,

    (i) what is the magnitude of \(\mathbf{P}\), and

    (ii) at what point will the resultant floor reaction act?

    (c) What is the smallest magnitude of \(\mathbf{P}\) that will cause the cabinet to move, i.e. either tip or slip?

    A rectangular cabinet 15 inches wide has lower left corner A and lower right corner D. Its center of mass G is 24 inches above the ground, centered horizontally, and a rightwards force P is applied to the cabinet 36 inches above point A.

    Figure \(\PageIndex{18}\): A horizontal force is applied to a tall cabinet resting on the floor.

    Problem \(6.19\)

    Water flows steadily through the elbow-nozzle assembly shown in the figure. The assembly is in the vertical plane (the plane of the paper) and gravity acts as indicated. The assembly is supported entirely by the flange bolts which must resist forces in the \(x\)-and \(y\)-directions as well as a moment. For purposes of analysis, you may assume that all flange reactions are concentrated at the dark "dot" at the centerline of the flange. The available information about the geometry and operating conditions of the assembly are shown in the figure.

    Determine the forces and moment (the reactions) at the flange to support the elbow-nozzle assembly.

    Water flows towards the right through a horizontal pipe connected to an elbow at a flange (interface 1). Interface 1 has a cross-sectional area of 0.450 square meters, water pressure of 400 kPa, and water velocity of 3 m/s. The elbow continues straight for 1 meter before curving down and to the left in a semicircle, then narrowing to a nozzle facing the left (interface 2) whose opening is 0.3 meters to the left of the flange. Interface 2 has a cross-sectional area of 0.050 square meters and water pressure of 100 kPa. The elbow-nozzle assembly filled with water has mass of 4000 kg, with the center of gravity G 1 meter to the right of the flange, 1 meter below the central axis of interface 1, and 1 meter above the central axis of interface 2. Gravity acts straight down. Atmospheric pressure is 100 kPa.

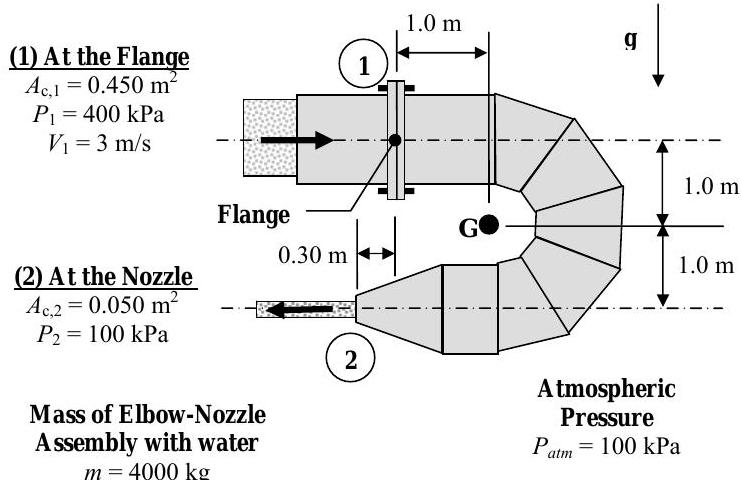
    Figure \(\PageIndex{19}\): Water flows from a horizontal pipe into an elbow-nozzle assembly, connected to the pipe by a flange.

    Problem \(6.20)

    A cyclist is traveling on a level road at a speed \(V=15 \mathrm{~m} / \mathrm{s}\). The combined mass of the person and bicycle shown in the figure is \(m=77 \mathrm{~kg}\). The location of the combined center of mass relative to the wheels is also shown.

    Suddenly the cyclist uses the handlebar brakes to stop the bike. If she only applies the front brakes, determine

    (a) the maximum horizontal force of the ground (the braking force) on the bike at Point \(A\) that can be applied without the bike flipping (neglect any horizontal force exerted by the ground on the rear wheel), and

    (b) the corresponding deceleration of the cyclist and bike measured in \(g\)'s, e.g. \(8.3g\), and

    (c) the maximum allowable value of the kinetic friction coefficient between the front wheel and the ground if the front wheel locks under these conditions and slides on the ground.

    Side view of a cyclist traveling towards the left on a level road. The front wheel of the bike contacts the ground at point A, and the back wheel contacts the ground at point B. The system's center of gravity is located 985 mm above the road, 615 mm to the right of point A, and 445 mm to the left of point B.

    Figure \(\PageIndex{20}\): System consisting of a cyclist and bike, traveling at a constant velocity.

    Problem \(6.21\)

    As part of a school bus safety test program, school buses are being tested for potential roll-over hazards. To test the bus, it is placed on a \(1000\text{-pound}\) moveable concrete pad that rolls freely without friction. The horizontal motion of the pad is produced by a hydraulic ram which pulls the pad to left. A \(5000 \text{-pound}\) school bus is placed on the pad as shown in the figure

    (a) Assuming the bus does not slip on the pad, determine the minimum value of the horizontal acceleration \((d V / d t)\) of the pad in the direction indicated that will cause the bus to tip, in \(\mathrm{ft} / \mathrm{s}^{2}\).

    (b) Determine the force, in \(\mathrm{lbf}\), that the ram must apply to the platform to produce the acceleration found in part (a).

    (c) Determine the minimum static coefficient of friction between the tires and the concrete pad that is required to keep the bus from slipping on the moving pad.

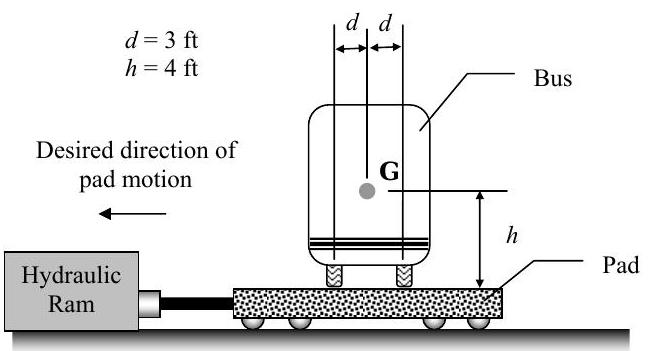
    A concrete pad that can roll freely is attached to a hydraulic ram that pulls the pad to the left. A school bus is placed on the pad, end-on so only two wheels, 6 feet apart, are visible. The bus's center of gravity G is midway between the wheels and 4 feet above the pad.

    Figure \(\PageIndex{21}\): End-on view of a school bus, placed on a rolling pad that is pulled to the left.

    Problem \(6.22\)

    A manufacturer of handheld showerheads uses the setup shown in the figure to test the "handling" characteristics of their showerheads. For the tests, the showerhead is hung from a vertical water-supply pipe using a pinned-joint connection that can only resist horizontal and vertical forces.

    Water enters the showerhead at \(B\) with a purely vertical velocity of \(1.00 \ \mathrm{ft} / \mathrm{s}\) and a volumetric flow rate of \(0.0050 \ \mathrm{ft}^{3} / \mathrm{s}\). The water pressure in the water supply line is \(35 \ \mathrm{psia}\).

    At the spray outlet, the water velocity is \(25 \ \mathrm{ft} / \mathrm{s}\) and the pressure is atmospheric (\(P_{\mathrm{atm}}=14.7 \ \mathrm{psia}\)).

    The test showerhead weighs \(1.3 \ \mathrm{lbf}\). The density of water can be assumed to be \(62.4 \ \mathrm{lbm} / \mathrm{ft}^{3}\)

    For these steady-state test conditions, determine

    (a) the angle \(\theta\) the showerhead makes with the vertical, and

    (b) the horizontal reaction force at the pinned joint.

    Water moves down a vertical pipe until it reaches point B, where a 12-inch-long showerhead is connected to the vertical by a pinned joint. The showerhead has center of gravity G 8 inches from B, and makes an angle theta with the vertical. The showerhead's spray outlet is located at its free end, and sprays water at an angle perpendicular to the showerhead. Gravity acts straight down.

    Figure \(\PageIndex{22}\): Water moves through a system consisting of a shower head pinned to a vertical pipe.

    Problem \(6.23\)

    A steel pipe \(B C\), of length \(L_{p}\) with a mass \(m_{p}\), is attached to the rear bumper of a truck using a lightweight rope \(A B\) of length \(L_{r}\). The coefficient of kinetic friction at point \(C\) is \(\mu_{\mathrm{k}}\). The angles that the rope and the steel pipe make with the horizontal are constant and shown on the figure.

    Determine the constant acceleration of the truck \(a_{\text {truck}}\) and the tension in the rope \(T\) required to maintain these conditions.

    SET UP BUT DO NOT SOLVE. Clearly identify your unknowns and the set of equations you would use to solve for the unknowns.

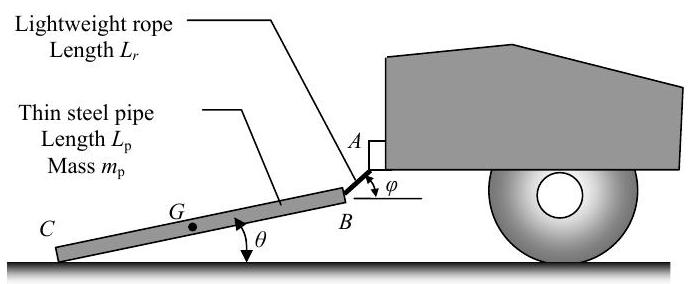
    Side view of a truck facing towards the right. The rear bumper of the truck, point A, is connected by a taut lightweight rope to a long steel pipe BC. Point C rests against the ground. The rope AB makes an angle of Phi with the horizontal, and BC makes an angle of theta with the horizontal.

    Figure \(\PageIndex{23}\): A pipe with one end resting on the ground is connected to a truck bumper by a taut rope.

    Problem \(6.24\)

    The air-handling unit (AHU) shown in the figure is attached to a fixed support that ultimately rests on the roof. The fixed support corresponds with the center of mass \(G\) of the AHU. The AHU weighs \(500 \ \mathrm{lbf}\).

    The expected steady-state operating conditions are shown on the figure. Note that the pressure around the AHU is everywhere atmospheric, \(P_{\text {atm}}=14.7 \ \mathrm{psia}\), except at the air inlet (state 1) where \(P_{1}=14.6 \ \mathrm{psia}\). You may assume that the air density is constant and uniform at \(\rho_{\text {air}}=0.075 \ \mathrm{lbm} / \mathrm{ft}^{3}\).

    Determine the reactions at point \(G\) required to support the AHU.

    Both ends of a fixed horizontal support rest on a flat roof. The center of the support passes through the center of mass G of the AHU. The central portion of the AHU is a vertical column; the air inlet is 1 foot to the right of and 2 feet below point G, and the outlet is 1 foot to the left of and 3 feet above point G. At the inlet, which has an area of 20 square feet, air pressure is 14.6 psia, velocity is 100 ft/s, and volumetric flow rate is 2000 cubic ft/s. At the outlet, which has an area of 10 square feet, air pressure is 14.7 psia, and velocity is 200 ft/s.

    Figure \(\PageIndex{24}\): An air handling unit with one inlet and one outlet is suspended via a fixed support through its center of mass.

    Problem \(6.25\)

    The dragster has a mass of \(1200 \mathrm{~kg}\) and a center of mass at \(G\). A braking parachute is attached at \(C\) and, when released, provides a horizontal braking force of \(F=k_{\mathrm{O}} V^{2}\) where \(k_{\mathrm{O}}=1.6 \mathrm{~N} \cdot \mathrm{s}^{2} / \mathrm{m}^{2}\) and \(V\) is the dragster velocity. If the parachute is deployed when the dragster is traveling too fast, there is a danger that the dragster will flip.

    You may neglect the mass of the wheels and assume the engine is disengaged so that the wheels are freely rolling (so there is no horizontal force between the wheels and the ground) when the parachute is released.

    a) Determine the critical speed (the maximum safe speed) the dragster can have such that the wheels at \(B\) are on the verge of leaving the ground when the parachute is released

    b) If the dragster is traveling at the critical speed when the parachute is deployed, determine the distance it will travel before it stops. Does your answer make sense? If not, what do you think is the problem?

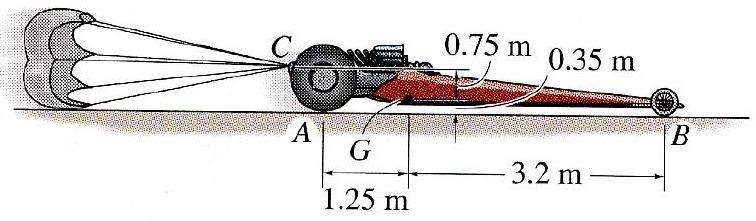
    A dragster is traveling towards the right. Its front wheel contacts the ground at point B and its rear wheel contacts the ground at point A, 4.45 meters to the left of B. It deploys a parachute from point C at its rear, 1.1 meters above the ground. Its center of mass G is located 1.25 meters to the right of A and 0.35 meters above the ground.

    Figure \(\PageIndex{25}\): A dragster traveling to the right deploys a parachute from its rear.

    Problem \(6.26\)

    The girder shown in the figure weighs \(4000 \mathrm{~N}\) and the motor weighs \(1200 \mathrm{~N}\). The motor is hoisting a load that weighs \(8000 \mathrm{~N}\). (Assume mass moment of inertia of the motor \(I_{\text {motor}}\) is negligible.)

    Determine the reactions at \(A\) and \(B\) if the motor is raising the load and the load has an acceleration of \(1.5 \mathrm{~m} / \mathrm{s}\) upward.

    An 8-meter-long girder has its left endpoint A and its right endpoint B supported on rollers. A motor rests on the girder, 6 meters from point B, and turns counterclockwise to raise a load. The horizontal distance from B to the load is 6.15 meters.

    Figure \(\PageIndex{26}\): A motor resting on a girder turns to raise a load.

    Problem \(6.27\)

    A jet of water with density \(\rho\) hits a hinged flap with a mass \(m\) as shown in the figure. The velocity of the water of both the incoming and outgoing jet is \(V_{\text {jet}}\). The incoming water jet is circular with a diameter \(d\). Known dimensions are given in the figure.

    a) Find the angle \(\theta\) that the stationary flap makes with the horizontal. Express your answer in terms of the known quantities.

    b) Find the horizontal and vertical reaction forces at the pin connection \(A\). You may assume that \(\theta\) is known from part (a).

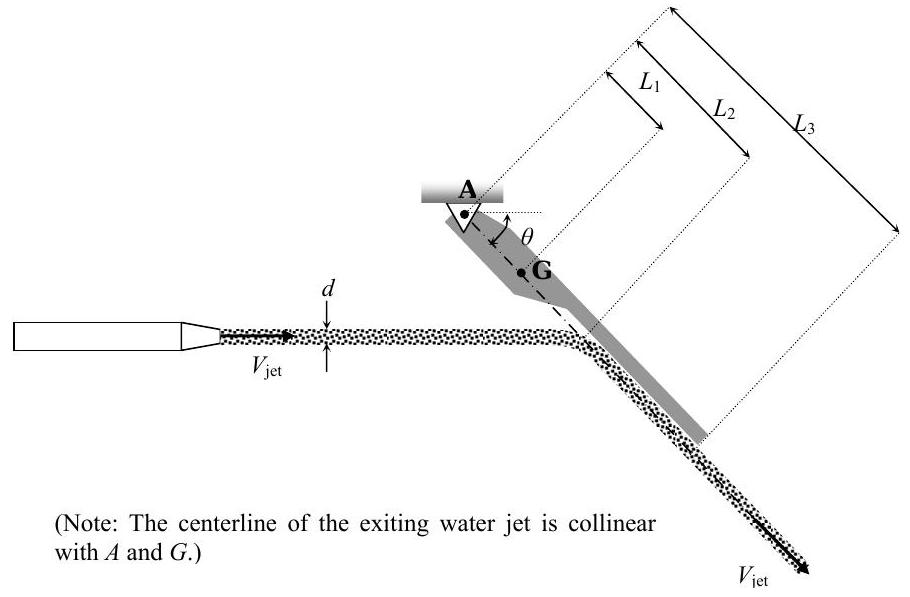
    A flap slants down and to the right at an angle of theta below the horizontal, supported at its upper left endpoint A by a hinge joint. The flap's center of gravity G is a distance of L1 along the flap. A horizontal jet of water at the left of the figure strikes the flap at a point located a distance of L2 from point A, and runs down the flap at constant speed. The flap as a whole has a length of L3. The centerline of the water jet coming off the flap is collinear with AG.

    Figure \(\PageIndex{27}\): Water strikes a hinged, angled flap and runs down it.

    Problem \(6.28\)

    Moo's Dairy has entered the annual Dairy Drag Race at the State Fair. His drag racer is a fully-loaded milk truck shown in the figure. When fully loaded, the milk truck weighs \(5000 \ \mathrm{lbf}\). The truck is a rear-wheel drive vehicle, and the front tires provide negligible frictional drag when rolling.

    The maximum traction between the tires and the road occurs when there is no slip between the tires and the road, i.e. the force between the road and the tires is due to static friction. The static coefficient of friction between the rubber tires and the concrete pavement is \(\mu_{\mathrm{s}}=0.80\).

    (a) Determine the reactions between the tires and the road at points \(A\) and \(B\), in \(\mathrm{lbf}\), when the truck is stationary.

    (b) Determine the maximum acceleration possible for the fully-loaded, rear-wheel drive milk truck, in \(\mathrm{ft} / \mathrm{s}^{2}\) or in \(g\)'s. Also determine the corresponding reactions at points \(A\) and \(B\), in \(\mathrm{lbf}\). Is there any danger of the truck tipping under these conditions?

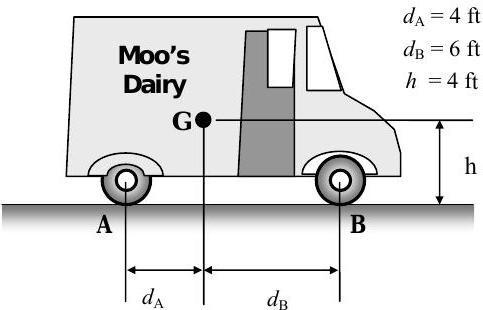
    A milk truck faces towards the right. Its rear wheel contacts the ground at point A on the left, and its front wheel contacts the ground at point B, 10 ft to the right of A. Its center of gravity g is located 4 feet to the right of A and 4 feet above the ground.

    Figure \(\PageIndex{28}\): Side view of a milk truck on level ground.

    This page titled 6.4: Problems is shared under a CC BY-NC-SA 4.0 license and was authored, remixed, and/or curated by Donald E. Richards (Rose-Hulman Scholar) via source content that was edited to the style and standards of the LibreTexts platform; a detailed edit history is available upon request.