1.4: Kinematics of the Elementary Beam Theory

Last updated
Save as PDF

Page ID: 21472

\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \) \( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)\(\newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\) \( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\) \( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\) \( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\) \( \newcommand{\Span}{\mathrm{span}}\) \(\newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\) \( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\) \( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\) \( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\) \( \newcommand{\Span}{\mathrm{span}}\)\(\newcommand{\AA}{\unicode[.8,0]{x212B}}\)

The word “kinematics” is derived from the Greek word “kinema”, which means movements, motion. Any motion of a body involves displacements \(u_i\), their increments \(du_i\) and velocities \(\dot{u}_i\). If the rigid body translations and rotations are excluded, strains develop. We often say “Kinematic assumption” or “Kinematic boundary conditions” or “Kinematic quantities” etc. All it means that statements are made about the displacements and strains and/or their rates. By contrast, the word “static” is reserved for describing stresses and/or forces, even though a body could move. The point is that for statically determined structures, one could determine stresses and forces without invoking motion. Such expressions as “static formulation”, “static boundary conditions”, “static quantities” always refer to stresses and forces.

Elementary is another word in the title of this section that requires explanation. A beam is a slender structure that can be compressed, extended or bent. The beam must be subjected to a transverse load (perpendicular to its axis). Otherwise it becomes something else, as explained in Figure (\(\PageIndex{1}\)).

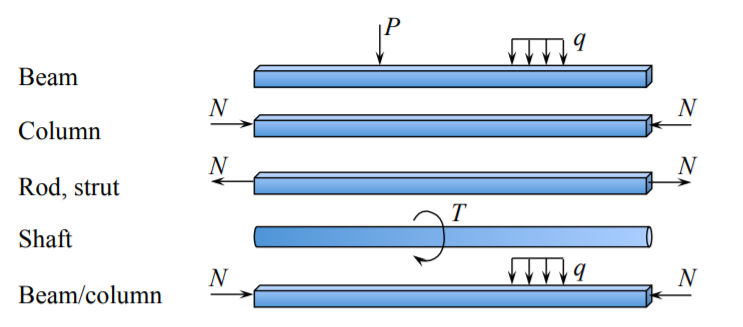
1.4.1.png — Figure \(\PageIndex{1}\): The type of loading distinguishes between five different types of structures.

All the above structures may have a similar slenderness. How slender the structure must be to become a beam. The slenderness is defined as a length to thickness ratio \(\frac{l}{h}\). If \(\frac{l}{h} > 20\), the beam obeys the simplified kinematic assumptions and it is called an “Euler beam”. Much shorter beams with \(\frac{l}{h} < 10\) develop considerable shear stresses in addition to bending stresses and must be treated by a different set of assumptions. Such beams are referred to as Timoshenko beams. The intermediate range \(10 < \frac{l}{h} < 20\) is a grey area where the simplifying assumptions of the elementary beam theory gradually lose validity.

This section deals with a solid section beams, as opposed to thin-walled sections. In the present lecture notes, the rectangular right handed coordinate system \((x, y, z)\) is consistently used. The x-axis is directed along the length of the beam with an origin at a convenient location, usually the end of the center of the beam. The y-axis is in the width direction with its origin on the symmetry plane of the cross-section, Figure (\(\PageIndex{2}\)). Finally, the z-axis is pointing out down and it is measured from the centroidal axis of the cross-section (see Recitation 2 for the definition of a centroidal axis).

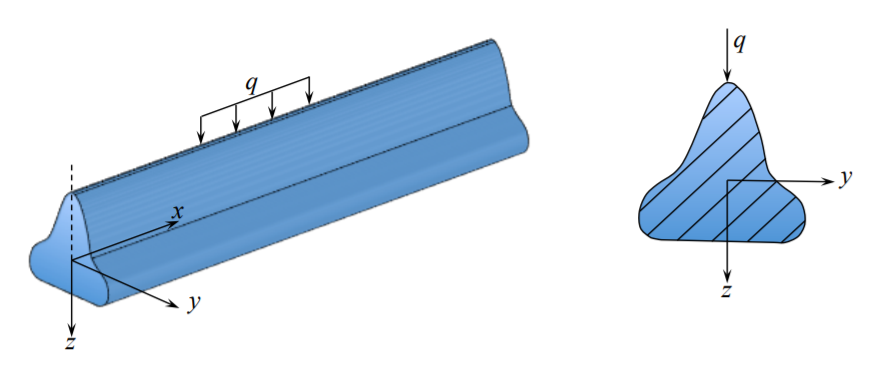
1.4.2.png — Figure \(\PageIndex{2}\): A prismatic slender beam with a symmetric cross-section.

In structural mechanics the components of the displacement vector in \(x\), \(y\), and \(z\) directions are denoted respectively by \((u, v, w)\). The development of elementary beam theory is based on three kinematic assumptions. Additional assumptions on the stress state will be introduced later.