4.2: The Equilibrium of Forces
- Page ID
- 29200
The settling velocity of grains depends on the grain size, shape and specific density. Discrete particles do not change their size, shape or weight during the settling process (and thus do not form aggregates). It also depends on the density and the viscosity of the liquid the grains are settling in, and whether the settling process is laminar or turbulent. A discrete particle in a liquid will settle under the influence of gravity. It will accelerate until the frictional drag force of the liquid equals the value of the gravitational force, after which the vertical (settling) velocity of the particle will be constant (Figure 4.2-1), the so called terminal settling velocity.
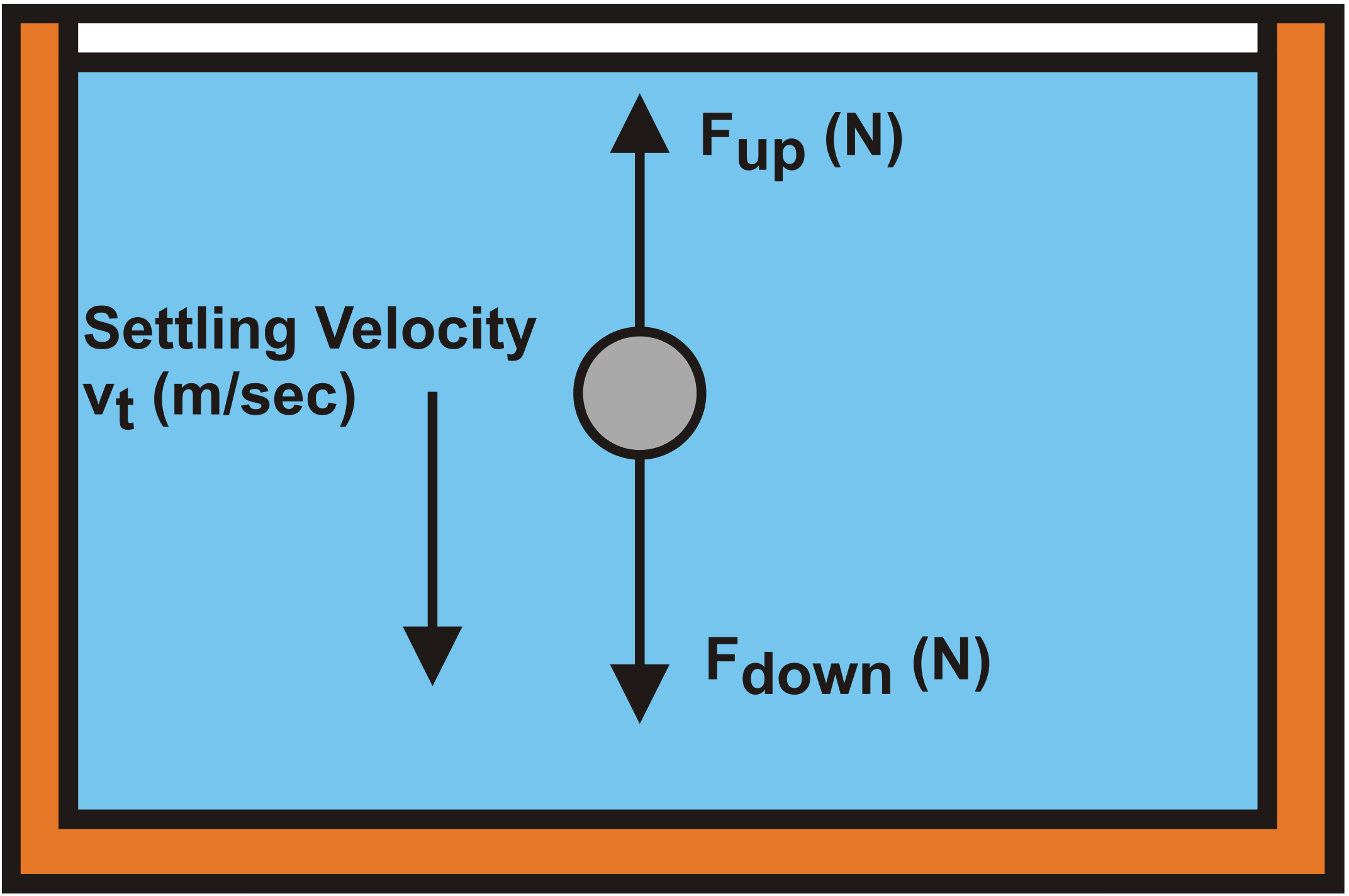
The upward directed force on the particle, caused by the frictional drag of the liquid, can be calculated by:
\[\ \mathrm{F}_{\mathrm{u p}}=\mathrm{C}_{\mathrm{D}} \cdot \frac{\mathrm{1}}{\mathrm{2}} \cdot \rho_{\mathrm{l}} \cdot \mathrm{v}_{\mathrm{t}}^{2} \cdot \mathrm{A}\]
The downward directed force, caused by the difference in density between the particle and the water can be calculated by:
\[\ \mathrm{F}_{\mathrm{down}}=\left(\rho_{\mathrm{s}}-\rho_{\mathrm{l}}\right) \cdot \mathrm{g} \cdot \mathrm{V} \cdot \psi\]
In this equation a shape factor ψ is introduced to compensate for the shape of real sand grains. This shape factor is 1 for spheres and about 0.7 for real sand particles. The projected surface of the particle is:
\[\ \mathrm{A}=\frac{\pi}{4} \cdot \mathrm{d}^{2}\]
The volume of the particle is:
\[\ \mathrm{V}=\frac{\pi}{6} \cdot \mathrm{d}^{3}\]
In general, the terminal settling velocity vt can be determined with the following equation:
\[\ \mathrm{v}_{\mathrm{t}}=\sqrt{\frac{\mathrm{4} \cdot \mathrm{g} \cdot\left(\rho_{\mathrm{s}}-\rho_{\mathrm{l}}\right) \cdot \mathrm{d} \cdot \mathrm{\psi}}{\mathrm{3} \cdot \rho_{\mathrm{l}} \cdot \mathrm{C}_{\mathrm{D}}}}\]
The Reynolds number of the settling process determines whether the process is laminar or turbulent. The Reynolds number can be determined by:
\[\ \mathrm{R e}_{\mathrm{p}}=\frac{\mathrm{v}_{\mathrm{t}} \cdot \mathrm{d}}{v_{\mathrm{l}}}\]


