1.8: Deflections of Structures- Work-Energy Methods
- Page ID
- 17614
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)Deflections of Structures: Work-Energy Methods
The virtual work method, also referred to as the method of virtual force or unit-load method, uses the law of conservation of energy to obtain the deflection and slope at a point in a structure. This method was developed in 1717 by John Bernoulli. To illustrate the principle of virtual work, consider the deformable body shown in Figure 8.1. First, applying a virtual or fictitious unit load Pv = 1 at a point Q, where the deflection parallel to the applied load is desired, will create an internal virtual or imaginary load f and will cause point Q to displace by a certain small amount. Then, placing the real external loads P1, P2, and M on the same body will cause an internal deformation, dS, and an external deflection of point Q to Q’ by an amount ∆.

Upon placement of the real load, the point of application of the virtual load also displaces by ∆, and the applied unit load performs work by traveling the distance ∆. The work done by the virtual forces are as follows:
External work done by the unit load Pv

Internal work done by the virtual load f

Applying the principle of conservation of energy by equating equation 8.1 and equation 8.2 suggests the following:
External work done = Internal work done

where
Pv = 1 = external virtual unit load.
f = internal virtual load.
∆ = external displacement caused by real loads.
dS = internal deformation caused by real loads.
Similarly, to obtain the slope at a point on a structure, apply a unit virtual moment Mv at the specified point where the slope is desired, and apply the following equation derived via the principle of conservation of energy:

where
Mv = 1 = external virtual unit moment.
f = internal virtual load.
θ = external rotational displacement caused by real loads.
dS = internal deformation caused by real loads.
8.1.1 Virtual Work Formulation for the Deflection and Slope of Beams and Frames
To develop the equations for the computation of deflection of beams and frames using the virtual work principles, consider the beam loaded as shown in Figure 8.2a. The deflection at point C due to the applied external loads is required. First, removing the loads P and W and applying a virtual unit load Pv = 1 will cause elementary forces and deformations to develop in the bar, and a small deflection to occur at C, as follows:

The stress acting on the differential cross-sectional area dA at a distance x from the left-end support due to a virtual unit load is as follows:

where
m = internal virtual moment at the section at a distance xe from the left-end support due to the virtual unit load.
I = moment of inertia of the section.
The force acting on the differential area due to the virtual unit load is written as follows:

The stress due to the external loads P1 and P2 on the beam is written as follows:

The deformation of a differential beam length dx at a distance x from the left-end support is as follows:

The work done by the force f acting on the differential area due to the deformation of the differential beam length dx is as follows:

The internal work done by the total force in the entire cross-sectional area of the beam due to the applied virtual unit load when the differential length of the beam dx deforms by δ can be obtained by integrating with respect to dA, as follows:

The internal work done Wi in the entire length of the beam due to the applied virtual unit load can now be obtained by integrating with respect to dx, which is written as follows:

The external work done We by the virtual unit load due to the deflection ∆ at point C of the beam caused by the external loads is as follows:

The principle of conservation of energy is applied to obtain the expression for the computation of the deflection at any point in a beam or frame, which is written as follows:

where
1 = external virtual or imaginary unit load on the beam or frame in the direction of the required deflection ∆.
∆ = external displacement at the specified point on a beam or frame caused by the real loads.
M = internal moment in the beam or frame caused by the real load, expressed in terms of the horizontal distance x.
m = internal virtual moment in the beam or frame caused by the external virtual unit load, expressed with respect to the horizontal distance x.
E = modulus of elasticity of the material of the beam or frame.
I = moment of inertia of the cross-sectional area of the beam or frame about its neutral axis.
Similarly, the following expression can be obtained for the computation of the slope at a point in a beam or frame:

where
θ = slope or tangent rotation at a point on a beam or frame.
mθ = internal virtual moment in the beam or frame, expressed with respect to the horizontal distance x, caused by the external virtual unit moment applied at the point where the rotation is required.
Procedure for Determination of Deflection in Beams and Frames by the Virtual Work Method
•Determine the support reactions in the real system using the equations of static equilibrium.
•Write an expression for the moment in the real structure as a function of the horizontal distance x. The number of the equations will depend on the number of regions of the beam due to discontinuous loading.
•Create a virtual system by removing all the loads acting on the beam and applying a unit load or a unit moment at the point where the deflection or slope is desired.
•Write the moment expression for the virtual system in terms of the distance x.
•Substitute the moment expressions into equation 8.1 and integrate to obtain the value of deflection or slope at the point considered.
8.1.2 Virtual Work Formulation for the Deflection of Trusses
Consider the truss shown in Figure 8.3 for the development of the virtual work expression for the determination of the deflection of trusses. The truss is subjected to the loads P1, P2, and P3, and the vertical deflection ∆ at joint F is desired. First, remove the loads P1, P2, and P3, and apply a vertical virtual unit load Pv = 1 at joint F, as shown in Figure 8.3b. The virtual unit load will cause the virtual internal axial load ni to act on each member of the truss. Applying the forces P1, P2, and P3 will cause the deflection ∆ at joint F and the internal deformation δLi in each member of the truss.

Using the law of conservation of energy, the work by the virtual unit load at joint F and the virtual internal axial loads on the members of the truss can be written as follows:
External work = internal work

But, for a member with length Li, area Ai, and material Young’s modulus Ei, the deformation is written as follows:

Thus, the virtual work expression for the deflection of a truss can be written as follows:

where
1 = external vertical virtual unit load applied at joint F.
n = internal axial virtual force in each truss member due to the virtual unit load, Pv = 1.
N = axial force in each truss member due to the real loads P1, P2, and P3.
∆ = external joint displacement caused by the real loads.
δL = deformation of each truss member caused by the real loads.
Procedure for Determination of Deflection in Trusses by the Virtual Work Method
•Determine the support reactions in the real system with the applied loads using the equations of equilibrium.
•Determine the internal forces N in truss members caused by the external loads on the real system.
•Remove all the external loads on the real system and apply a virtual unit load on the joint in the truss in the direction of required deflection.
•Determine the internal virtual forces n in the members of the truss caused by the external virtual unit load placed in the joint where the deflection is desired.
•Calculate the deflection ∆ in the joint of the truss caused by the real loads using equation 8.17.
Example 8.1
Using the virtual work method, determine the deflection and the slope at a point B of the cantilever beam shown in Figure 8.4a. E = 29 × 103ksi, I = 600 in4.


Solution
Real and virtual systems. The real and virtual systems are shown in Figure 8.4a, Figure 8.4c, and Figure 8.4e, respectively. Notice that the real system consists of the external loading carried by the beam, as specified in the problem. The virtual system consists of a unit 1-k load applied at B, where the deflection is required, and 1-k-ft moment applied at the same point where the slope is determined. The bending moments at each portion of the beam, with respect to the horizontal axis, are presented in Table 8.1. Notice that the origin of the horizontal distance, x, for both the real and virtual system is at the free end, as shown in Figure 8.4b, Figure 8.4d, and Figure 8.4f.
Table 8.1. Bending moments at portions of the beam.
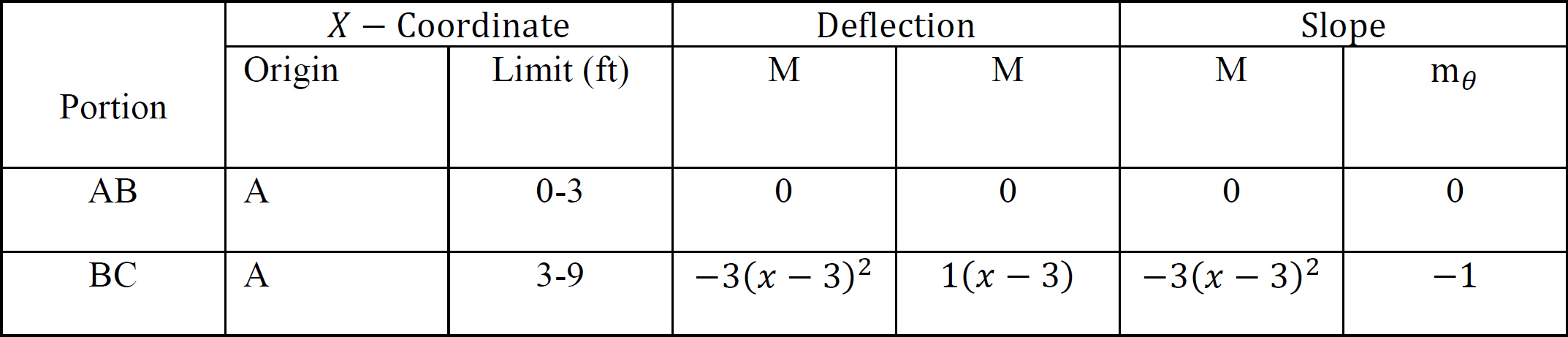
Deflection at B. The deflection at the free end of the beam is determined by using equation 8.1, as follows:
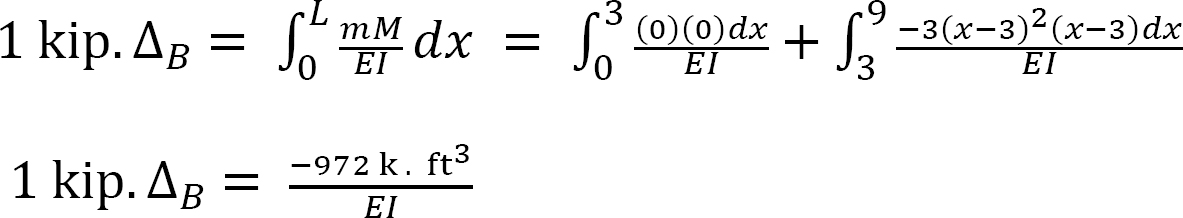
Therefore,
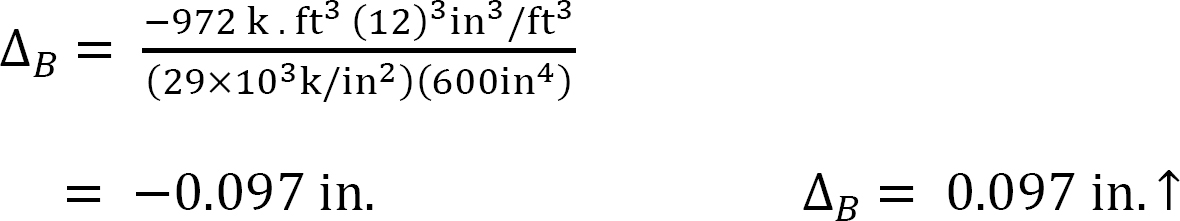
Slope at B. The slope at the free end of the beam is determined by using equation 8.2, as follows:
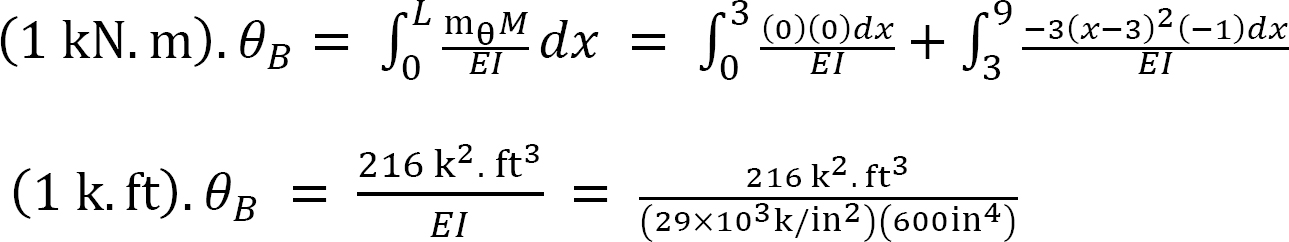
Therefore,
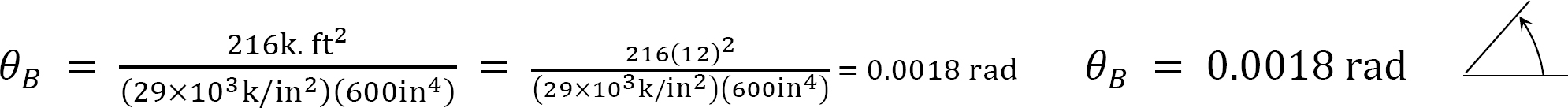
Example 8.2
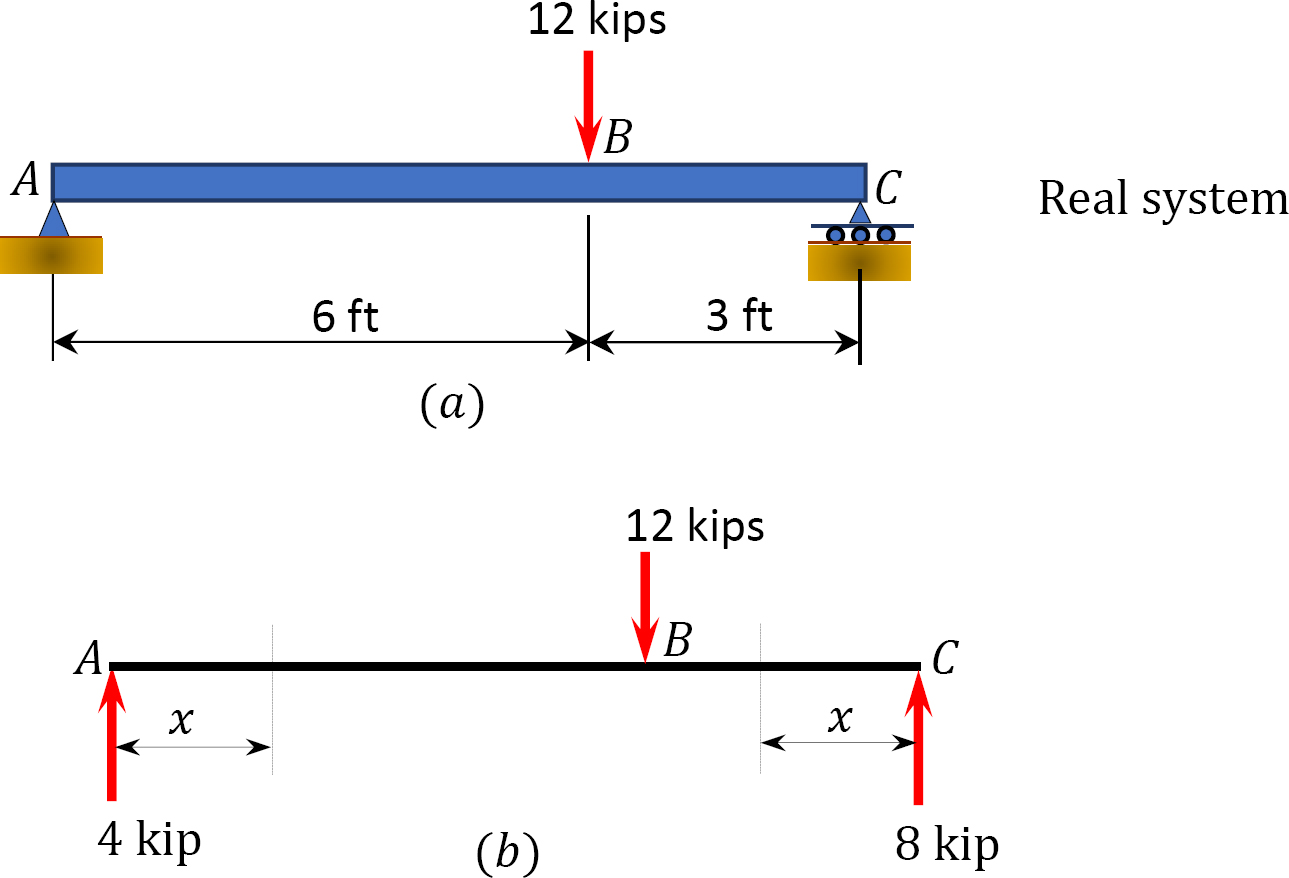
Using the virtual work method, determine the deflection at B and the slope at C for the simply supported beam subjected to a concentrated load, as shown in Figure 8.5a. EI= constant. E = 29 × 103ksi. I = 24 in4.

Solution
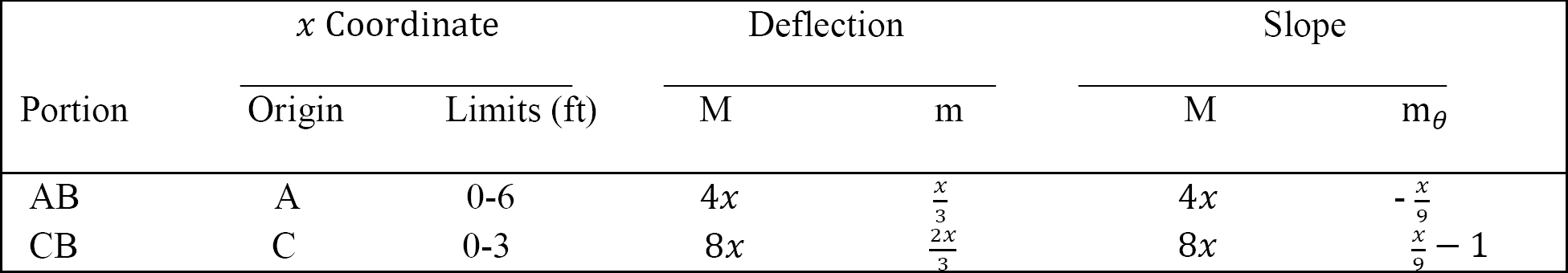
Real and virtual systems. The real and virtual systems are shown in Figure 8.5a, Figure 8.5c, and Figure 8.5e, respectively. The bending moments at each portion of the beam, with respect to the horizontal axis, are presented in Table 8.2. The origin of the horizontal distances for both the real and virtual system are shown in Figure 8.5b, Figure 8.5d, and Figure 8.5f.
Table 8.2. Bending moments at portions of the beam.
Deflection at B. The deflection at B can be determined by using equation 8.1, as follows:
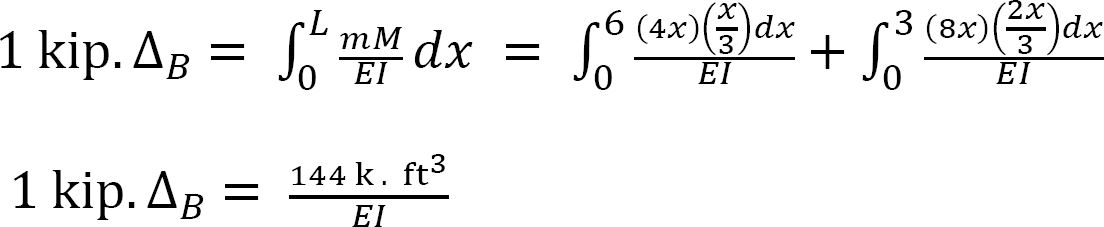
Therefore,
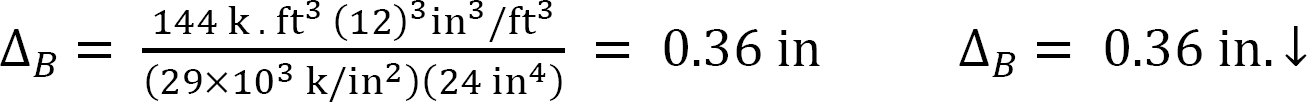
The positive value indicates deflection in the direction of the applied virtual load.
Slope at C. The slope at C can be determined by using equation 8.2, as follows:
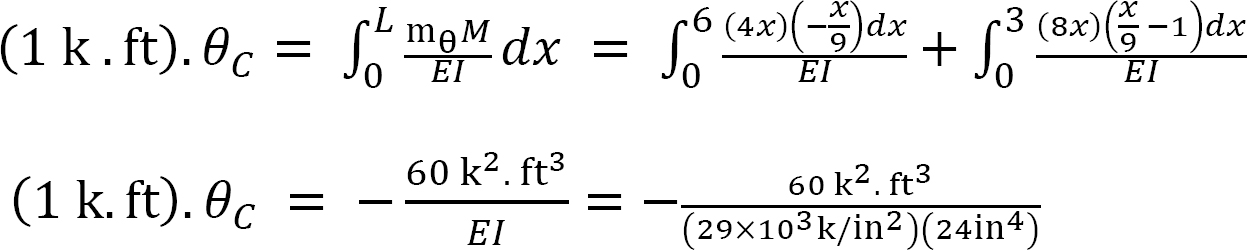
Therefore,
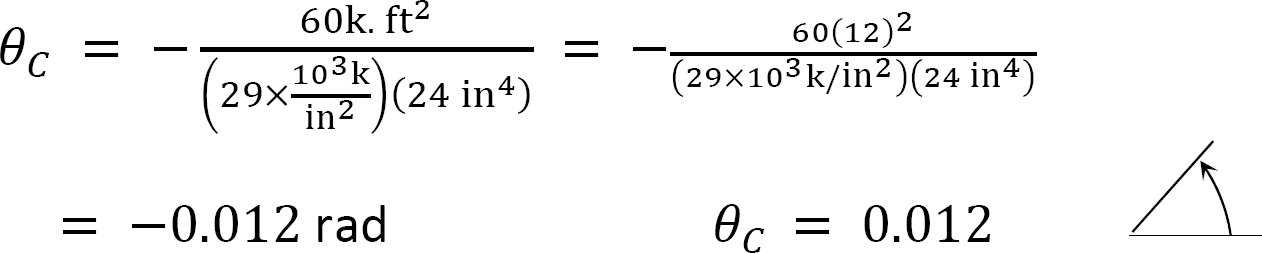
Example 8.3
Using the virtual work method, determine the deflection at B and the slope at D for the compound beam shown in Figure 8.6a. E = 200 GPa and I = 250 × 106 mm4.

Solution
Real and virtual systems. The real and virtual systems are shown in Figure 8.6a, Figure 8.6b, and Figure 8.6c, respectively. The bending moment at each portion of the beam, with respect to the horizontal axis, are presented in Table 8.3.
Table 8.3. Bending moments at portions of the beam.
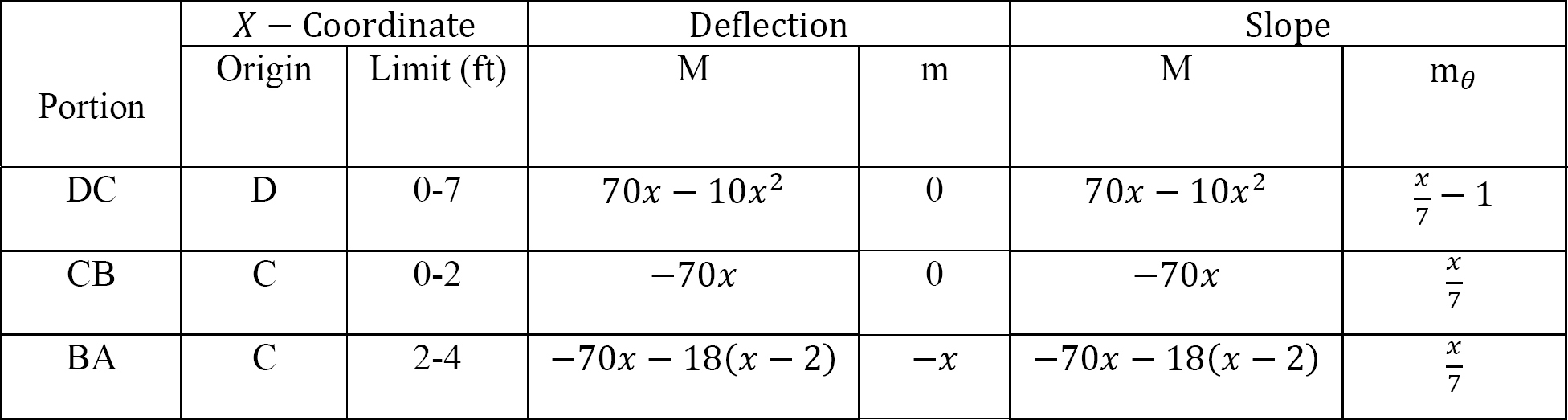
Deflection at B. The deflection at B can be determined using equation 8.1, as follows:
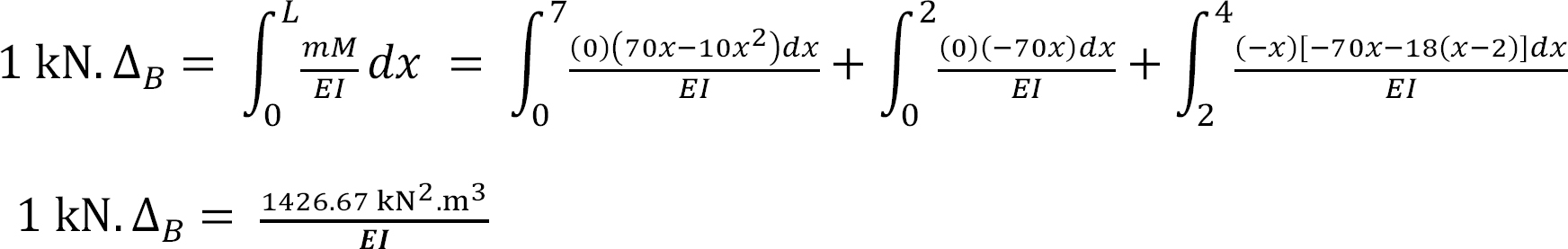
Therefore,
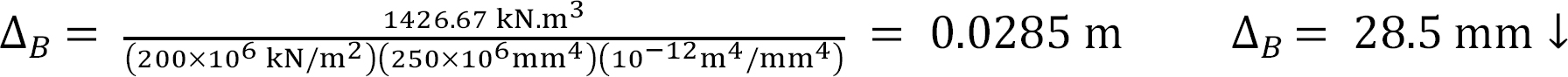
Slope at D. The slope at D can be determined using equation 8.2, as follows:
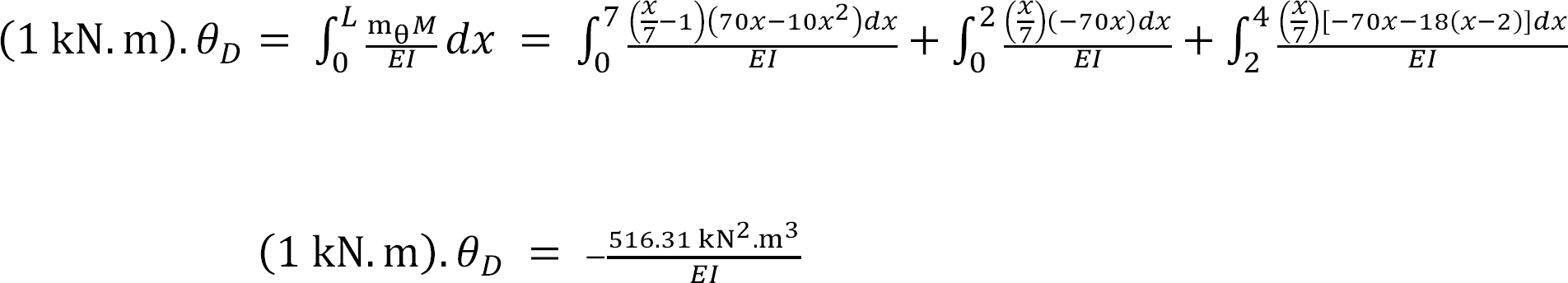
Therefore,
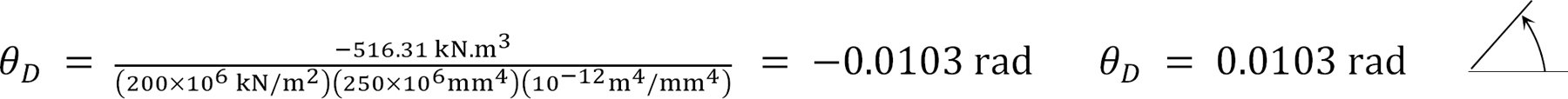
The negative sign indicates that the rotation at point D is in the direction opposite to the applied virtual moment.
Example 8.4
Using the virtual work method, determine the slope at joint A of the frame shown in Figure 8.7a. E = 29 × 103ksi and EI = 700 in4.
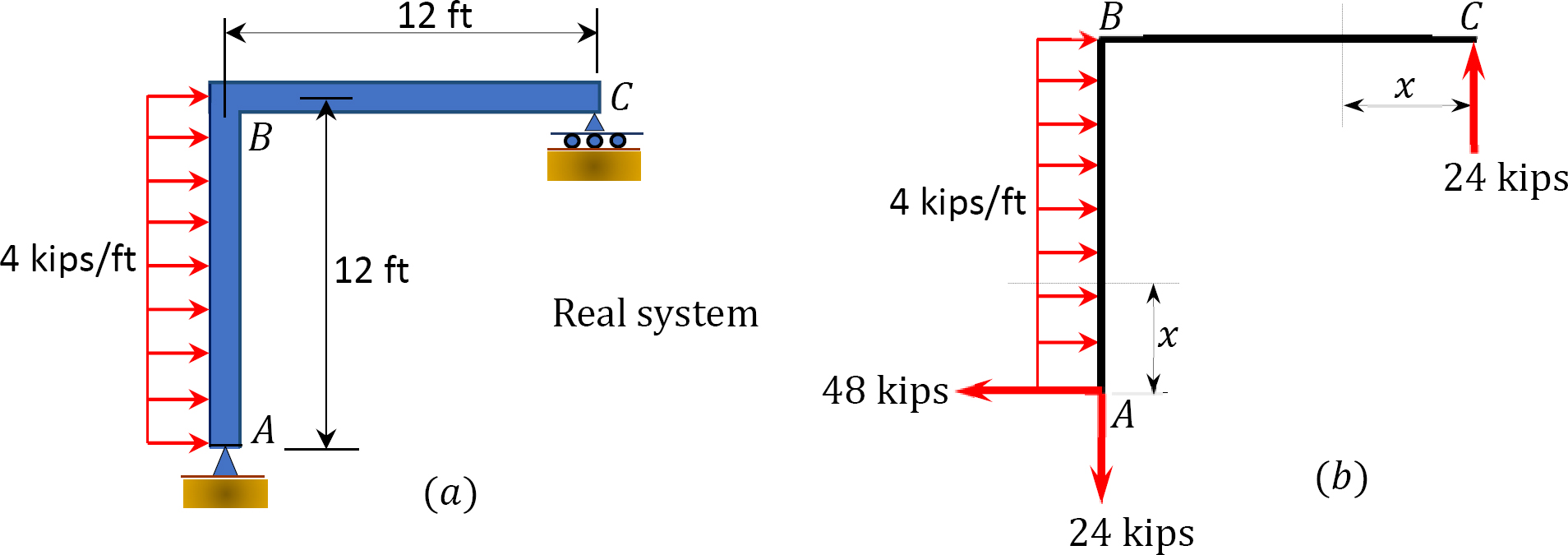

Solution
Real and virtual systems. The real and virtual systems are shown in Figure 8.7a and Figure 8.7c, respectively. The bending moment at each segment of the beam and column of the frame are presented in Table 8.4, and their origins are shown in Figure 8.7b and Figure 8.7d.
Table 8.4. Bending moments at portions of the beam.
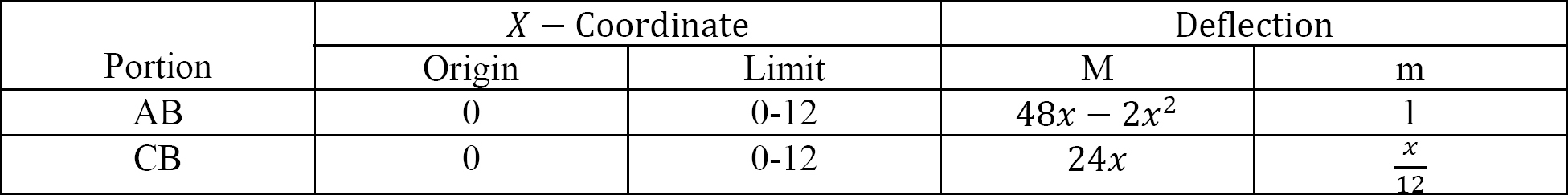
Slope at A. The slope at A can be determined by using equation 8.2, as follows:
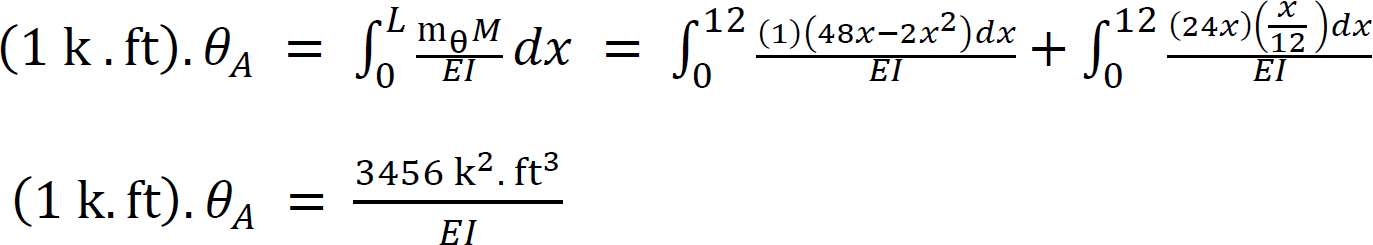
Therefore,
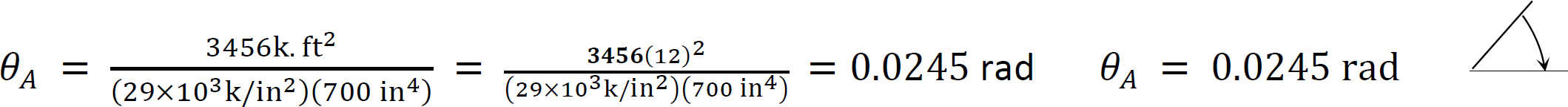
Example 8.5
Using the virtual work method, determine the vertical deflection at A of the frame shown in Figure 8.8a. E = 200 GPa and I = 250 × 106 mm4.

Solution
Real and virtual systems. The real and virtual systems are shown in Figure 8.8a and Figure 8.8c, respectively. The bending moment at each segment of the beam and column of the frame are presented in Table 8.5, and their origins are shown in Figure 8.8b and Figure 8.8d.
Table 8.5. Bending moments at portions of the beam.
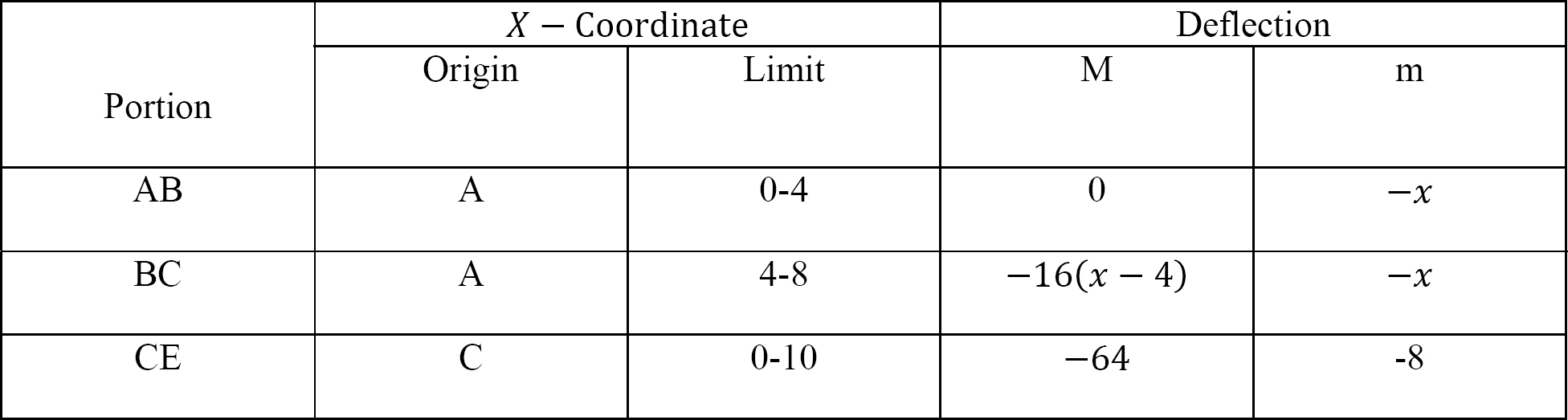
Deflection at A. The deflection at A can be determined by using equation 8.1, as follows:
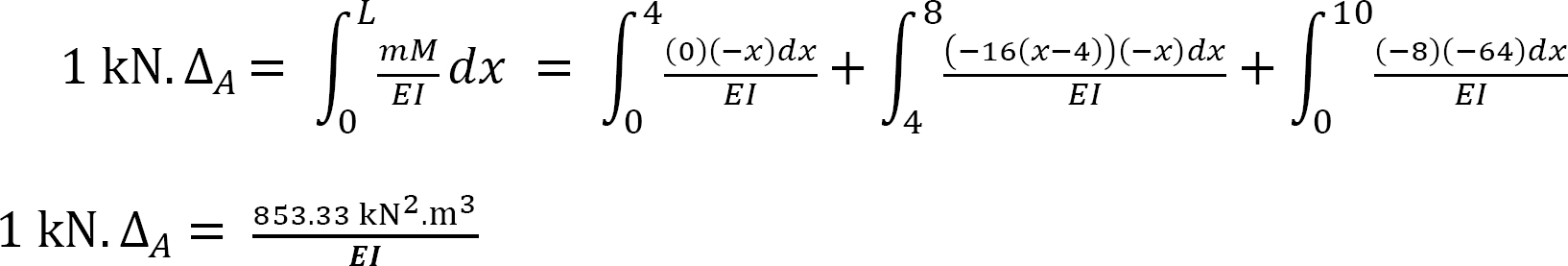
Therefore,

Example 8.6
Using the virtual work method, determine the horizontal deflection at joint B of the truss shown in Figure 8.9a. E = 12000 ksi and A = 3 in2.
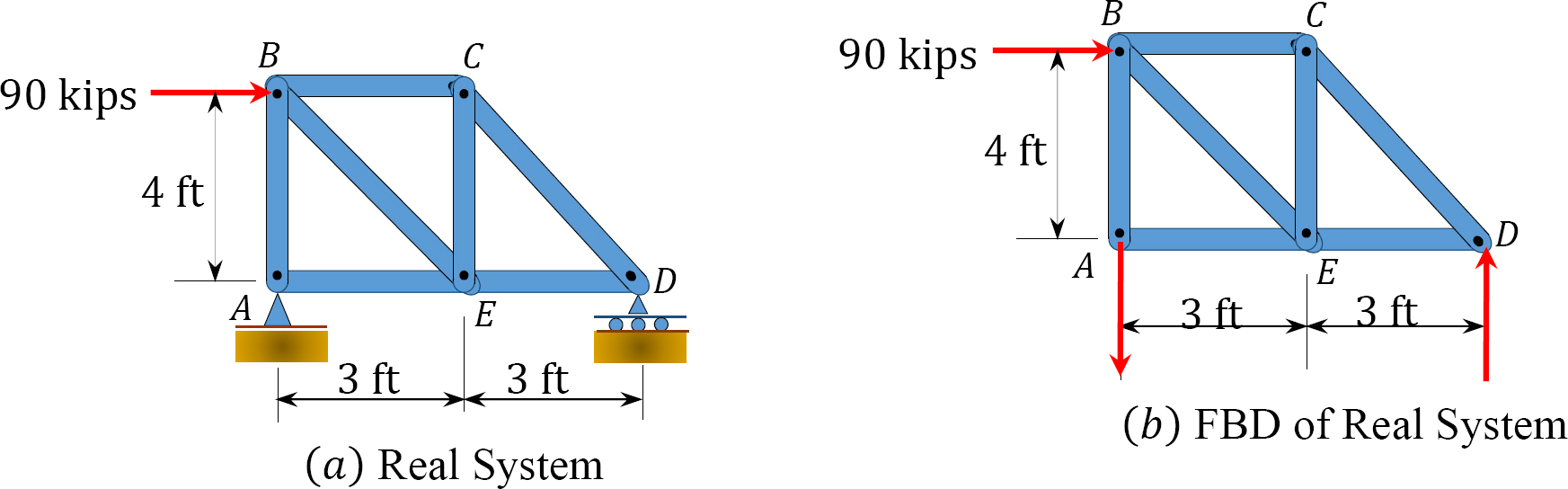

Solution
Real and virtual systems. The real and virtual systems are shown in Figure 8.9. Notice that the real system consists of the external loading carried by the truss, as specified in the problem. The virtual system consists of a unit 1-k load applied at B, where the deflection is desired, and a 1-k-ft moment applied also at B, where the slope is required.
Truss analysis. The analysis of the real system used to obtain the forces in members is presented below. The forces in members in the virtual system are obtained by dividing the forces in the real system by the applied external load, as the deflection is desired for the same joint where the deflection is required.
Support reactions. The reactions are computed by the application of the equations of equilibrium, as follows:

Joint A.

+ → ∑ Fx = 0
FAE – 90 = 0
FAE = 90 kips
Joint B.
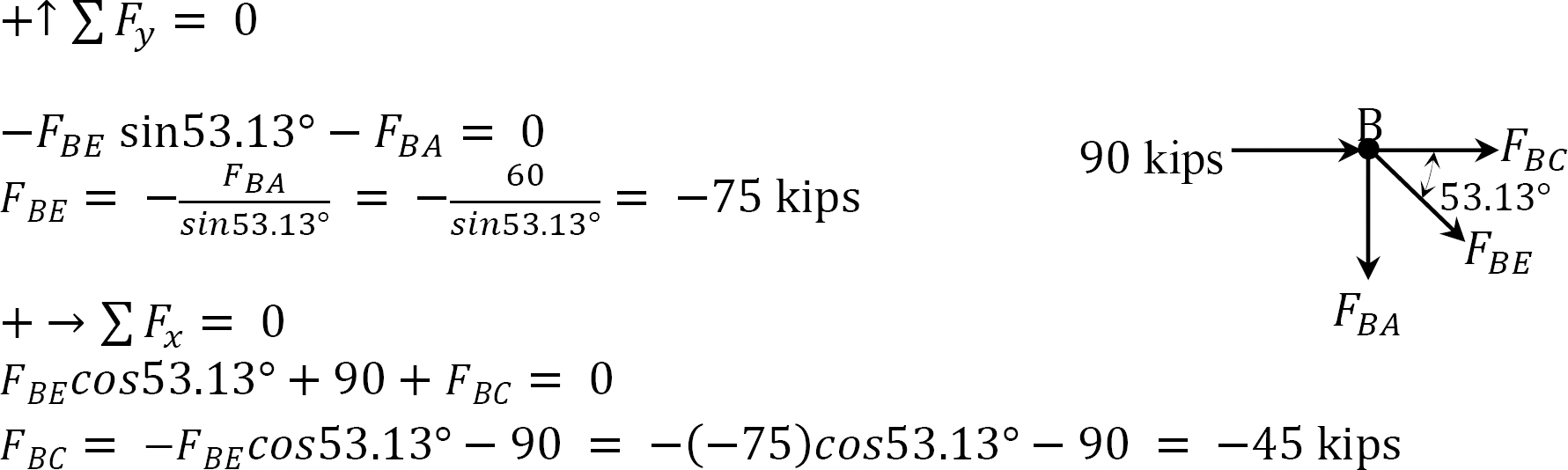
Joint C.

Joint D.
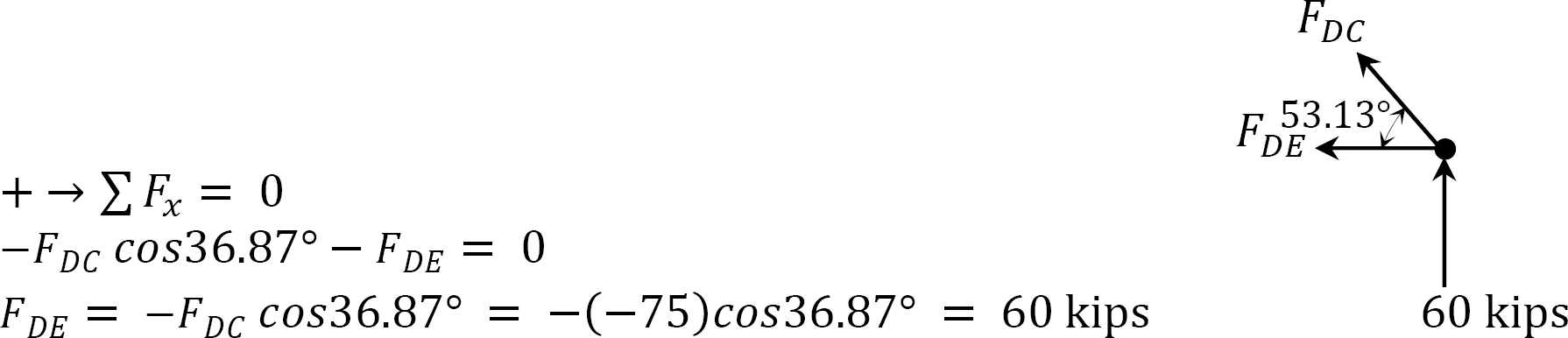

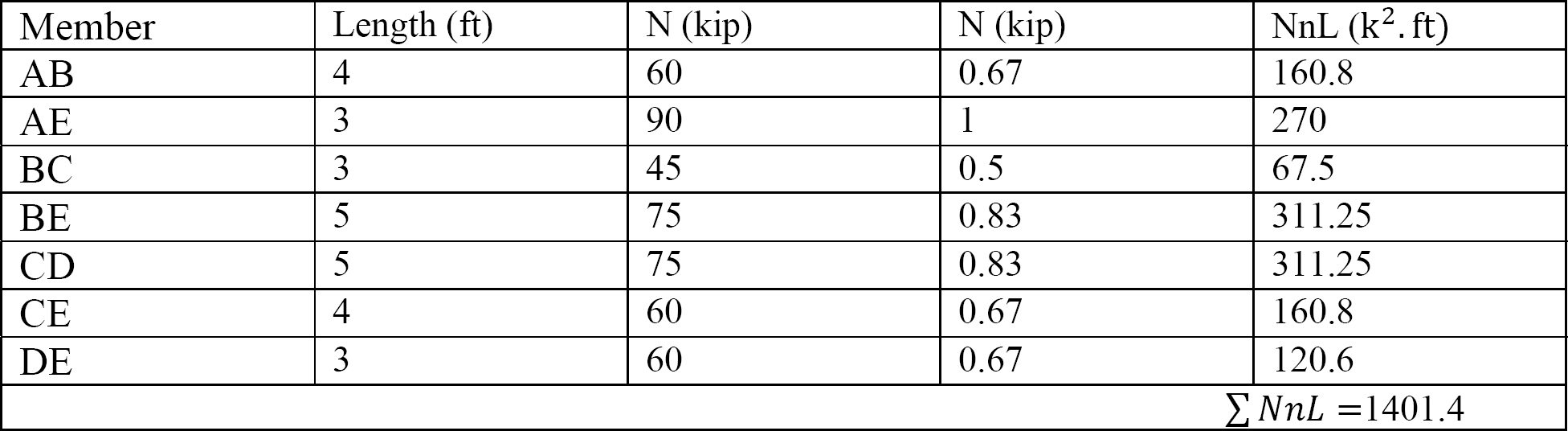
Horizontal deflection at B. The desired horizontal deflection at joint B is computed using equation 8.17, as presented in Table 8.6.
Table 8.6. Horizontal deflections.

Example 8.7
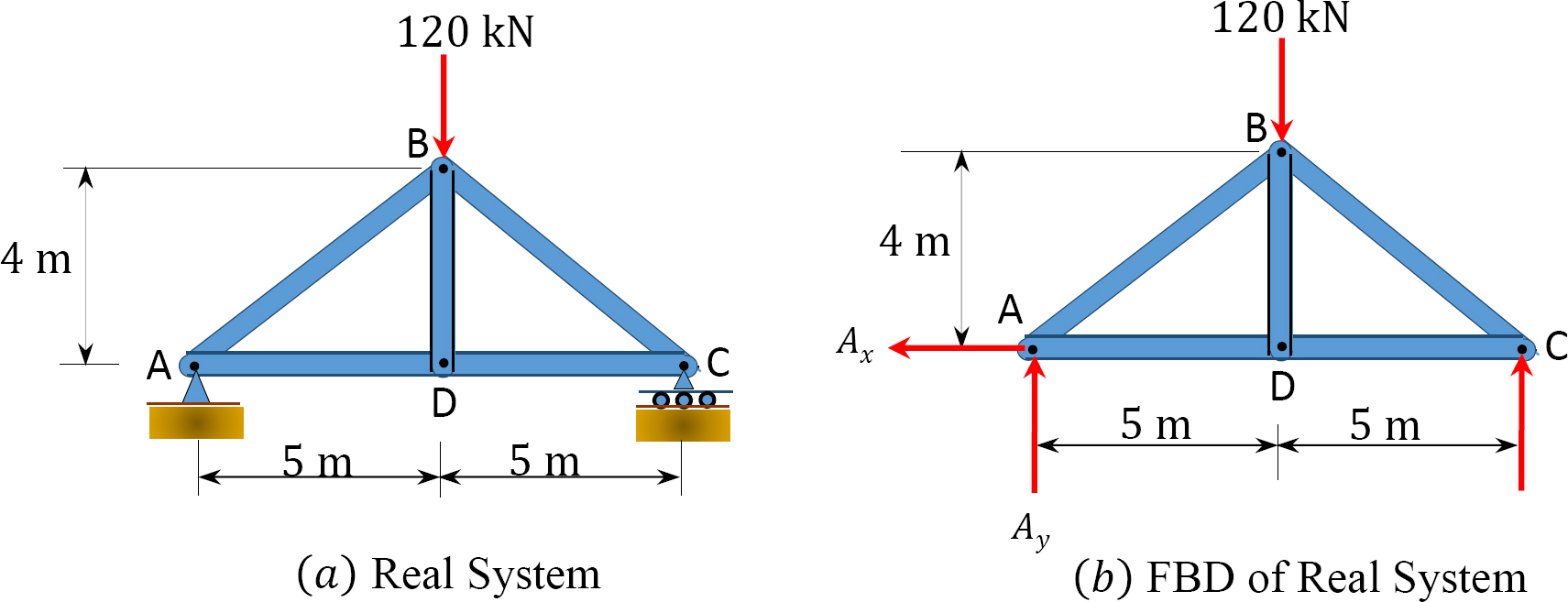
Using the virtual work method, determine the vertical deflection at joint D of the truss shown in Figure 8.10a. E = 200 GPa and A = 5 cm2.

Solution
Real and virtual systems. The real and virtual systems are shown in Figure 8.10. Notice that the real system consists of the external loading carried by the beam, as specified in the problem. The reactions in both supports in the real system are the same by reason of symmetry in loading and equal 60 kN. The virtual system consists of a unit 1-k load applied at B, where the deflection is required, and a 1-k-ft moment applied at the same point, where the slope is to be determined. The bending moment at each portion of the beam with respect to the horizontal axis is presented in Table 8.7. Notice that the origin of the horizontal distance x for both the real and virtual system is at the free end, as shown in Figure 8.10.
Real system-truss analysis.
Joint A.

Joint D.
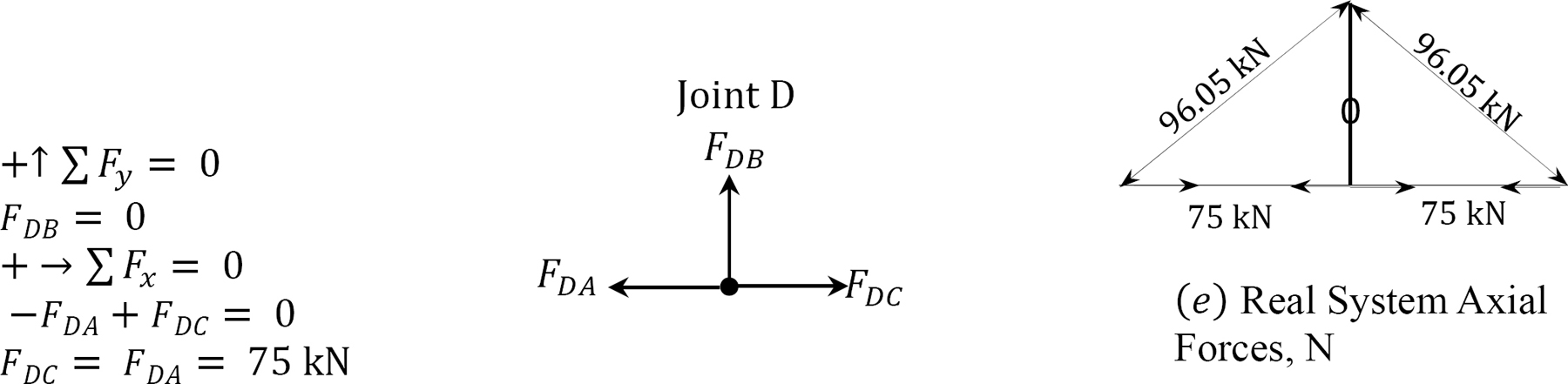
Virtual system truss analysis.
Joint A.
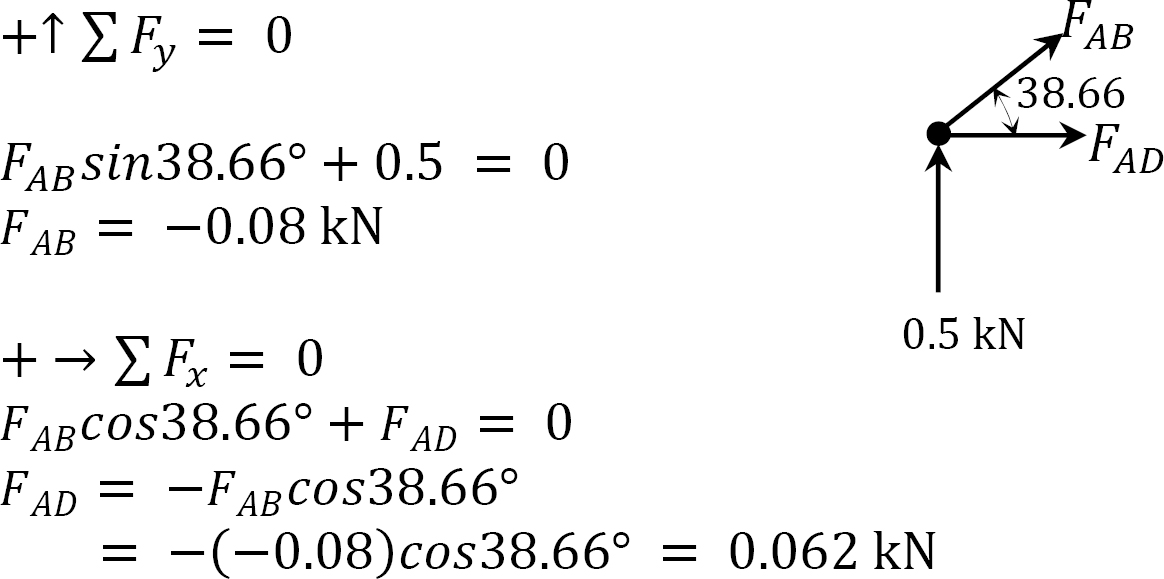
Joint D.
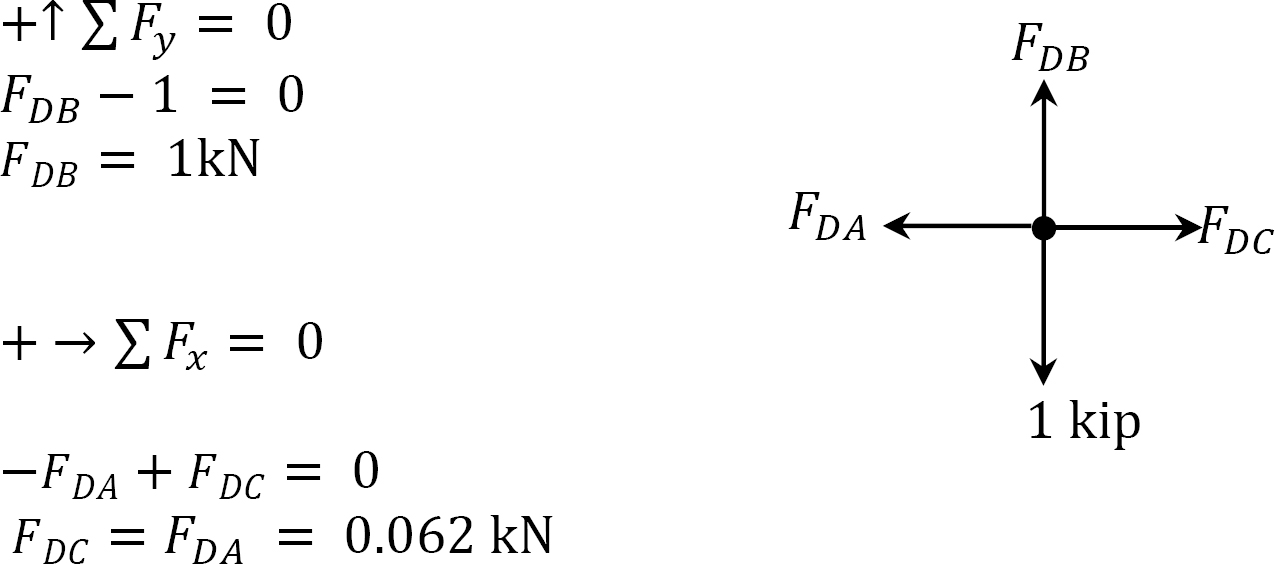
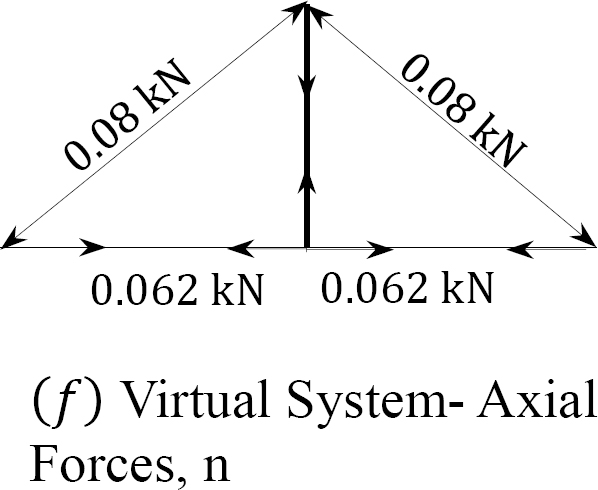
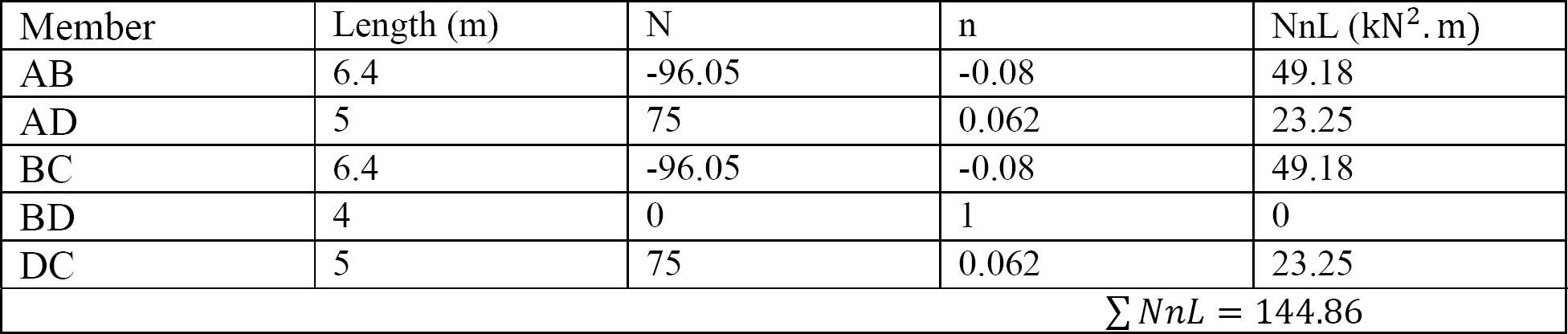
Vertical deflection at D. The desired vertical deflection at joint D is calculated using equation 8.17, as presented in Table 8.7.
Table 8.7. Vertical deflections.

The energy method for the determination of deflection is based on Alberto Castigliano’s second theorem, which was published in 1879. The theorem states the following:
The deflection or rotation in a specified direction and at a specified point in a linear elastic, statically determinate structure subjected to a given force or couple is equal to the partial derivative of the total external work or the total internal energy, with respect to the applied force or couple in the direction of the force or couple.
Castigliano’s second theorem, with respect to the applied force, can be expressed mathematically, as follows:

where
∆ = deflection at the point of application of the load P in the direction of the load P.
W = work done.
U = strain energy.
8.2.1 Energy Method Formulation for Beams and Frames
Equation 8.18 can be mathematically manipulated to include moment and is written as follows:

The total internal work done or strain energy stored in a beam or frame due to gradually applied real loads can be expressed as follows:

The partial derivative of equation 8.20, with respect to the moment, is as follows:

Substituting equation 8.21 into equation 8.19 yields the following equation for the computation of deflection for beams and frames by the energy method:

With respect to the applied couple, Castigliano’s second theorem can be expressed mathematically as follows:

where
θ = rotation at the point of application and direction of the couple M′.
Equation 8.23 can be mathematically manipulated to include the moment, as follows:

Substituting equation 8.21 into equation 8.24 suggests the following equation for the computation of slopes for beams and frames by the energy method:

Example 8.8
Using Castigliano’s second theorem, determine the deflection and the slope at the free end of the cantilever beam shown in Figure 8.11a.

Solution
Placement of imaginary force P and couple M′. The force P and the moment M′ are placed at point A, where the deflection and slope are desired, as shown in Figure 8.11b and Figure 8.11c, respectively.
Bending moment. To determine the deflection, write the bending moment equation for the beam as a function of the force P. To determine the slope, write the bending moment equation for the beam as a function of M′. The x coordinates for the moment equations are also shown in Figure 8.11b and Figure 8.11c. Compute the partial derivatives 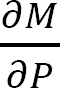 and
and 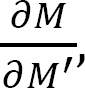 and then apply Castigliano’s equation 8.22 and equation 8.25 to determine the deflection and slope.
and then apply Castigliano’s equation 8.22 and equation 8.25 to determine the deflection and slope.
Deflection at A.
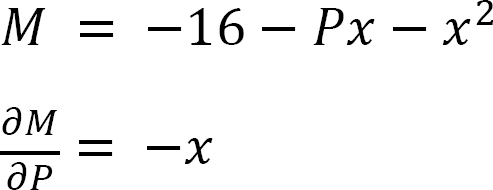
Setting P = 0 and applying Castigliano’s theorem suggests the following:
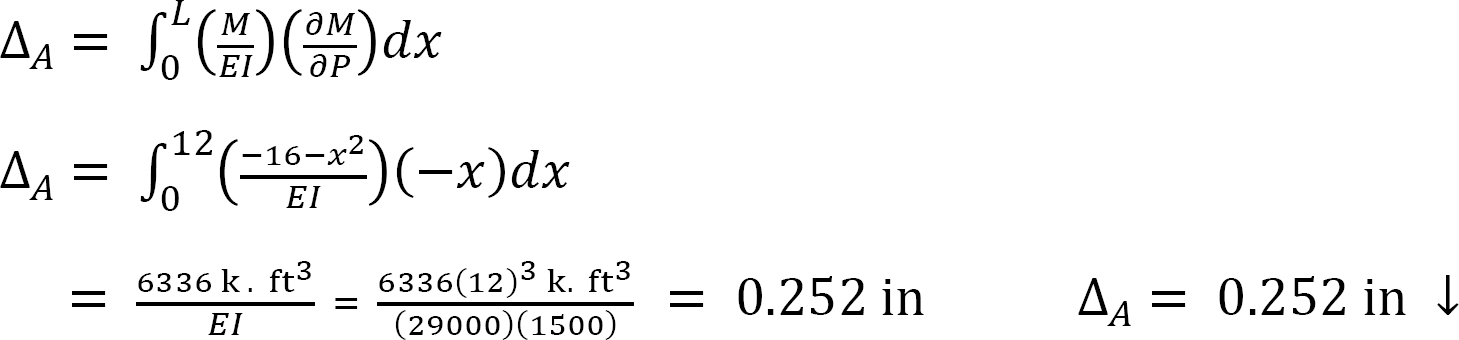
Slope at A.

Setting M′ = 16 k. ft and applying Castigliano’s theorem suggests the following:
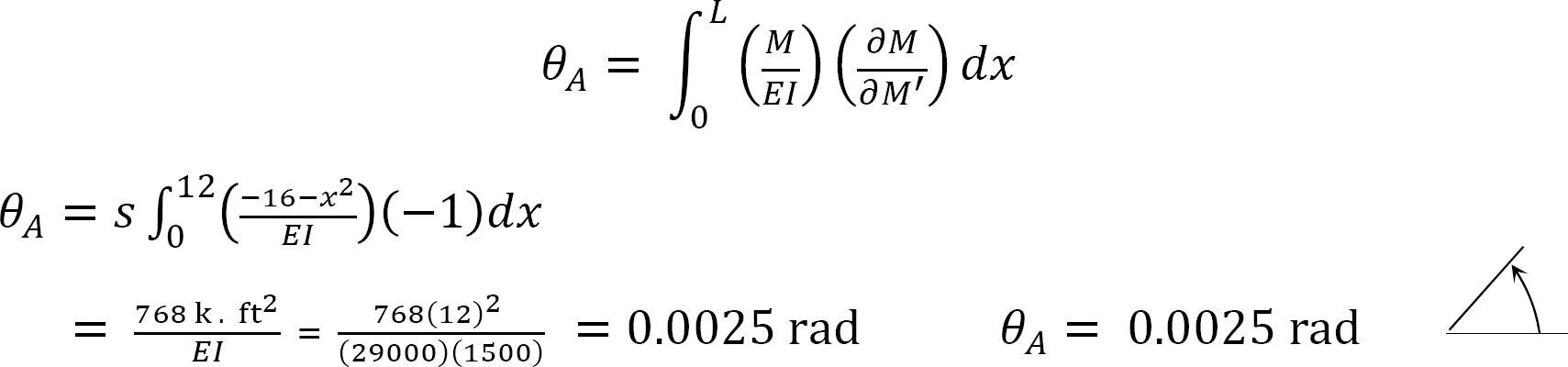
Example 8.9
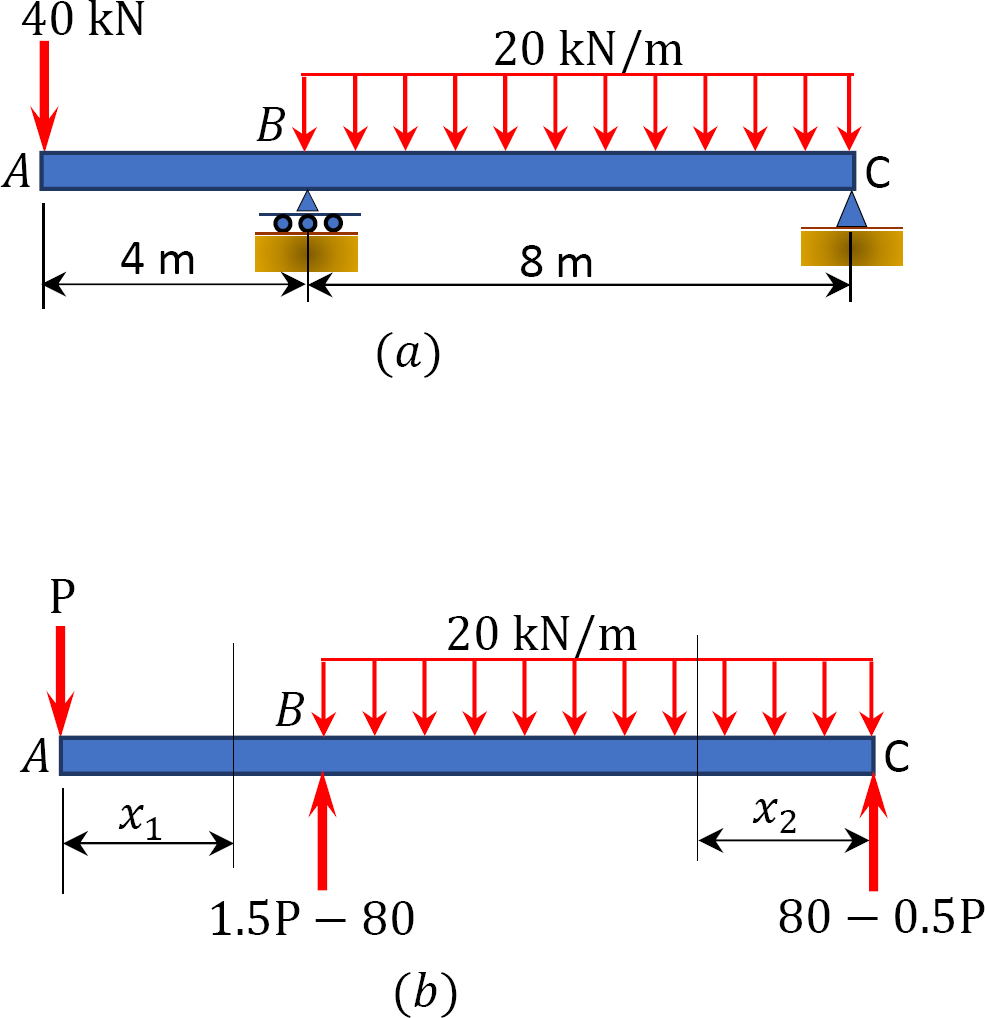
Using Castigliano’s second theorem, determine the deflection at point A of the beam with the overhang shown in Figure 8.12a.
Solution
Placement of imaginary force P. The force P is placed at point A, where the deflection is desired, as shown in Figure 8.12b. The x coordinates for the moment equations are also shown in this figure.
Bending moment. Compute the support reactions and write the bending moment equations for segments AB and BC of the beam as a function of the force P. The x coordinates for the moment equations are also shown in Figure 8.12b. Compute the partial derivatives 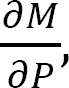 and then apply Castigliano’s equation 8.22 to compute the deflection.
and then apply Castigliano’s equation 8.22 to compute the deflection.
Segment AB. (0 < x1 < 4)
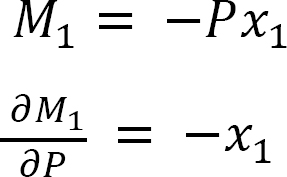
Segment BC. (0 < x2 < 8)
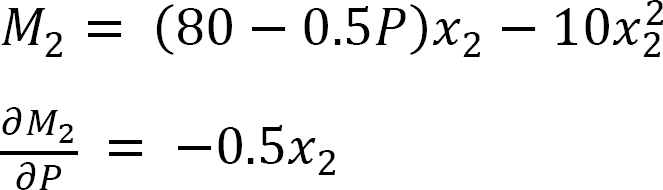
Setting P = 40 kN and applying Castigliano’s theorem suggests the following:
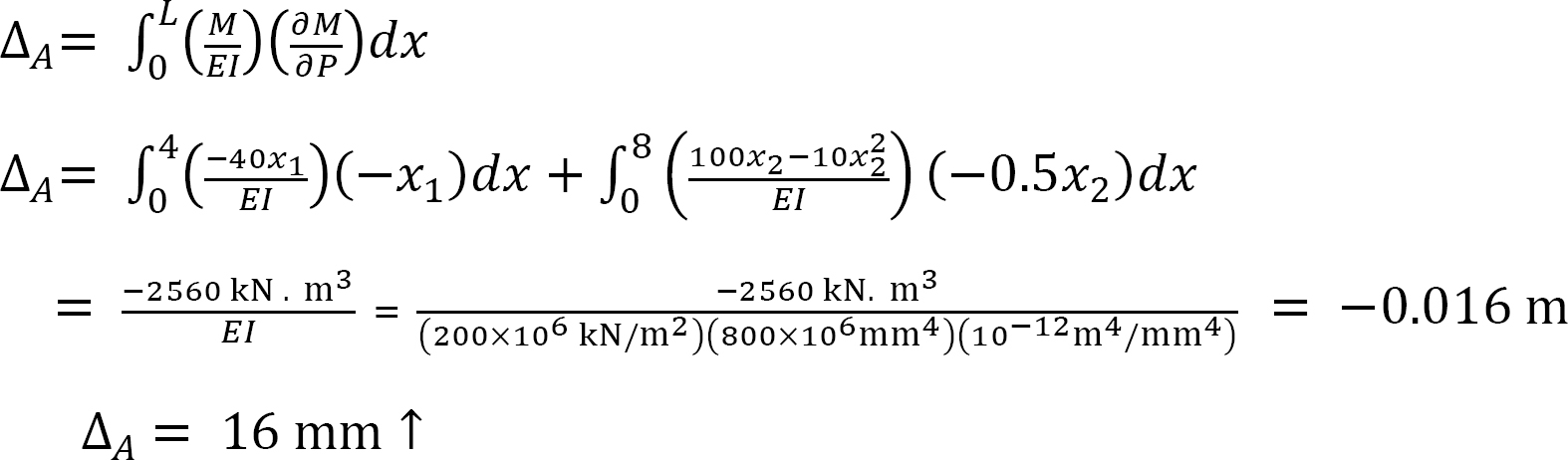
Example 8.10
Using Castigliano’s second theorem, determine the rotation of joint C of the frame shown in Figure 8.13a.

Solution
Placement of imaginary couple M′. The couple force M′ is placed at point C, where the rotation is desired, as shown in Figure 8.13b.
Bending moment. Compute the support reactions and write the bending moment equations for the columns AB and DC and the beam BC of the frame as a function of the couple M′. Compute the partial derivatives  and then apply Castigliano’s equation 8.25 for the computation of rotation.
and then apply Castigliano’s equation 8.25 for the computation of rotation.
Column AB. (0 < x1 < 10)
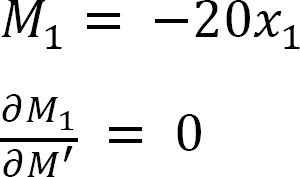
Beam BC. (0 < x2 < 8)
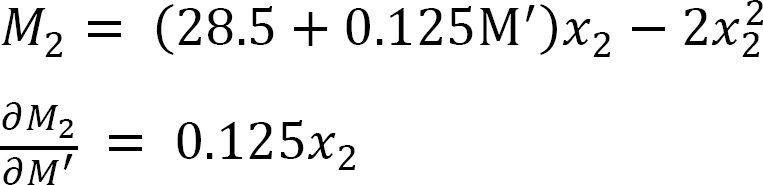
Column DC. (0 < x3 < 10)
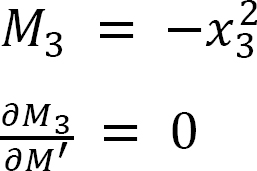
Setting M′ = 16 k. ft and applying Castigliano’s theorem suggests the following:
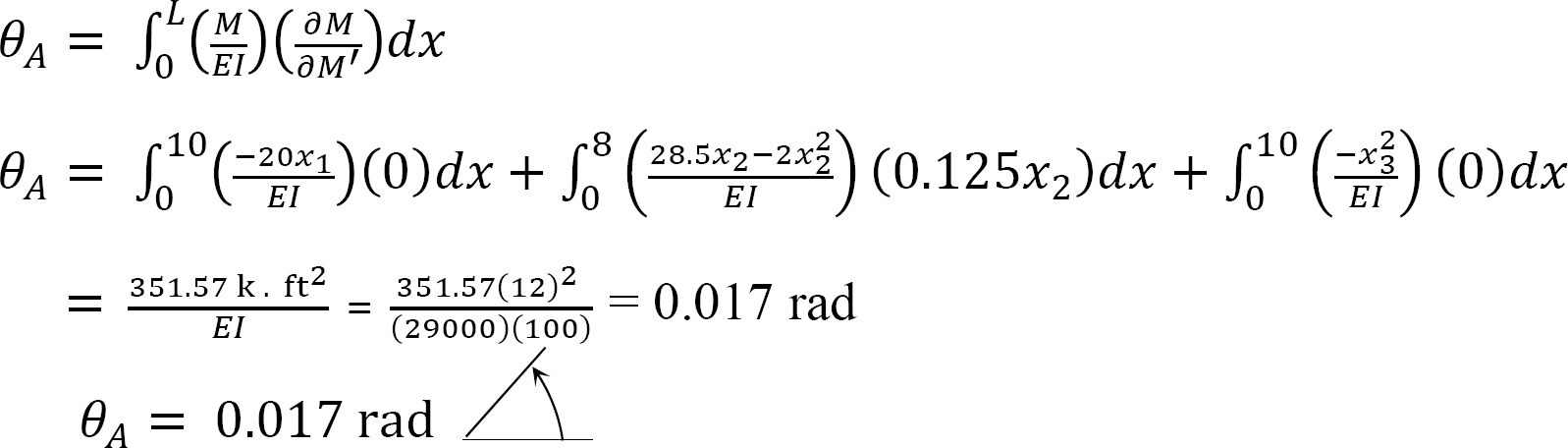
8.2.2 Energy Method Formulation for Trusses
Equation 8.18 can be mathematically manipulated to include axial force, as follows:

The total internal work done or strain energy stored in members of a truss due to gradually applied external loads is as follows:

The partial derivative of equation 8.27, with respect to the axial load, is as follows:

To determine the deflection at any joint of a truss, use the energy method by substituting equation 8.28 into equation 8.26 to obtain the following equation:

where
N = internal axial force in each member due to external load.
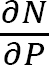 = axial force in each member due to unit load applied at the joint and in the direction of the required deflection.
= axial force in each member due to unit load applied at the joint and in the direction of the required deflection.
L = length of member.
A = area of a member.
E = modulus of elasticity of a member.
Example 8.11
Using Castigliano’s second theorem, determine the horizontal deflection at joint C of the truss shown in Figure 8.14a.

Solution
Placement of imaginary force P. The force P is placed as a replacement for the 30k force at point C, where the horizontal deflection is desired, as shown in Figure 8.14b.
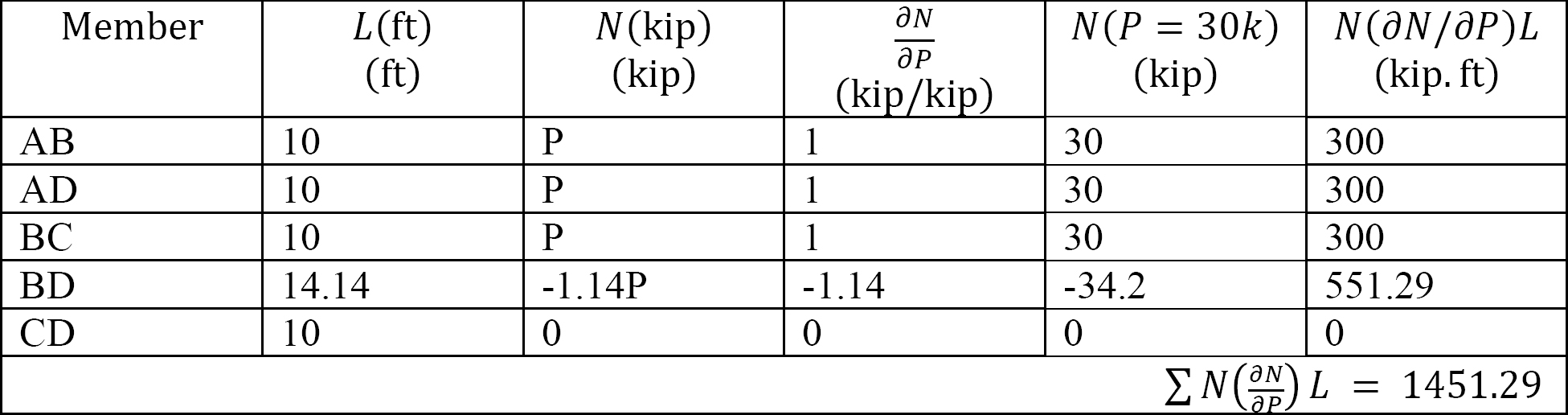
Member axial forces. Compute the support reactions and obtain the member-axial forces in terms of the imaginary force P. Member-axial forces are determined by using the method of joint, as shown below. To find the horizontal deflection at C, compute the partial derivatives 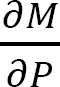 and apply Castigliano’s equation 8.22. Member lengths, axial forces, and partial derivatives with respect to the fictitious force P are shown in Table 8.8.
and apply Castigliano’s equation 8.22. Member lengths, axial forces, and partial derivatives with respect to the fictitious force P are shown in Table 8.8.
Analysis of truss (fig. 8.14b).
Joint A.

Joint B.

Joint C.

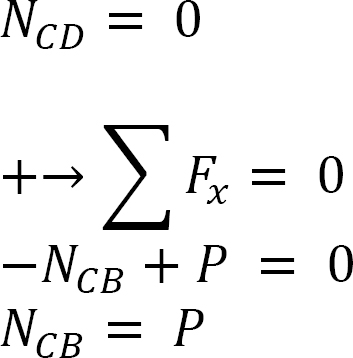
Table 8.8. Member lengths, axial forces, and partial derivatives with respect to the fictitious force P.
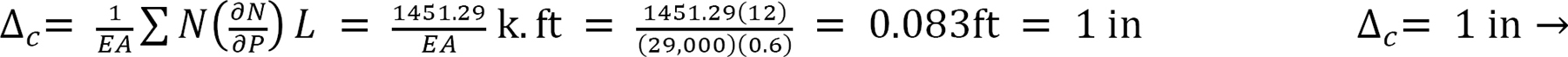
Example 8.12
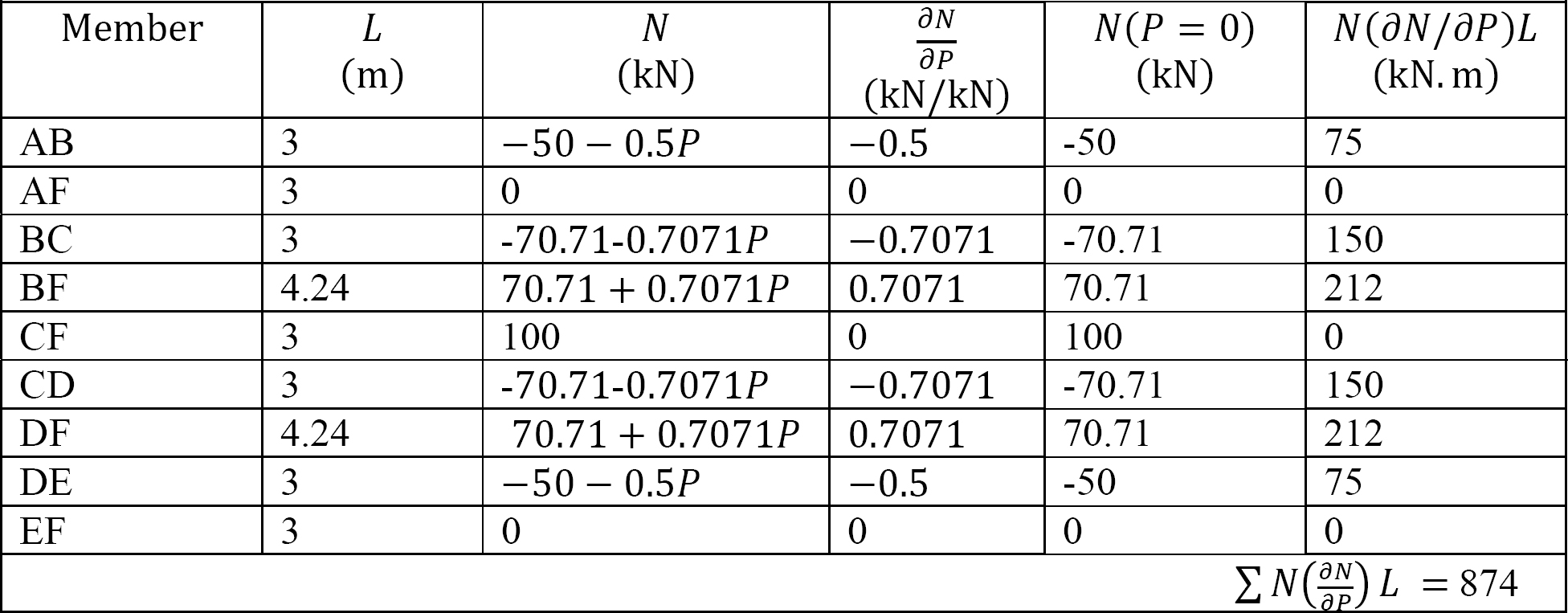
Using Castigliano’s second theorem, determine the vertical deflection at joint F of the truss shown in Figure 8.15a.. Members have the same cross-sectional area of 600 mm2 and E = 200 GPa.
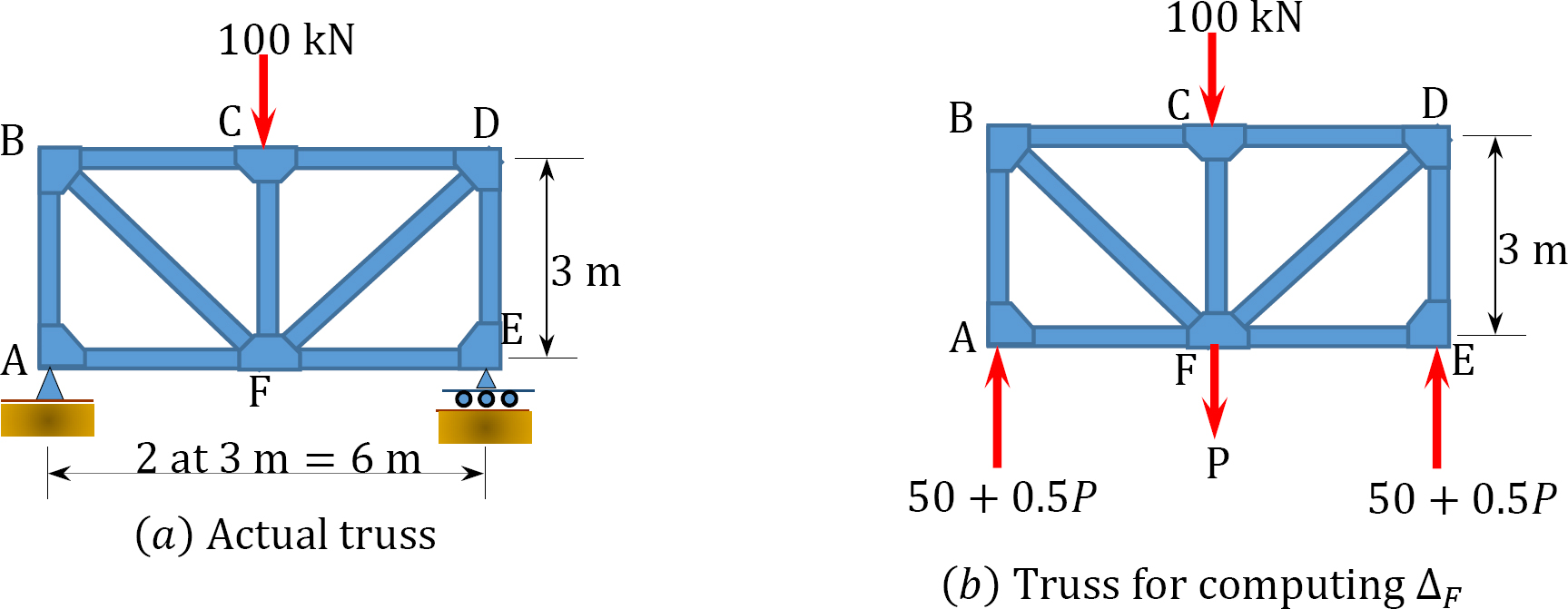
Solution
Placement of imaginary force P. The force P is placed at joint F, where the vertical deflection is desired, as shown in Figure 8.15b.
Member axial forces. Compute support reactions and obtain member-axial forces in terms of the imaginary force P. Member-axial forces are determined by using the method of joint, as shown below. To find the vertical deflection at F, compute the partial derivatives 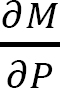 and apply Castigliano’s equation 8.22. Member lengths, axial forces, and partial derivatives with respect to the fictitious force P are shown in Table 8.9.
and apply Castigliano’s equation 8.22. Member lengths, axial forces, and partial derivatives with respect to the fictitious force P are shown in Table 8.9.
Analysis of truss (fig. 8.15b).
Joint A.

Joint B.
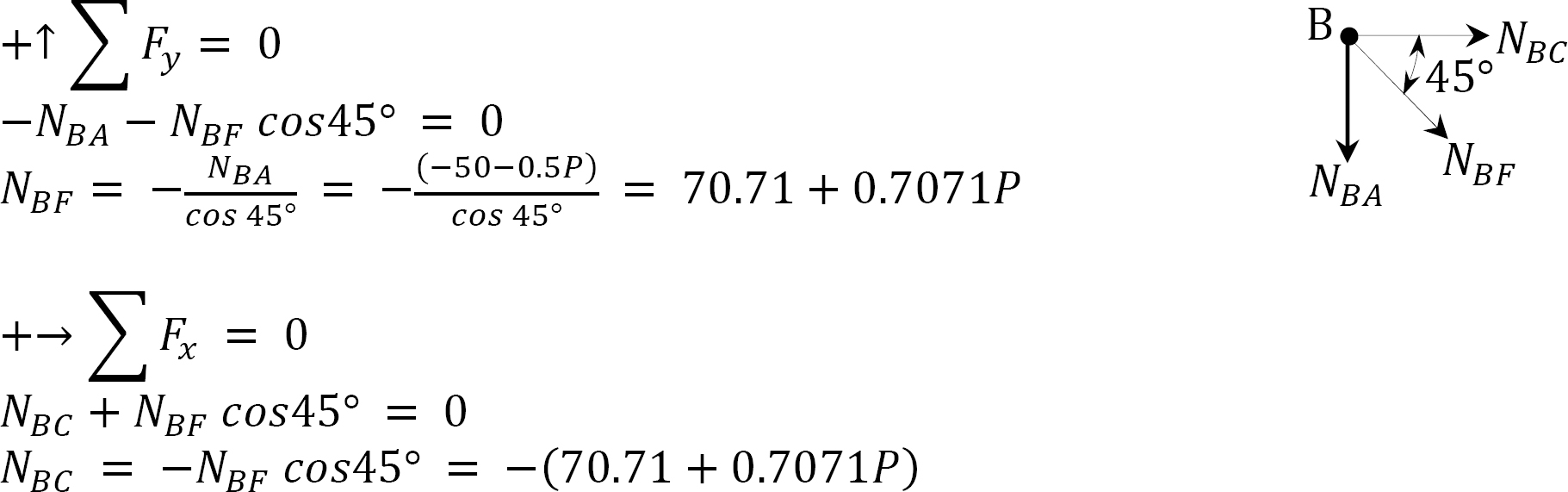
Joint C.

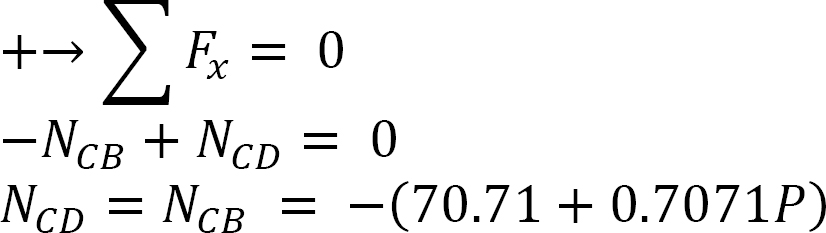
Table 8.9. Member lengths, axial forces, and partial derivatives with respect to the fictitious force P.
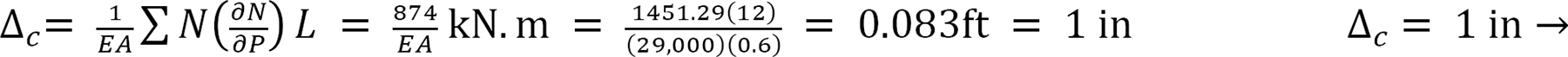
Chapter Summary
Principle of virtual work: The principle of virtual work states that if a body acted upon by several external forces is in a state of equilibrium and is subjected to a small virtual displacement, the virtual work done by the externally applied forces is zero. This principle can be expressed mathematically, as follows:
We = Wi
The expressions for the determination of deflection by virtual work method for beams and trusses are as follows:
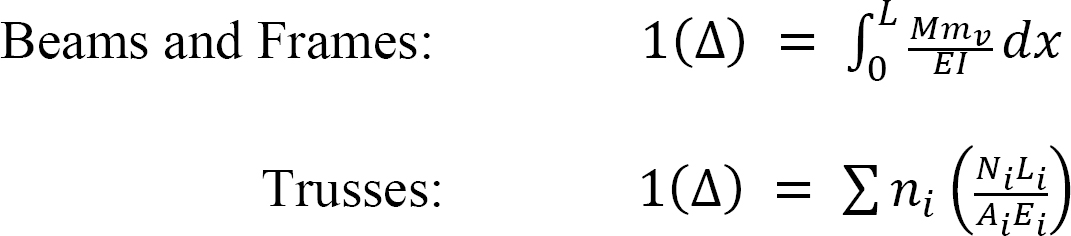
Principle of conservation of energy: The principle of conservation of energy states that the work done by external forces acting on an elastic body in equilibrium are equal to the strain energy stored in the body. This principle can be expressed mathematically, as follows:
W(or Ue) = Ui
The energy method for the determination of deflection is based on Alberto Castigliano’s second theorem. The theorem states that the deflection in a specified direction and at a specified point in a linear elastic structure subjected to a given force is equal to the partial derivative of the total external work or the total internal energy with respect to the applied force. The expressions for the determination of deflection by Castigliano’s second theorem for beams and trusses are as follows:
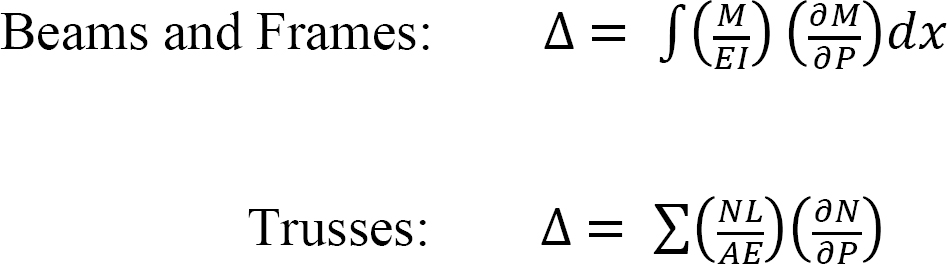
Practice Problems
8.1 Using the virtual work method, determine the slope and deflection at point A of the cantilever beams shown in Figure P8.1 and Figure P8.2.


8.2 Determine the deflection at point D of the beams shown in Figure P8.3 and Figure P8.4.
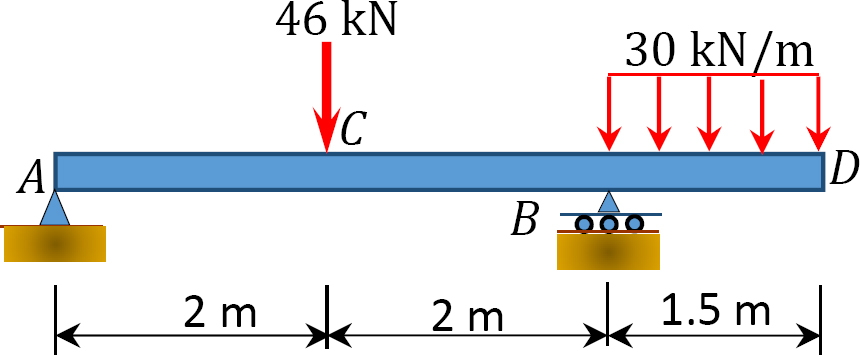

8.3 Using the energy method, determine the slope at support B of the beams shown in Figure P8.5 and Figure P8.6.


8.4 Using the virtual work method, determine the deflection at point H of the trusses shown in Figure P8.7 through Figure P8.10.




8.5 Using the energy method, determine the deflection at point F of the trusses shown in Figure P8.11 and Figure P8.12.
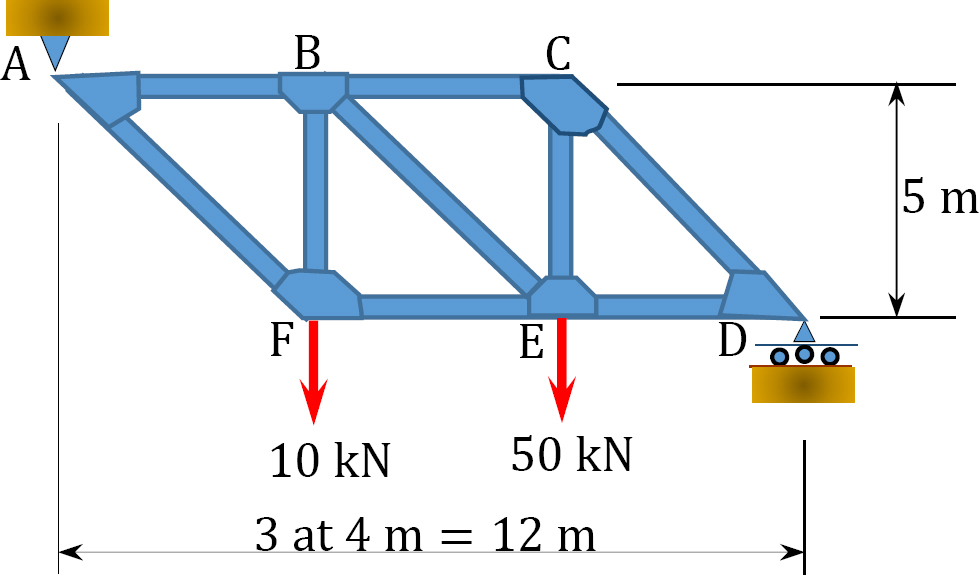

8.6 Using the virtual work method, determine the horizontal deflection at joint C of the trusses shown in Figure P8.13 and Figure P8.14.




