3. Band Gaps
- Page ID
- 5951
Electrons moving in free space have an essentially continuous spectrum of energies; however, when bound to a nucleus in an atom, electrons have discrete energies they can attain (see Atoms and Orbitals), a result of quantum mechanics. Furthermore, when many atoms are brought close together (a solid has something like 1023 atoms in it) in a configuration such as a crystalline semiconductor, these energy levels are smeared out into “bands” that depend on the distance between the atoms in the material1. To be clear, each band contains many energy levels, because the solid contains not only many atoms, but even more electrons. This means that atoms in different materials will have different sets of energy levels, and therefore electrons are allowed to possess a certain spectrum of energies in one material but a different spectrum in another type of material.
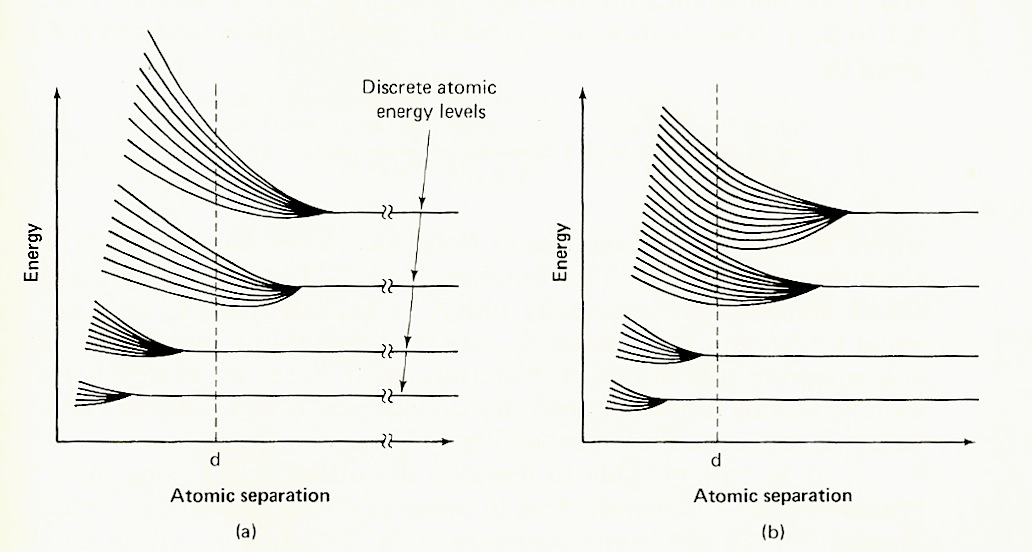
Above (Ref. 1, M.A. Green, UNSW): In the material of figure (a) we see that there is a relatively large gap between the uppermost energy bands, as opposed to (b), where at the atomic separation d the bands overlap and there is no forbidden region between the valence and conduction band.
The two highest bands of energy states of an electron in a material are the valence band and the conduction band. The valence band is the highest range of energy states of an electron that is still bound to an atom; the conduction band is the range of energy states at which the electron is stripped from the atom to flow freely through the atomic lattice of the material. This freedom is what contributes to electric current. The range of forbidden energy levels between the highest level in the valence band and the lowest energy in the conduction band is called the band gap. The material’s band gap is determined by its molecular structure; the periodic, crystalline atomic structure of semiconductors gives their valence electrons the ability to become conductive at certain temperatures.
The main points about band gaps:
- The band gap is the minimum amount of energy required for an electron to break free from its bound state and become conductive (flow freely in the material).
- When an electron becomes conductive, a hole is left behind. This hole flows in the opposite direction of the electron, and also participates in conduction (see Bond Model of a Group IV Semiconductor).
- In a solar context, a photon of the sunlight needs an energy at least that of the bandgap to excite an electron to the conduction band, in other terms to free the electron.
References
1. Green, Martin A. Solar Cells: Operating Principles, Technology, and System Applications. Englewood Cliffs: Prentice-Hall, Inc., 1982. Full book ordering information at www.pv.unsw.edu.au.

