6.6: Off–Axis Loading of a Lamina

Last updated
Save as PDF

Page ID: 8204

\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \) \( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)\(\newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\) \( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\) \( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\) \( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\) \( \newcommand{\Span}{\mathrm{span}}\) \(\newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\) \( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\) \( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\) \( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\) \( \newcommand{\Span}{\mathrm{span}}\)\(\newcommand{\AA}{\unicode[.8,0]{x212B}}\)

Under off-axis loading of a single lamina the applied stress state can be resolved to give the stresses along the laminar principal axes. The stress state of a body can be described by a stress tensor, shown schematically below, which can be related to the strain tensor by the equation:

\[\sigma_{i j}=C_{i j k l} e_{k l}\]

where C_ijkl is a fourth rank stiffness tensor containing 81 components.

Equation 1 can be rearranged to give:

\[e_{i j}=S_{i j k l} \sigma_{k l}\]

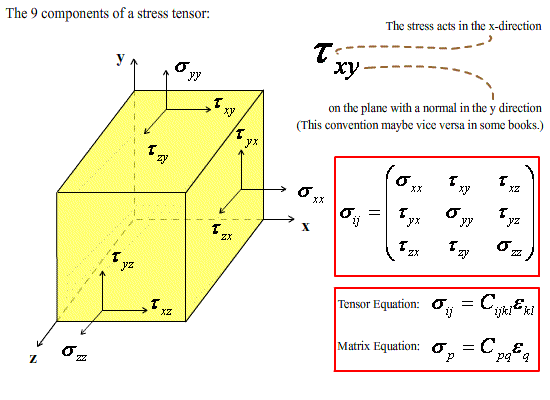
Equation for stress tensor

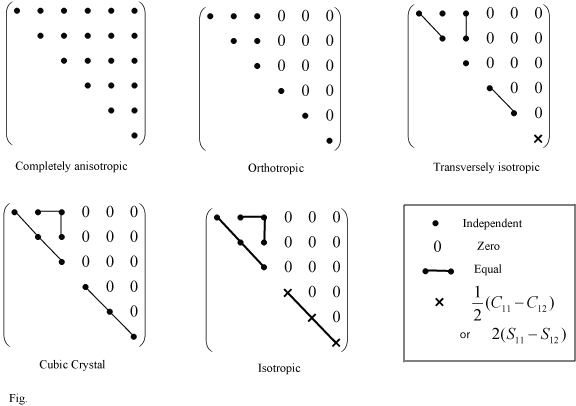
where S_ijkl is the compliance tensor. If the body is in equilibrium both the stress tensor and the stiffness tensor must be symmetric about the diagonal. Then writing Equation 11 as a matrix equation (See Nye 1985) and taking into account the symmetry of the composite itself, the number of independent terms in C_pq reduces to a reasonably small number. A few examples are shown here:

Matrix maths

Resolving Stresses within a Lamina

For a single lamina it is reasonable to assume that all the stresses acting are in the laminar plane, so that σ₃ = τ₂₃ = τ₃₁ = 0. Assuming orthotropic symmetry (likely for a lamina) equation 2 becomes

\[\left[\begin{array}{c}
\varepsilon_{1} \\
\varepsilon_{2} \\
\gamma_{12}
\end{array}\right]=[S]\left[\begin{array}{c}
\sigma_{1} \\
\sigma_{2} \\
\tau_{12}
\end{array}\right]=\left[\begin{array}{ccc}
S_{11} & S_{12} & 0 \\
S_{21} & S_{22} & 0 \\
0 & 0 & S_{66}
\end{array}\right]\left[\begin{array}{c}
\sigma_{1} \\
\sigma_{2} \\
\tau_{12}
\end{array}\right]\]

when stresses are applied along the principal axes of the lamina.

Clearly, when σ₂ = τ₁₂ = 0.

\[S_{11}=\frac{1}{E_1}\]

Similar considerations give

\[S_{22}=\frac{1}{E_{2}}, \quad S_{66}=\frac{1}{G_{12}}, \quad S_{12}=\frac{-\nu_{12}}{E_{1}}=\frac{-\nu_{21}}{E_{2}}\]

where

\[\nu_{21}=\left[f \nu_{f}+(1-f) \nu_{m}\right] \frac{E_{2}}{E_{1}}\]

and

\[v_{12}=\left[f v_{\mathrm{f}}+(1-f) v_{\mathrm{m}}\right]\]

Click here for derivation of Poisson's ratio.

We can now find elastic constants for a lamina whose fibres are at an angle θ to the loading direction by the following resolving procedure:

\[\left[\begin{array}{l}
\varepsilon_{x} \\
\varepsilon_{y} \\
\gamma_{x y}
\end{array}\right]=[\bar S]\left[\begin{array}{l}
\sigma_{x} \\
\sigma_{y} \\
\tau_{x y}
\end{array}\right]\]

The result is that under an arbitrary planar loading system, the transformed compliance tensor replaces the compliance tensor in equation 3. Similar to before,

\[E_{x}=\frac{1}{\bar{S}_{22}}, \quad E_{y}=\frac{1}{\bar{S}_{22}}, \quad G_{x y}=\frac{1}{\bar{S}_{66}}, \quad \nu_{x y}=-E_{x} \bar{S}_{12}, \quad \nu_{y x}=-E_{y} \bar{S}_{12}\]