8.5.2: Osmotic (Salinity Gradient) Power Generation
- Page ID
- 85144
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)Every day over the globe a huge volume of river water flows into oceans and “disappears” – not exactly, but it gets mixed with sea water. Is the process of mixing freshwater with seawater energetically completely neutral? No, it isn’t, the mixing process does release some thermal energy, about 2.2 kJ per liter. But it’s definitely not enough to run any thermal engine.
There is, however, a futuristic concept of capturing that “energy of mix- ing”, using a phenomenon called “osmosis”. It’s a very real phenomenon playing a major role in all living organisms.
Let’s first explain what osmosis is. Suppose that you take an empty rectangular aquarium of an A´B´H volume, where A is the longer side, and H is the height. Inside, in the middle, you install a vertical semipermeable membrane. So now there are two open containers of ½´A´B´H volume each.
The semipermeable membrane is such that it has micro-pores wide enough so that a water molecule can pass through, but too tight for a molecule of salt, NaCl, to pass1. Now, to our divided aquarium, let’s pour: on one side, pure water, to the height of H/2. And to the other half, to the same level, sea water – i.e., 3.5% water solution of table salt, NaCl.
Then wait. And soon a strange thing starts to happen: relative to the original level, the seawater level starts increasing, and the freshwater level – decreasing. Apparently, the water molecules start sneaking through the pores. But note, water molecules are present on both sides of the membrane! They should also pass in the other direction! Perhaps our membrane has one- way micro-holes? Let’s check: let’s empty the aquarium and pour fresh water into the part where the seawater has been, and vice-versa. Well, everything happens as it has happened before. So what’s going on? Why are the water molecules from the freshwater side “privileged”, and those from the seawater side “underprivileged”?
Well, because osmosis plays a crucial role in life processes, and not only for this reason, the phenomenon of osmosis has been thoroughly investigated for about two hundred years – and now our understanding of it, practical as well as theoretical, is really good. However, the “cornerstone” in the theo- retical explanation is the notion of chemical potential. It’s name is perhaps a bit misleading, because it may suggest that it’s something that has an ap- plication only in chemistry. Which is not true! In many areas of physics it has, too!
The chemical potential, commonly denoted as µ, is in certain sense analogous to temperature. Risking a slight simplification, one can say: if one adds/removes heat to/from a given object, the change in its temperature is the measure of how much energy has ben added/removed from it. And for µ, similarly: if you add/remove constituent particles to a given object, the change in its µ is the measure of how much energy has been added/removed from it. Simple? Surely! Yet, in the introductory level academic physics course there is very little time for thermodynamics, so that the chemical potential is not included – in fact, more and more often thermal phenomena are altogether skipped. So, even though the µ-based theory makes it possible to obtain a thorough explanation of all the facts observed in an osmosis process, we cannot present it here.
Instead, we can relay on a conceptual description (the Author hopes that not too many voices of protest). A nice and concise explanation of such kind – one that the Author of this text likes – is presented at the beginning of the Web document posted by Dr. Howard S. Neufeld, a Professor of phys- iological plant ecology at the Appalachian University, who also has strong ties with Corvallis. So, let me quote Dr. Neufeld: When substances are dis- solved in the water, such as salt or sugar, they cause water molecules to lose kinetic energy, because they are attracted to charged sites on these ions and molecules, effectively immobilizing them, and keeping them from evaporating. This lowers the overall energy state of the water.... And then, after a mo- ment, the text continues: Now, if we were to take two containers of water, and separate them by a semi-permeable membrane (one that allows water to go through its pores, but not solutes like salt or sugar), and add sugar to one side, this would result in a lowering of the kinetic energy of the water-sugar solution. Thus, from a statistical-probability point of view, we would expect the molecules of pure water to encounter the membrane more often than the lower energy water molecules on the solution side, and thus, over time, wa- ter will move from the pure water to the solution. Of course some water molecules do go the other way, but the net exchange favors movement into the solution. This is known as osmosis.
Beautiful and simple. This is all we need for understanding the Odsmosis Power idea (but if you are not satisfied with the above explanation and you would like to review the more advanced version based on µ, please continue reading Dr. Neufeld document, there is such version in the page, too!
The Fig. \(\PageIndex{1}\) explains what the osmotic pressure is. When extra pressure is exerted to the solution, the water molecules in it get extra “vigor”, and a process of “reverse osmosis” begins.
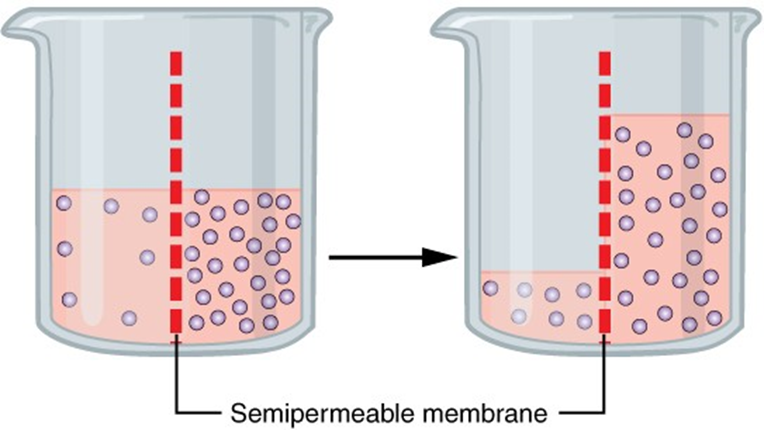
In the experiment shown in Fig. \(\PageIndex{1}\), when the osmosis process proceeds, extra hydrostatic pressure ph = h ρ g builds up, where h is the difference of fluid levels in the two vessels, ρ is the density of the solution, and g is the acceleration due to gravity. The rate of the reverse diffusion is proportional to the pressure ph. At certain moment, the pressure attains such a value that the rates of the forward-osmosis and reverse-osmosis become equal – then the system reaches osmotic equilibrium (source: Wikipedia).
There is a theoretical formula for calculating the osmotic pressure for any combinations of pure solvent and a solution (solvent + solute). The set of parameters needed for calculations is pretty simple: the molecular mass of the solute, the solution concentration (i.e., the mass of the solute per one kg of solvent), and the temperature at which the process occur. The formula has been tested in zillion experiments, so it can be trusted. The formula, as well and a convenient osmotic pressure calculator is provided at HyperPhysics Web Page.
Let’s use the HyperPhysics calculator to find the osmotic pressure for a pure water - sea water combination. The concentration of salt in seawater is about \(3.5 \%\), so in the boxes "solute mass" and the "solution volume" we put, respectively, 35 grams and \(1000 \mathrm{~cm}^{3}\). Next, the calculator needs the molecular weight of the solute, so we add the atomic weights of \(\mathrm{Na}\) (22.99) and \(\mathrm{Cl}(35.45)\), so we type in \(58.44\). And finally, it needs the temperature in absolute scale - so let put in \(293 \mathrm{~K}\), which is a pleasant room temperature. And we get a prompt answer from the calculator, \(1459 \mathrm{kPa}\).
Well, kiloPacal means \(1000 \mathrm{~N} / \mathrm{m}^{2}\), but it is not a very popular pressure unit. In America everybody knows what is a pound per square inch pressure, but a kPa? \(1459 \mathrm{kPa}\) may not look unusual until we do some checking. OK, then let's check what's the pressure exerted by a 1 meter column of seawater. \(p=h \times \rho \times g\), right? For seawater \(\rho=1025 \mathrm{~kg} / \mathrm{m}^{3}\), so:
\[ p=1 \mathrm{~m} \times 1025 \mathrm{~kg} / \mathrm{m}^{3} \times 9.81 \mathrm{~m} / \mathrm{s}^{2}=10055\left(\mathrm{~kg} \cdot \mathrm{m} / \mathrm{s}^{2}\right) / \mathrm{m}^{2} \]
\[=10055 \mathrm{~N} / \mathrm{m}^{2}=10055 \mathrm{~Pa}=10.055 \mathrm{kPa} \]
Only now the osmotic pressure provided by the calculator becomes really impressive: it turns out it's equal to the hydrostatic pressure of a column of seawater 145 meters high, almost one-half of the height of the famous Eiffel Tower in Paris! Or, one can say, the osmotic pressure of seawater in apure water-seawater combination is \(14.4\) times the atmospheric pressure.
Such a very high water column could never be obtained in the apparatus shown in the Fig. 8.22. Why? Because when the osmosis goes on, more and more pure water enters the seawater compartment, gradually diluting the seawater. And the osmotic pressure is proportional to the solute concentration in the solvent. Therefore, even if the "neck" of the bottle-like vessel in the Fig. \(8.22\) were much longer, the column height would stop increasing after reaching a much smaller value than \(h=145 \mathrm{~m}\).
But you don't need columns as high as \(145 \mathrm{~m}\) to operate water turbines! Much lower \(h\) values could also do. And this is exactly the idea underlying the osmotic power generation, or salinity gradient power generation method. Use water river as the "pure water", and seawater as the "solution" - and by osmosis, raise the level of the fluid in the seawater compartment high enough so that the hydrostatic pressure which builds up is sufficient to drive a turbine.
In a Web article a team of Norwegian researchers presents a thorough explanation of how osmoticenergy production can beimplemented in practice. Diagrams of such power plants are shown and the issue of manufacturing semi-permeable warranting a high efficiency of the process membranes is dis- cussed in detail.
The method discussed in the Norwegian paper is not the only one possible – more sophisticated methods have been conceived. More details about such ideas can be found in this Web document, in which there is also a link to a short YouTube video explaining the principles of the osmotic power generation method. Other instructive articles can be found in Wikipedia, in Sciencedirect.com, or in this report from the Science Advances journal.
According to the data discussed in the Web documents linked, the world resources of osmotic power generation are such that they may satisfy more that 10% of the current global electric power consumption. It’s therefore a bit disappointing that until now only installations with sub-kiloWatt power has been tested. Nothing has yet emerged over the level of “early R&D projects”. The relatively low prices of fossil fuels are probably a “strong slowing-down factor” for such projects. But it’s important to continue the R&D work, because – who knows? – such times may come that the world will need to quickly activate all existing reserves.
___________________________________________
1. Many such membranes are available. In the old days, researchers used membranes taken from slaughtered animals. Fortunately, now we can make synthetic semipermeable membranes using different methods. For instance, by irradiating thin plastic sheets by beams of heavy high-energy ions, which punch “microholes” of desired width. Such mem- branes are widely used, e.g., in the apparatus for artificial dialysis – they save life of people whose kidneys do not function normally. Or, in the Big 5 store in Corvallis for about 20 bucks you may buy a “magic straw” for backpackers: one may drink water from very con- taminated creeks or waterholes, and 99.99% of germs have no chance of sneaking through the micropores in the straw.

