5.4: Electric Field Due to a Continuous Distribution of Charge
- Page ID
- 3926
The electric field intensity associated with \(N\) charged particles is (Section 5.2):
\[{\bf E}({\bf r}) = \frac{1}{4\pi\epsilon} \sum_{n=1}^{N} { \frac{{\bf r}-{\bf r}_n}{\left|{\bf r}-{\bf r}_n\right|^3}~q_n} \label{m0104_eCountable} \]
where \(q_n\) and \({\bf r}_n\) are the charge and position of the \(n^{\mbox{th}}\) particle. However, it is common to have a continuous distribution of charge as opposed to a countable number of charged particles. In this section, we extend Equation \ref{m0104_eCountable} using the concept of continuous distribution of charge (Section 5.3) so that we may address this more general class of problems.
Distribution of Charge Along a Curve
Consider a continuous distribution of charge along a curve \(\mathcal{C}\). The curve can be divided into short segments of length \(\Delta l\). Then, the charge associated with the \(n^{\mbox{th}}\) segment, located at \({\bf r}_n\), is
\[q_n = \rho_l({\bf r}_n)~\Delta l \nonumber \]
where \(\rho_l\) is charge density (units of C/m) at \({\bf r}_n\). Substituting this expression into Equation \ref{m0104_eCountable}, we obtain
\[{\bf E}({\bf r}) = \frac{1}{4\pi\epsilon} \sum_{n=1}^{N} { \frac{{\bf r}-{\bf r}_n}{\left|{\bf r}-{\bf r}_n\right|^3}~\rho_l({\bf r}_n)~\Delta l} \nonumber \]
Taking the limit as \(\Delta l\to 0\) yields:
\[\boxed{ {\bf E}({\bf r}) = \frac{1}{4\pi\epsilon} \int_{\mathcal C} { \frac{{\bf r}-{\bf r}'}{\left|{\bf r}-{\bf r}'\right|^3}~\rho_l({\bf r}')~dl} } \label{m0104_eLineCharge} \]
here \({\bf r}'\) represents the varying position along \({\mathcal C}\) with integration.
The simplest example of a curve is a straight line. It is straightforward to use Equation \ref{m0104_eLineCharge} to determine the electric field due to a distribution of charge along a straight line. However, it is much easier to analyze that particular distribution using Gauss’ Law, as shown in Section 5.6. The following example addresses a charge distribution for which Equation \ref{m0104_eLineCharge} is more appropriate.
Consider a ring of radius \(a\) in the \(z=0\) plane, centered on the origin, as shown in Figure \(\PageIndex{1}\). Let the charge density along this ring be uniform and equal to \(\rho_l\) (C/m). Find the electric field along the \(z\) axis.
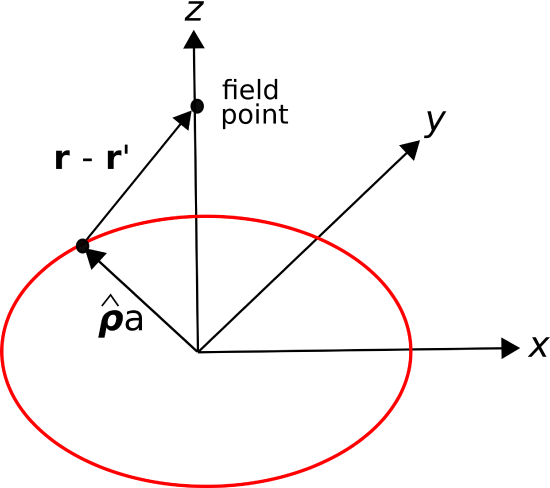
Solution
The source charge position is given in cylindrical coordinates as
\[{\bf r}' = \hat{\bf \rho}a \nonumber \]
The position of a field point along the \(z\) axis is simply
\[{\bf r} = \hat{\bf z}z \nonumber \]
Thus,
\[{\bf r}-{\bf r}' = -\hat{\bf \rho}a + \hat{\bf z}z \nonumber \]
and
\[\left|{\bf r}-{\bf r}'\right| = \sqrt{a^2+z^2} \nonumber \]
Equation \ref{m0104_eLineCharge} becomes:
\[{\bf E}(z) = \frac{1}{4\pi\epsilon} \int_{0}^{2\pi} { \frac{-\hat{\bf \rho}a + \hat{\bf z}z}{\left[a^2+z^2\right]^{3/2}}~\rho_l~\left(a~d\phi\right)} \nonumber \]
Pulling factors that do not vary with \(\phi\) out of the integral and factoring into separate integrals for the \(\hat{\bf \phi}\) and \(\hat{\bf z}\) components, we obtain:
\[\frac{\rho_l~a}{4\pi\epsilon\left[a^2+z^2\right]^{3/2}} \left[ -a\int_{0}^{2\pi}{\hat{\bf \rho}~d\phi} +\hat{\bf z}z\int_{0}^{2\pi} {d\phi} \right] \nonumber \]
The second integral is equal to \(2\pi\). The first integral is equal to zero. To see this, note that the integral is simply summing values of \(\hat{\bf\rho}\) for all possible values of \(\phi\). Since \(\hat{\bf\rho}(\phi+\pi)=-\hat{\bf\rho}(\phi)\), the integrand for any given value of \(\phi\) is equal and opposite the integrand \(\pi\) radians later. (This is one example of a symmetry argument.) Thus, we obtain
\[{\bf E}(z) = \hat{\bf z}\frac{\rho_l~a}{2\epsilon}\frac{z}{\left[a^2+z^2\right]^{3/2}} \nonumber \]
It is a good exercise to confirm that this result is dimensionally correct. It is also recommended to confirm that when \(z\gg a\), the result is approximately the same as that expected from a particle having the same total charge as the ring.
Distribution of Charge Over a Surface
Consider a continuous distribution of charge over a surface \(\mathcal{S}\). The surface can be divided into small patches having area \(\Delta s\). Then, the charge associated with the \(n^{\mbox{th}}\) patch, located at \({\bf r}_n\), is
\[q_n = \rho_s({\bf r}_n)~\Delta s \nonumber \]
where \(\rho_s\) is the surface charge density (units of C/m\(^2\)) at \({\bf r}_n\). Substituting this expression into Equation \ref{m0104_eCountable}, we obtain
\[{\bf E}({\bf r}) = \frac{1}{4\pi\epsilon} \sum_{n=1}^{N} { \frac{{\bf r}-{\bf r}_n}{\left|{\bf r}-{\bf r}_n\right|^3}~\rho_s({\bf r}_n)~\Delta s} \nonumber \]
Taking the limit as \(\Delta s\to 0\) yields:
\[\boxed{ {\bf E}({\bf r}) = \frac{1}{4\pi\epsilon} \int_{\mathcal S} { \frac{{\bf r}-{\bf r}'}{\left|{\bf r}-{\bf r}'\right|^3}~\rho_s({\bf r}')~ds} } \label{m0104_eSurfCharge} \]
where \({\bf r}'\) represents the varying position over \({\mathcal S}\) with integration.
Consider a circular disk of radius \(a\) in the \(z=0\) plane, centered on the origin, as shown in Figure \(\PageIndex{2}\). Let the charge density over this disk be uniform and equal to \(\rho_s\) (C/m\(^2\)). Find the electric field along the \(z\) axis.
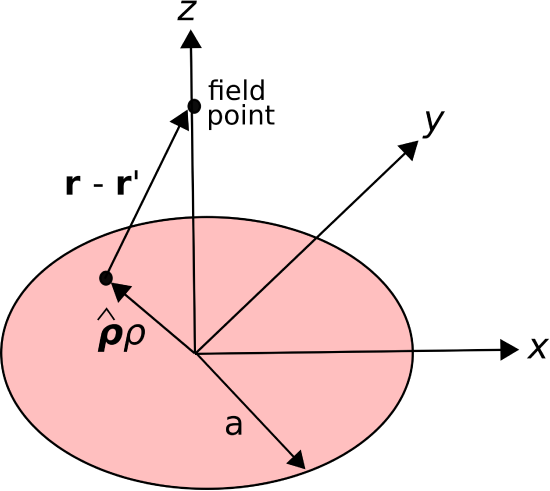
Solution
The source charge position is given in cylindrical coordinates as
\[{\bf r}' = \hat{\bf \rho}\rho \nonumber \]
The position of a field point along the \(z\) axis is simply
\[{\bf r} = \hat{\bf z}z \nonumber \] Thus, \[{\bf r}-{\bf r}' = -\hat{\bf \rho}\rho + \hat{\bf z}z \nonumber \]
and
\[\left|{\bf r}-{\bf r}'\right| = \sqrt{\rho^2+z^2} \nonumber \]
Equation \ref{m0104_eSurfCharge} becomes:
\[{\bf E}(z) = \frac{1}{4\pi\epsilon} \int_{\rho=0}^{a} \int_{\phi=0}^{2\pi} { \frac{-\hat{\bf \rho}\rho + \hat{\bf z}z}{\left[\rho^2+z^2\right]^{3/2}}~\rho_s~\left(\rho~d\rho~d\phi\right)} \nonumber \]
To solve this integral, first rearrange the double integral into a single integral over \(\phi\) followed by integration over \(\rho\):
\[\frac{\rho_s}{4\pi\epsilon} \int_{\rho=0}^{a} { \frac{\rho}{\left[\rho^2+z^2\right]^{3/2}} \left[ \int_{\phi=0}^{2\pi} { \left(-\hat{\bf \rho}\rho + \hat{\bf z}z \right) d\phi } \right] d\rho } \label{m0104_eDisk1} \]
Now we address the integration over \(\phi\) shown in the square brackets in the above expression:
\[\int_{\phi=0}^{2\pi} { \left(-\hat{\bf \rho}\rho + \hat{\bf z}z \right) d\phi } = -\rho\int_{\phi=0}^{2\pi} { \hat{\bf \rho} d\phi } + \hat{\bf z}z\int_{\phi=0}^{2\pi} { d\phi } \nonumber \]
The first integral on the right is zero for the following reason. As the integral progresses in \(\phi\), the vector \(\hat{\bf \rho}\) rotates. Because the integration is over a complete revolution (i.e., \(\phi\) from 0 to \(2\pi\)), the contribution from each pointing of \(\hat{\bf \rho}\) is canceled out by another pointing of \(\hat{\bf \rho}\) that is in the opposite direction. Since there is an equal number of these canceling pairs of pointings, the result is zero. Thus:
\[\int_{\phi=0}^{2 \pi}(-\hat{\rho} \rho+\hat{\mathbf{z}} z) d \phi =0+\hat{\mathbf{z}} z \int_{\phi=0}^{2 \pi} d \phi = \hat{\mathbf{z}} 2 \pi z \nonumber \]
Substituting this into Expression \ref{m0104_eDisk1} we obtain:
\begin{align*}
& \frac{\rho_{s}}{4 \pi \epsilon} \int_{\rho=0}^{a} \frac{\rho}{\left[\rho^{2}+z^{2}\right]^{3 / 2}}[\hat{\mathbf{z}} 2 \pi z] d \rho \\
=& \hat{\mathbf{z}} \frac{\rho_{s} z}{2 \epsilon} \int_{\rho=0}^{a} \frac{\rho d \rho}{\left[\rho^{2}+z^{2}\right]^{3 / 2}}
\end{align*}
This integral can be solved using integration by parts and trigonometric substitution. Since the solution is tedious and there is no particular principle of electromagnetics demonstrated by this solution, we shall simply state the result:
\begin{align*}
\int_{\rho=0}^{a} \frac{\rho d \rho}{\left[\rho^{2}+z^{2}\right]^{3 / 2}} &=\left.\frac{-1}{\sqrt{\rho^{2}+z^{2}}}\right|_{\rho=0} ^{a} \\
&=\frac{-1}{\sqrt{a^{2}+z^{2}}}+\frac{1}{|z|}
\end{align*}
Substituting this result:
\begin{align*}
\mathbf{E}(z) &=\hat{\mathbf{z}} \frac{\rho_{s} z}{2 \epsilon}\left(\frac{-1}{\sqrt{a^{2}+z^{2}}}+\frac{1}{|z|}\right) \\
&=\hat{\mathbf{z}} \frac{\rho_{s}}{2 \epsilon}\left(\frac{-z}{\sqrt{a^{2}+z^{2}}}+\frac{z}{|z|}\right) \\
&=\hat{\mathbf{z}} \frac{\rho_{s}}{2 \epsilon}\left(\frac{-z}{\sqrt{a^{2}+z^{2}}}+\operatorname{sgn} z\right)
\end{align*}
where “sgn” is the “signum” function; i.e., \(\mbox{sgn}~z =+1\) for \(z>0\) and \(\mbox{sgn}~z =-1\) for \(z<0\).
Summarizing
\[{\bf E}(z) = \hat{\bf z}\frac{\rho_s }{2\epsilon} \left( \mbox{sgn}~z - \frac{z}{\sqrt{a^2+z^2}} \right) \label{m0104_eDisk2} \]
It is a good exercise to confirm that this result is dimensionally correct and yields an electric field vector that points in the expected direction and with the expected dependence on \(a\) and \(z\).
A special case of the “disk of charge” scenario considered in the preceding example is an infinite sheet of charge. The electric field from an infinite sheet of charge is a useful theoretical result. We get the field in this case simply by letting \(a\to\infty\) in Equation \ref{m0104_eDisk2}, yielding:
\[{\bf E}({\bf r}) = \hat{\bf z}\frac{\rho_s}{2\epsilon} \mbox{sgn}~z \label{m0104_eISC} \]
Again, it is useful to confirm that this is dimensionally correct: C/m\(^2\) divided by F/m yields V/m. Also, note that Equation \ref{m0104_eISC} is the electric field at any point above or below the charge sheet – not just on \(z\) axis. This follows from symmetry. From the perspective of any point in space, the edges of the sheet are the same distance (i.e., infinitely far) away.
Distribution of Charge in a Volume
Consider a continuous distribution of charge within a volume \(\mathcal{V}\). The volume can be divided into small cells (volume elements) having volume \(\Delta v\). Then, the charge associated with the \(n^{\mbox{th}}\) cell, located at \({\bf r}_n\), is \[q_n = \rho_v({\bf r}_n)~\Delta v \nonumber \] where \(\rho_v\) is volume charge density (units of C/m\(^3\)) at \({\bf r}_n\). Substituting this expression into Equation \ref{m0104_eCountable}, we obtain
\[{\bf E}({\bf r}) = \frac{1}{4\pi\epsilon} \sum_{n=1}^{N} { \frac{{\bf r}-{\bf r}_n}{\left|{\bf r}-{\bf r}_n\right|^3}~\rho_v({\bf r}_n)~\Delta v} \nonumber \]
Taking the limit as \(\Delta v\to 0\) yields:
\[\boxed{ {\bf E}({\bf r}) = \frac{1}{4\pi\epsilon} \int_{\mathcal V} { \frac{{\bf r}-{\bf r}'}{\left|{\bf r}-{\bf r}'\right|^3}~\rho_v({\bf r}')~dv} } \nonumber \]
where \({\bf r}'\) represents the varying position over \({\mathcal V}\) with integration.


