1.2: Pulse Characteristics

Last updated
Save as PDF

Page ID: 44639

Franz X. Kaertner
Massachusetts Institute of Technology via MIT OpenCourseWare

\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \) \( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)\(\newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\) \( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\) \( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\) \( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\) \( \newcommand{\Span}{\mathrm{span}}\) \(\newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\) \( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\) \( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\) \( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\) \( \newcommand{\Span}{\mathrm{span}}\)\(\newcommand{\AA}{\unicode[.8,0]{x212B}}\)

Most often, there is not an isolated pulse, but rather a pulse train.

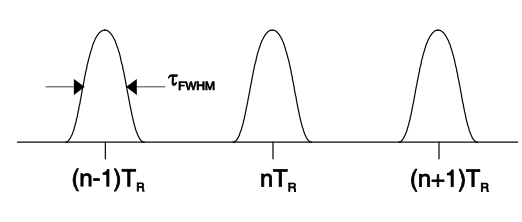
截屏2021-03-24 下午7.24.41.png — Figure 1.1: Periodic pulse train

\(T_R\): pulse repetition time
\(W\) : pulse energy
\(P_{ave} = W/T_R\): average power
\(\tau{\text{FWHM}}\) is the Full Width at Half Maximum of the intensity envelope of the pulse in the time domain.
The peak power is given by

\[P_p = \dfrac{W}{\tau{\text{FWHM}}} = P_{ave} \dfrac{T_R}{\tau{\text{FWHM}}} \nonumber \]

and the peak electric field is given by

\[E_p = \sqrt{2 Z_{F_0} \dfrac{P_p}{A_{\text{eff}}} \nonumber \]

\(A_{\text{eff}}\) is the beam cross-section and \(Z_{F_0} = 377 \Omega\) is the free space impedance.

Time scales:

\[\begin{array} {lcl} {\text{1 ns}} & \sim & {30\text{ cm (high-speed electronics, GHz}} \\ {\text{1 ps}} & \sim & {300\ \mu\text{m}} \\ {\text{1 fs}} & \sim & {\text{300 nm}} \\ {1 \text{ as} = 10^{-18} s} & \sim & {\text{0.3 nm = 3} \mathring{A} \text{ (typ-lattice constant in metal)}} \end{array} \nonumber \]

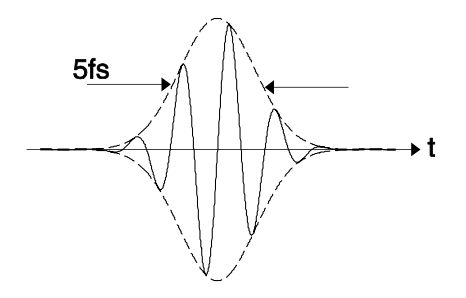
The shortest pulses generated to date are about 4 - 5 fs at 800 nm \((\lambda/c = 2.7\) fs), less than two optical cycles and 250 as at 25 nm. For few-cycle pulses, the electric field becomes important, not only the intensity!

截屏2021-03-24 下午7.41.37.png — Figure 1.2: Electric field waveform of a 5 fs pulse at a center wavelength of 800 nm. The electric field depends on the carrier-envelope phase.

average power:

\[\begin{array} {cl} {P_{ave} \sim} & {\text{1W, up to 100 W in progress.}} \\ {\ } & {\text{kW possible, not yet pulsed}} \end{array} \nonumber \]

repetition rates:

\[T_R^{-1} = f_R = \text{m Hz - 100 GHz}\nonumber \]

pulse energy:

\[W = 1pJ - 1kJ\nonumber \]

pulse width:

\[\tau_{\text{FWHM}} = \begin{array} {ll} {\text{5 fs - 50 ps,}} & {\text{modelocked}} \\ {\text{30 ps - 100 ns,}} & {\text{Q - switched}} \end{array}\nonumber \]

peak power:

\[P_p = \dfrac{\text{1 kJ}}{\text{1 ps}} \sim \text{1 PW},\nonumber \]

obtained with Nd:glass (LLNL - USA, [1][2][3]).

For a typical lab pulse, the peak power is

\[P_p = \dfrac{\text{10 nJ}}{\text{10 fs}} \sim \text{1 MW}\nonumber \]

peak field of typical lab pulse:

\[E_p = \sqrt{2 \times 377 \times \dfrac{10^6 \times 10^{12}}{\pi \times (1.5)^2}} \dfrac{\text{V}}{\text{m}} \approx 10^{10} \dfrac{\text{V}}{\text{m}} = \dfrac{10\text{V}}{\text{nm}}\nonumber \]