6.4: Dispersion Managed Soliton Formation
- Page ID
- 52403
The nonlinear Schrödinger equation describes pulse propagation in a medium with continuously distributed dispersion and self-phase-modulation. For lasers generating pulses as short as 10 fs and below, it was first pointed out by Spielmann et al. that large changes in the pulse occur within one roundtrip and that the ordering of the pulse-shaping elements within the cavity has a major effect on the pulse formation [9]. The discrete action of linear disper- sion in the arms of the laser resonator and the discrete, but simultaneous, action of positive SPM and positive GDD in the laser crystal cannot any longer be neglected. The importance of strong dispersion variations for the laser dynamics was first discovered in a fiber laser and called stretched pulse modelocking [11]. The positive dispersion in the Er-doped fiber section of a fiber ring laser was balanced by a negative dispersive passive fiber. The pulse circulating in the ring was stretched and compressed by as much as a factor of 20 in one roundtrip. One consequence of this behavior was a dramatic decrease of the nonlinearity and thus increased stability against the SPM induced instabilities. The sidebands, due to periodic perturbations of the soliton, as discussed in section 3.6, are no longer observed (see Figure 6.12).
Image removed due to copyright restrictions.
Please see:
Tamura, K., E. P. Ippen, H. A. Haus, and L. E. Nelson. "77-fs pulse generation from a stretched-pulse mode-locked all-fiber ring laser." Optics Letters 18 (1993): 1080-1082.
Figure 6.12: Spectra of mode-locked Er-doped fiber lasers operating in the conventional soliton regime, i.e. net negative dispersion and in the stretched pulse mode of operation at almost zero average dispersion [11].
The energy of the output pulses could be increased 100 fold. The minimum pulsewidth was 63 fs, with a bandwdith much broader than the erbium gain bandwidth [12]. Figure 6.12 also shows the spectral enhancement of the fiber laser in the dispersion managed regime. The generation of ultrashort pulses from solid state lasers like Ti:sapphire has progressed over the past decade and led to the generation of pulses as short as 5 fs directly from the laser. At such short pulse lengths the pulse is streched up to a factor of ten when propagating through the laser crystal creating a dispersion managed soliton [10]. The spectra generated with these lasers are not of simple shape for many reasons. Here, we want to consider the impact on the spectral shape and laser dynamcis due to dispersion managed soliton formation.
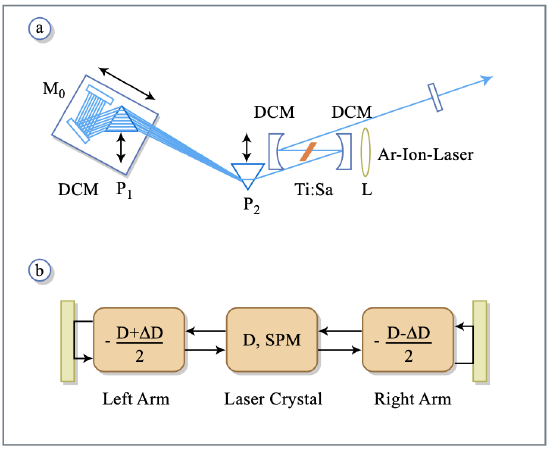
Figure by MIT OCW.
A mode-locked laser producing ultrashort pulses consists at least of a gain prism pairs), see Figure 6.13 a. The system can be decomposed into the res- onator arms and the crystal, see Figure 6.13 b. To achieve ultrashort pulses, the dispersion-balancing components should produce near-zero net dispersion while the dispersion element(s) individually produce significant group delay over the broad bandwidth of the laser pulse. This fact suggests an analogy with dispersion-managed pulse propagation along a dispersion-managed fiber transmission link [14]. A system with sufficient variation of dispersion can support solitary waves. One can show that the Kerr nonlinearity produces a self-consistent nonlinear scattering potential that permits formation of a perodic solution with a simple phase factor in a system with zero net dispersion. The pulses are analogous to solitons in that they are self-consistent solutions of the Hamiltonian (lossless) problem as the conventional solitons discussed above. But they are not secant hyperbolic in shape. Figure 6.14 shows a numerical simulation of a self-consistent solution of the Hamiltonian pulse-propagation problem in a linear medium of negative dispersion and subsequent propagation in a nonlinear medium of positive dispersion and positive self-phase modulation, following the equation
\[\dfrac{\partial}{\partial z} A(z, t) = j D(z) \dfrac{\partial^2}{\partial t^2} A(z, t) - j \delta (z) |A|^2 A(z, t)\label{eq6.4.1} \]
Image removed due to copyright restrictions. Please see:
Chen, Y., et al. "Dispersion managed mode-locking." Journal of Optical Society of Americas B 16 (1999): 1999-2004.
Figure 6.14: Pulse shaping in one round trip. The negative segment has no nonlinearity.
In Figure 6.15 the steady state intensity profiles are shown at the center of the negative dispersion segment over 1000 roundtrips. It is clear that the solution repeats itself from period to period, i.e. there is a new solitary wave that solves the piecewise nonlinear Schroedinger equation 6.65, dispersion managed soliton. In contrast to the conventional soliton the dispersion mangaged soliton of equation 6.65 (with no SAM and no filtering) resemble Gaussian pulses down to about -10 dB from the peak, but then show rather complicated structure, see Figure 6.15.The dispersion map \(D(z)\) used is shown as an inset in Figure 6.14. One can additionally include saturable gain, Lorentzian gain filtering, and a fast saturable absorber. Figure 6.14 shows the behavior in one period (one round trip through the resonator) including these effects. The response of the absorber is \(q(A) = q_o/(1+|A|^2/P_A)\), with \(q_o = 0.01\)/mm and \(P_A = 1\) MW. The bandwidth-limited gain is modeled by the Lorentzian profile with gain bandwidth \(2\pi \times 43\) THz. The filtering and saturable absorption reduce the spectral and temporal side lobes of the Hamiltonian problem. As can be inferred from Figure 6.14, the steady state pulse formation can be understood in the following way. By symmetry the pulses are chirp free in the middle of the dispersion cells. A chirp free pulse starting in the center of the gain crystal, i.e. nonlinear segment is spectrally broadened by the SPM and disperses in time due to the GVD, which generates a rather linear chirp over the pulse. After the pulse is leaving the crystal it experiences negative GVD during propagation through the left or right resonator arm, which is compressing the positively chirped pulse to its transform limit at the end of the arm, where an output coupler can be placed. Back propagation towards the crystal imposes a negative chirp, generating the time reversed solution of the nonliner Schrödinger equation (\(\ref{eq6.4.1}\)). Therefore, subsequent propagation in the nonlinear crystal is compressing the pulse spectrally and temporally to its initial shape in the center of the crystal. The spectrum is narrower in the crystal than in the negative-dispersion sections, because it is negatively prechirped before it enters the SPM section and spectral spreading occurs again only after the pulse has been compressed. This result further explains that in a laser with a linear cavity, for which the negative dispersion is located in only one arm of the laser resonator (i.e. in the prism pair and no use of chirped mirrors) the spectrum is widest in the arm that contains the negative dispersion . In a laser with a linear cavity, for which the negative dispersion is equally distributed in both arms of the cavity, the pulse runs through the dispersion map twice per roundtrip. The pulse is short at each end of the cavity and, most importantly, the pulses are identical in each pass through the crystal, which exploits the saturable absorber action (Kerr-Lens Modelocking in this case, as will be discussed in the next chapter) twice per roundtrip, in contrast to an asymmetric dispersion distribution in the resonator arms. Thus a symmetric dispersion distribution leads to an effective saturable absorption that is twice as strong as an asymmetric dispersion distribution resulting in substantially shorter pulses. Furthermore, the dispersion swing between the negative and positive dispersion sections is only half, which allows for shorter dispersion-managed solitons operating at the same average power level.
Image removed due to copyright restrictions. Please see:
Chen, Y., et al. "Dispersion managed mode-locking." Journal of Optical Society of Americas B 16 (1999): 1999-2004.
Figure 6.15: Simulation of the Hamiltonian problem. Intensity profiles at the center of the negatively dispersive segment are shown for successive roundtrips. The total extent in 1000 roundtrips. \(D = D^{(\pm)} = \pm 60\ fs^2/mm\), segment of crystal length \(L = 2\) mm, \(\tau_{FWHM} = 5.5\) fs, \(\delta = 0\) for \(D < 0\), \(\delta = 1\ (MW\ mm)^{-1}\) for \(D > 0\).[10]
To further illustrate the efficiency of the dispersion managed soliton formation, we present a series of simulations that start with a linear segment of negative dispersion and a nonlinear segment of positive dispersion of the same magnitude, saturable absorber action, and filtering.
Image removed due to copyright restrictions. Please see:
Chen, Y., et al. "Dispersion managed mode-locking." Journal of Optical Society of Americas B 16 (1999): 1999-2004.
Figure 6.16: Sequence of pulse profiles in the center of the negatively dis- persive segment for three magnitudes of SPM. \(t_o = 3\ fs\), with solid curves (5.5 fs) for \(\delta = 1\ (MW\ mm)^{-1}\), dashed-dotted curve (7 fs) for \(\delta = 0.5 \ (MW\ mm)^{-1}\), and dashed cuves for no SPM of \(\delta = 0\). The dispersion map is of Figure 6.14. The output coupler loss is 3%. [10]
The dashed curve in Figure 6.16 shows the pulse shape for gain, loss, saturable absorption and gain filtering only. We obtained the other traces by increasing the SPM while keeping the energy fixed through adjustment of the gain. As one can see, increasing the SPM permits shorter pulses. The shortest pulse can be approximately three times shorter than the pulse without SPM. The parameters chosen for the simulations are listed in the figure caption. In this respect, the behavior is similar to the fast saturable absorber case with conventional soliton formation as discussed in the last section.
A major difference in the dispersion managed soliton case is illustrated in Figure 6.17. The figure shows the parameter ranges for a dispersion-managed soliton system (no gain, no loss, no filtering) that is unbalanced such as to result in the net dispersion that serves as the abscissa of the figure. Each curve gives the locus of energy versus net cavity dispersion for a stretching ratio \(S = LD/\tau_{FWHM}^2\) (or pulse width with fixed crystal length \(L\)). One can see that for pulse width longer than 8 fs with crystal length \(L = 2\ mm\), no solution of finite energy exists in the dispersion managed system for zero or positive net dispersion. Pulses of durations longer than 8 fs require net negative dispersion. Hence one can reach the ultrashort dispersion managed soliton operation at zero net dispersion only by first providing the system with negative dispersion. At the same energy, one can form a shorter pulse by reducing the net dispersion, provided that the 8 fs threshold has been passed. For a fixed dispersion swing \(\pm D\), the stretching increases quadratically with the spectral width or the inverse pulse width. Long pulses with no stretching have a sech shape. For stretching ratios of 3-10 the pulses are Gaussian shaped. For even larger stretching ratios the pulse spectra become increasingly more flat topped, as shown in Figure 6.16.
Image removed due to copyright restrictions. Please see:
Chen, Y., et al. "Dispersion managed mode-locking." Journal of Optical Society of Americas B 16 (1999): 1999-2004.
Figure 6.17: Energy of the pulse in the lossless dispersion-managed system with stretching \(S = LD/\tau_{FWHM}^2\) or for a fixed crystal length \(L\) and pulsewidth as parameters; \(D = 60\ fs^2/mm\) for Ti:sapphire at 800 nm [10].
To gain insight into the laser dynamics and later on in their noise and tuning behavior, it is advantageous to formulate also a master equation approach for the dispersion managed soliton case [16]. Care has to be taken of the fact that the Kerr-phase shift is produced by a pulse of varying amplitude and width as it circulates around the ring. The Kerr-phase shift for a pulse of constant width, \(\delta |a|^2\) had to be replaced by a phase profile that mimics the average shape of the pulse, weighted by its intensity. Therefore, the SPM action is replaced by
\[\delta |A|^2 = \delta_o |A_o|^2 \left ( 1 - \mu \dfrac{t^2}{\tau^2} \right ) \nonumber \]
where \(A_o\) is the pulse amplitude at the position of minimum width. The Kerr-phase profile is expanded to second order in \(t\). The coefficient \(\delta_o\) and \(\mu\) are evaluated variationally. The saturable absorber action is similarly expanded. Finally, the net intracavity dispersion acting on average on the pulse is replaced by the effective dispersion \(D_{\text{net}}\) in the resonator within one roundtrip. The master equation becomes
\[T_R \dfrac{\partial}{\partial T} A = (g - l) A + \left (\dfrac{1}{\Omega_f^2} + j D_{\text{net}} \right ) \dfrac{\partial^2}{\partial t^2} A + (\gamma_o - j \delta_o) |A_o|^2 \left (1 - \mu \tfrac{t^2}{\tau^2} \right ) A\label{eq6.4.3} \]
This equation has Gaussian-pulse solutions. The master equation (\(\ref{eq6.4.3}\)) is a patchwork, it is not an ordinary differential equation. The coefficients in the equation depend on the pulse solution and eventually have to be found iteratively. Nevertheless, the equation accounts for the pulse shaping in the system in an analytic fashion. It will allow us to extend the conventional soliton perturbation theory to the case of dispersion managed solitons.
There is one more interesting property of the stretched pulse operation that needs to be emphasized. Dispersion managed solitons may form even when the net dispersion as seen by a linearly propagating pulse is zero or slightly positive. This is a surprising result which was discovered in the study of dispersion managed soliton propagation [14]. It turns out that the stretched pulse changes its spectrum during propagation through the two segments of fiber with opposite dispersion or in the case of a Ti:Sapphire laser in the nonlinear crystal. The spectrum in the segment with normal (positive) dispersion is narrower, than in the segment of anomalous (nega- tive) dispersion, see Figure 6.14. The pulse sees an effective net negative dispersion, provided that the positive \(D_{\text{net}}\) is not too large. In (\(\ref{eq6.4.3}\)) the \(D_{\text{net}}\) is to be replaced by \(D_{\text{eff}}\) which can be computed variationally. Thus, dispersion managed soliton-like solutions can exist even when \(D_{\text{net}}\) is zero. However, they exist only if the stretching factor is large, see Figure 6.17.
A remarkable property of the dispersion managed solitons is that they do not radiate (generate continuum) even though they propagate in a medium with abrupt dispersion changes. This can be understood by the fact, that the dispersion mangaged soliton is a solution of the underlaying dynamics incorporating already the periodic dispersion variations including the Kerr-effect. This is in contrast to the soliton in a continuously distributed dispersive environment, where periodic variations in dispersion and nonlinearity leads to radiation.
Bibliography
[1] G.H.C. New: "Pulse evolution in mode-locked quasicontinuous lasers," IEEE J. Quantum Electron. 10, 115-124 (1974)
[2] H. A. Haus, ”Theory of Mode Locking with a Slow Saturable Absorber,” IEEE J. Quantum Electron. 11, pp. 736 — 746 (1975).
[3] H. A. Haus, ”Theory of modelocking with a fast saturable absorber,” J. Appl. Phys. 46, pp. 3049 — 3058 (1975).
[4] H. A. Haus, J. G. Fujimoto, E. P. Ippen, ”Structures for additive pulse modelocking,” J. Opt. Soc. of Am. B 8, pp. 2068 — 2076 (1991).
[5] E. P. Ippen, ”Principles of passive mode locking,” Appl. Phys. B 58, pp. 159 — 170 (1994).
[6] F. X. Kärtner and U. Keller, ”Stabilization of soliton-like pulses with a slow saturable absorber,” Opt. Lett. 20, 16 — 19 (1995).
[7] F.X. Kärtner, I.D. Jung, U. Keller: TITLE, "Soliton Modelocking with Saturable Absorbers," Special Issue on Ultrafast Electronics, Photonics and Optoelectronics, IEEE J. Sel. Top. Quantum Electron. 2, 540-556 (1996)
[8] I. D. Jung, F. X. Kärtner, L. R. Brovelli, M. Kamp, U. Keller, ”Exper- imental verification of soliton modelocking using only a slow saturable absorber,” Opt. Lett. 20, pp. 1892 — 1894 (1995).
[9] C. Spielmann, P.F. Curley, T. Brabec, F. Krausz: Ultrabroad-band fem- tosecond lasers, IEEE J. Quantum Electron. 30, 1100-1114 (1994).
[10] Y. Chen, F. X. Kärtner, U. Morgner, S. H. Cho, H. A. Haus, J. G. Fujimoto, and E. P. Ippen, “Dispersion managed mode-locking,” J. Opt. Soc. Am. B 16, 1999-2004, 1999.
[11] K. Tamura, E.P. Ippen, H.A. Haus, L.E. Nelson: 77-fs pulse generation from a stretched-pulse mode-locked all-fiber ring laser, Opt. Lett. 18, 1080-1082 (1993)
[12] K. Tamura, E.P. Ippen, H.A. Haus: Pulse dynamics in stretched-pulse lasers, Appl. Phys. Lett. 67, 158-160 (1995)
[13] F.X. Kärtner, J. A. d. Au, U. Keller, "Mode-Locking with Slow and Fast Saturable Absorbers-What’s the Difference,". Sel. Top. Quantum Electron. 4, 159 (1998)
[14] J.H.B. Nijhof, N.J. Doran, W. Forysiak, F.M. Knox: Stable soliton-like propagation indispersion-managed system with net anomalous, zero, and nromal dispersion, Electron. Lett. 33, 1726-1727 (1997)
[15] Y. Chen, H.A. Haus: Dispersion-managed solitons in the net positive dispersion regime, J. Opt. Soc. Am. B 16, 24-30 (1999)
[16] H.A. Haus, K. Tamura, L.E. Nelson, E.P. Ippen, "Stretched-pulse ad- ditive pulse modelocking in fiber ring lasers: theory and experiment," IEEE J. Quantum Electron. 31, 591-598 (1995)
[17] I. D. Jung, F. X. Kärtner, N. Matuschek, D. H. Sutter, F. Morier- Genoud, Z. Shi, V. Scheuer, M. Tilsch, T. Tschudi, U. Keller, ”Semicon- ductor saturable absorber mirrors supporting sub-10 fs pulses,” Appl. Phys. B 65, pp. 137-150 (1997).

