4.5: Parallel Plate Waveguide
- Page ID
- 41040
The parallel-plate waveguide shown in Figure 4.4.1 is the closest regular structure to planar transmission lines such as a microstrip line. The aim here is to develop design guidelines that will enable transmission line structures to be designed to avoid multimoding.
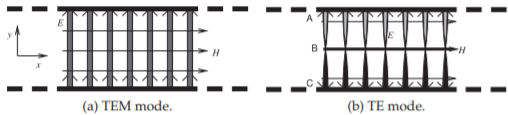
Figure \(\PageIndex{1}\): Parallel-plate waveguide showing electric, \(E\), and magnetic, \(H\), field lines. The electric \((E)\) and magnetic \((H)\) field lines are shown with the line thickness indicating field strength. The shading of the \(E\) field lines indicates polarity (\(+\) or \(−\)) and the arrows indicate direction (and also polarity). The \(z\) direction is into the page.
4.5.1 Electromagnetic Derivation
The development of the wave equations begins with Maxwell’s equations (Equations (1.5.1)–(1.5.4)). A simplification to the equations is to assume a linear, isotropic, and homogeneous medium, a uniform dielectric, so that \(\varepsilon\) and \(\mu\) are independent of signal level and are independent of the field direction and position. Thus (in the time domain)
\[\begin{align}\label{eq:1} \nabla\times\overline{\mathcal{E}}&=\frac{\partial\overline{\mathcal{B}}}{\partial t} &\nabla\times\overline{\mathcal{H}}&=\overline{\mathcal{J}}+\frac{\partial\overline{\mathcal{D}}}{\partial t} \\ \label{eq:2} \nabla\cdot\overline{\mathcal{D}}&=\rho_{V} &\nabla\cdot\overline{\mathcal{B}}&=0\end{align} \]
where \(\rho_{V}\) is the volume charge density and \(\overline{\mathcal{J}}\) is the current density. \(\overline{\mathcal{J}}\) and \(\rho_{V}\) will be zero except at an electric wall. The above equations do not include magnetic charge or magnetic current density. These do not actually exist and so a modified form of Maxwell’s equations incorporating these is not necessary to solve the fields on a structure.
In the lateral direction, the \(x\) direction, the parallel-plate waveguide in Figure 4.4.1 extends indefinitely. For this regular structure, and with a few assumptions, the form of Maxwell’s equations with multidimensional spatial derivatives can be simplified. One approach to solving differential equations is to assume a form of the solution and then test to see if it is a valid solution. The first solution to be considered is called the TEM mode and corresponds to the minimum possible variation of the fields. Also, it is assumed that the variation in the \(z\) direction is described by the traveling-wave equations. So the only fields of interest here are \(E\) and \(H\) in the transverse plane; all that is seen in Figure 4.4.1(b). If all the fields are in the \(xy\) plane, then it is sufficient to apply just the boundary conditions that come from the top and bottom ground planes. At first it appears that there are many possible solutions to the differential equations. This is simplified by assuming certain variational properties of the \(E_{x},\: E_{y},\: H_{x},\) and \(H_{y}\) fields and then seeing if these solutions can be supported. The simplest solution is when there is no variation in the fields and then the only possible outcome is that \(E_{x} =0= H_{y}\). This is the TEM mode indicated in Figure \(\PageIndex{1}\)(a). At the boundaries, the top and bottom metal planes, there is a divergence of the electric field, as immediately inside the (ideal) conductor there is no electric field and immediately outside there is. This divergence is supported by the surface charge on the ground planes (see Equation (1.5.2)).
In Figure \(\PageIndex{1}\)(a), the thickness of the lines indicates relative field strength and there is no variation in field strength in either the electric or magnetic fields shown. The coefficients of the field components are determined by the boundary conditions. The trial solution used here has \(E_{x} =0= H_{y}\) and \(E_{z} = 0 = H_{z}\); that is, the trial solution has the electric field in the y direction and the magnetic field is in the x direction. Maxwell’s equations (Equations \(\eqref{eq:1}\) and \(\eqref{eq:2}\)) become (note that \(\overline{\mathcal{E}} =\overline{\mathcal{E}}_{x}\hat{\mathbf{x}} +\overline{\mathcal{E}}_{y}\hat{\mathbf{y}}+\overline{\mathcal{E}}_{z}\hat{\mathbf{z}}\))
\[\begin{align}\label{eq:3}\nabla\times\overline{\mathcal{E}}_{y}\hat{\mathbf{y}}&=-\frac{\partial\overline{\mathcal{B}}_{x}\hat{\mathbf{x}}}{\partial t} &\nabla\times\overline{\mathcal{H}}_{x}\hat{\mathbf{x}}&=\frac{\partial\overline{\mathcal{D}}_{y}\hat{\mathbf{x}}}{\partial t}+\overline{\mathcal{J}} \\ \label{eq:4}\nabla\cdot\overline{\mathcal{D}}_{y}\hat{\mathbf{y}}&=\rho &\nabla\cdot\overline{\mathcal{B}}_{x}\hat{\mathbf{x}}&=0\end{align} \]
Expanding the curl, \(\nabla\times\), and div, \(\nabla\cdot\), operators using Equations (1.5.21) and (1.5.22) these become (with \(D =\varepsilon E\) and \(B =\mu H\))
\[\begin{align}\label{eq:5}\hat{x}\frac{\partial\overline{\mathcal{E}}_{y}}{\partial z}&=\frac{\partial\overline{\mathcal{B}}_{x}\hat{\mathbf{x}}}{\partial t}=\frac{\partial\mu\overline{\mathcal{H}}_{x}\hat{\mathbf{x}}}{\partial t} \\ \label{eq:6} \hat{\mathbf{y}}\frac{\partial\overline{\mathcal{H}}_{x}}{\partial z} &=\frac{\partial\overline{\mathcal{D}}_{y}\hat{\mathbf{y}}}{\partial t}+\overline{\mathcal{J}}=\frac{\partial\varepsilon\overline{\mathcal{E}}_{y}\hat{\mathbf{y}}}{\partial t}+\overline{\mathcal{J}} \\ \label{eq:7}\frac{\partial\overline{\mathcal{D}}_{y}}{\partial y}=\frac{\partial\varepsilon\overline{\mathcal{E}}_{y}}{\partial y}&=\rho \\ \label{eq:8}\frac{\partial\overline{\mathcal{B}}_{x}}{\partial x}=\frac{\partial\mu\overline{\mathcal{H}}_{x}}{\partial x}&=0\end{align} \]
These equations describe what happens at each point in space.
Equation \(\eqref{eq:5}\) indicates that if there is a time-varying component of the \(x\)-directed \(B\) field then there must be a \(z\)-varying component of the \(E_{y}\) field component. This is just part of the wave equation describing a field propagating in the \(z\) direction. Equation \(\eqref{eq:6}\) indicates the same thing, but now the roles of the electric and magnetic fields are reversed. Equation \(\eqref{eq:5}\) shows that the \(y\) component of the electric field can be constant between the plates, but at the plates there must be a charge on the surface of the conductors (see Equation \(\eqref{eq:7}\)), to terminate the electric field (as indicated by Equation \(\eqref{eq:7}\)), as there is no electric field inside the conductors. Equation \(\eqref{eq:8}\) indicates that the \(x\) component of the magnetic field cannot vary in the \(x\) direction (i.e., \(B_{x}\) and \(H_{x}\) are constant). Thus the assumption behind the trial solution about the form of this mode is correct. The electric and magnetic field are uniform in the transverse plane, the \(xy\) plane, and the only variation is in the direction of propagation, the \(z\) direction (but there is no \(z\)-directed component of the fields). Thus the test solution satisfies Maxwell’s equations. This is the TEM mode, as all field components are in the \(x\) and \(y\) directions and none are in the \(z\) direction. The TEM mode can be supported at all frequencies in the parallel-plate waveguide.
In exploring the existence of higher-order modes, Maxwell’s equations need to be simplified further. Putting Equations \(\eqref{eq:5}\)–\(\eqref{eq:8}\) in phasor form, and considering the source free region between the plates (so \(J = 0\) and \(\rho = 0\)), these become (note that \(E_{x}\) is the phasor of \(E_{x}\))
\[\begin{align}\label{eq:9}\frac{\partial E_{y}}{\partial z}&=\jmath\omega\mu H_{x}&\frac{\partial H_{x}}{\partial z}&=\jmath\omega\varepsilon E_{y} \\ \label{eq:10}\frac{\partial E_{y}}{\partial y}&=0 &\frac{\partial H_{x}}{\partial x}&=0\end{align} \]
and the solution becomes
\[\label{eq:11}\frac{\partial^{2}E_{y}}{\partial z^{2}}=\gamma^{2}E_{y}\quad\text{and}\quad\frac{\partial^{2}H_{x}}{\partial z^{2}}=\gamma^{2}H_{x} \]
where \(\gamma =\jmath\omega\sqrt{\mu\varepsilon}\) and there is no variation of the \(E_{y}\) component of the electric field in the \(y\) direction and no variation of the \(H_{x}\) component of the magnetic field in the \(x\) direction.
Another possible set of modes occurs when the electric field is only in the y direction, but then there must be a variation of the field strength, as shown in Figure \(\PageIndex{1}\)(b). The simplest variation is when there is a half-sinusoidal spatial variation of the \(E_{y}\) field component. Applying the methodology described above, it is found that there must be a component of the magnetic field in the \(z\) direction to support this mode. Hence these modes are called transverse electric (TE) modes. (Interchanging the roles of the electric and magnetic fields yields the transverse magnetic (TM) modes where there is an electric field component in the \(z\) direction.) The half-sinusoidal variation still enables the charge to support the existence of an \(E_{y}\) electric field.
A key result from our previous discussion is that there must be enough distance for the field to curl and this is related to wavelength, \(\lambda\). This transverse electric mode can only exist when \(h ≥ \lambda/2\). When \(h\) is smaller than one-half wavelength, this mode cannot be supported, and is said to be cut off. Only the TEM mode can be supported all the way down to DC, so modes other than TEM have a cutoff frequency, \(f_{c}\), and a cutoff wavelength, \(\lambda_{c}\). The concept of wavenumber \(k(= 2π/\lambda=\omega\sqrt{\mu\varepsilon})\) is also used. The cutoff wavelength, \(\lambda_{c}\), and the cutoff wavenumber, \(k_{c}\), are both related to the dimension below which a mode cannot “curl” sufficiently to be self-supporting. For the lowest TE mode, \(k_{c} = 2π/\lambda_{c}\) with \(\lambda_{c} = 2h\). In general, for TE modes, there can be \(n\) variations of the electric field, and \(n\) indicates the \(n\)th TE mode, denoted as \(\text{TE}_{n}\), for which \(k_{c,n} = n\pi/h\) and \(\lambda_{c} = 2h/n\). The propagation constant of any mode in a uniform lossless medium (not just in a parallel-plate waveguide mode) is
\[\label{eq:12}\beta=\sqrt{k^{2}-k_{c}^{2}} \]
For the TEM mode, \(k_{c} = 0\). High-order modes are described by their own \(k_{c}\).
TM modes are similarly described, and again \(k_{c} = n\pi /h\) for the \(\text{TM}_{n}\) mode. A mode can be supported at any frequency above the cutoff frequency of the mode, it just cannot be supported at frequencies below the cutoff frequency as it is not possible for the fields to vary (or curl) below cutoff.
4.5.2 Multimoding and Electric and Magnetic Walls
In the above discussion, the parallel-plate waveguide had two electric walls—the top and bottom metal walls. Here results will be presented when magnetic walls are introduced. A magnetic wall can only be approximated, as magnetic conductors do not exist (since magnetic charges do not exist). Whereas an electric wall appears as a short circuit, a magnetic wall is an open circuit. Maxwell’s equations and the electric and magnetic walls establish the following requirements on the fields:
| Electric wall |
Perpendicular electric field Tangential magnetic field |
| Magnetic wall |
Perpendicular magnetic field Tangential electric field |
Table \(\PageIndex{1}\): Requirements imposed on fields by electric and magnetic walls.
The lowest-order modes that can be supported by combinations of electric and magnetic walls are shown in Figure 4.6.1. With two electric or two magnetic walls, a TEM mode (having no field variations in the transverse plane) can be supported. Of course, there will be variations in the field components in the direction of propagation. The modes with the simplest geometric variations in the plane transverse to the direction of propagation establish the critical wavelength. In Figure 4.6.1 the distance between the walls is \(d\). For the case of two like walls (Figures 4.6.1(a and c)), \(\lambda_{c} = 2h\), as one-half sinusoidal variation is required. With unlike walls (see Figure 4.6.1(b)), the varying modes are supported with just one-quarter sinusoidal variation, and so \(\lambda_{c} = 4h\).
A magnetic wall and an electric wall are \(1\text{ cm}\) apart and are separated by a lossless material having \(\varepsilon_{r} = 9\). What is the cut-off frequency of the lowest-order mode in this system?
Solution
The EM field established by the electric and magnetic walls is described in Figure 4.6.1(b). There is no solution to the Maxwell’s equations that has no variation of the EM fields since it is not possible to have a spatially uniform electric field which is perpendicular to an electric wall while also being perpendicular to a parallel magnetic wall. The other solutions of Maxwell’s equations require that the fields vary spatially, i.e. curl. Without electric and magnetic walls the minimum distance over which the EM fields will fold back on to themselves is a wavelength. With parallel electric and magnetic wall separated by \(h\) the minimum distance for a solution of Maxwell’s equations is a quarter wavelength, \(\lambda\), of the walls as shown on the right in Figure 4.6.1(b). That is
\[\label{eq:13}h=\lambda/4\quad\text{or}\quad\lambda=4h=4\text{ cm}=\lambda_{0}/(\sqrt{\varepsilon_{t}\mu_{r}}) \]
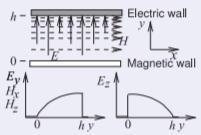
Figure \(\PageIndex{2}\)
Since the relative permeability has not been specified assume that \(\mu_{r} = 1\) so
\[\lambda_{0}=\lambda\sqrt{9}=12\text{ cm}=c/f\nonumber \]
The cut-off frequency is
\[\begin{align} f&=(2.998 × 10^{8}\text{ m/s})/(0.12\text{ m})\nonumber \\ \label{eq:14}&=2.498\text{ GHz}\end{align} \]


