3.7: Summary and Further Problems

Last updated
Save as PDF

Page ID: 30842

\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \) \( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)\(\newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\) \( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\) \( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\) \( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\) \( \newcommand{\Span}{\mathrm{span}}\) \(\newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\) \( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\) \( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\) \( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\) \( \newcommand{\Span}{\mathrm{span}}\)\(\newcommand{\AA}{\unicode[.8,0]{x212B}}\)

In the midst of change, find what does not change—the invariant or conserved quantity. Finding these quantities simplifies problems: We focus on the few quantities that do not change rather than on the many ways in which quantities do change. An instance of this idea with wide application is a box model, where what goes in must come out. By choosing suitable boxes, we could estimate rainfalls and drag forces, and understand lift.

Exercise \(\PageIndex{1}\): Raindrop speed

Use the drag force \(F_{drag} \sim \rho A_{cs} v^{2}\) to estimate the terminal speed of a typical raindrop with a diameter of 0.5 centimeters. How could you check the prediction?

Exercise \(\PageIndex{2}\): Average value of sin squared

Use symmetry to find the average value of sin²t over the interval t = [0,\(\pi\)].

Exercise \(\PageIndex{3}\): Moment of inertia of a spherical shell

The moment of inertia of an object about an axis of rotation is

\[\sum{m_{i}d_{i}^{2}}\]

summed over all mass points i, where d_iis the distance of the point from an axis of rotation. Use symmetry to find the moment of inertia of a spherical shell with mass m and radius r about an axis through its center. You shouldn’t need to do any integrals!

Exercise \(\PageIndex{4}\): Flying bicyclist

Estimate the wingspan a world-champion bicyclist would require in order to get enough lift for takeoff.

Exercise \(\PageIndex{5}\): Maximum-gain frequency for a second-order system

In this problem, you use symmetry to maximize the gain of an LRC circuit or a spring–mass system with damping (using the analogy in Section 2.4.1). The gain G, which is the amplitude ratio V_out/V_in, depends on the signal’s angular frequency \(\omega\)

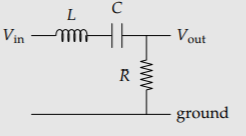
\[G(\omega) = \frac{\frac{j\omega}{\omega_{0}}}{1 + \frac{j}{Q} \frac{\omega}{\omega_{0}}-\frac{\omega^{2}}{\omega^{2}_{0}}}\]

where \(j = \sqrt{-1}\), \(\omega_{0}\) is the natural frequency of the system, and Q, the quality factor, is a dimensionless measure of the damping. Don’t worry about where the gain formula comes from: You can derive it using the impedance method (Problem 2.22), but the purpose of this problem is to maximize its magnitude \(| G (\omega)|\). Do so by finding a symmetry operation on \(\omega\) that leaves \(|G(\omega)|\) invariant.

Exercise \(\PageIndex{6}\): Runway length

Estimate the runway length required by a 747 in order to take off.

Exercise \(\PageIndex{7}\): Hovering versus flying

At what forward flight speed does the hummingbird of Section 3.6.1 require as much power to generate lift as it would to hover? How does this speed compare to its typical flight speed?

Exercise \(\PageIndex{8}\):Resistive grid

In an infinite grid of 1-ohm resistors, what is the resistance measured across one resistor?

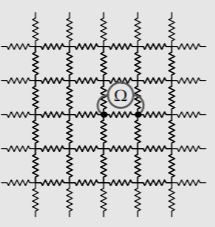
To measure resistance, an ohmmeter injects a current I at one terminal (for simplicity, imagine that I = 1 ampere). It removes the same current from the other terminal, and measures the resulting voltage difference V between the terminals. The resistance is R=V/I.

Hint: Use symmetry. But it’s still a hard problem!

Exercise \(\PageIndex{9}\): Inertia tensor

Here is an inertia tensor (the generalization of moment of inertia) of a particular object, calculated in an ill-chosen (but Cartesian) coordinate system:

\[\left (\begin{array}{ccc} 4 & 0 & 0 \\ 0 & 5 & 4 \\ 0 & 4 & 5 \end{array} \right) \]

a. Change the coordinate system to a set of principal axes, where the inertia tensor has the diagonal form

\[ \left (\begin{array}{ccc} I_{xx} & 0 & 0 \\ 0 & I_{yy} & 0 \\ 0 & 0 & I_{zz} \end{array} \right) \]

and give the principal moments of inertia I_xx , I_{yy ,} and I_zz. Hint: Which properties of a matrix are invariant when changing coordinate systems?

b. Give an example of an object with a similar inertia tensor. Rhetorical question: In which coordinate system is it easier to think of such an object?

This problem was inspired by a problem on the physics written qualifying exam during my days as a PhD student. The problem required diagonalizing an inertia tensor, and there was too little time to rederive or even apply the change-of-basis formulas. Time pressure sometimes pushes one toward better solutions!

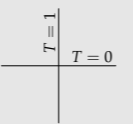
Exercise \(\PageIndex{10}\): Temperature distribution on an infinite sheet

On this infinite, uniform sheet, the x axis is held at zero temperature, and the y axis is held at unit temperature (T= 1). Find the temperature everywhere (except the origin!). Use Cartesian coordinates \(T(x,y)\) or polar coordinates \(T(r, \theta)\), whichever choice makes it easier to describe the temperature.