8.4: Limiting cases
- Page ID
- 18084
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)The nondimensional parameter \(kH\) is the ratio of water depth to wavelength (times 2\(\pi\)). Two important limiting cases are \(kH\rightarrow\inf\) (short waves or deep water) and \(kH \rightarrow 0\) (long waves or shallow water).
8.4.1 Deep water (short) waves
In the short wave limit \(|kH|\rightarrow\inf\) we can simplify the dispersion relation using:
\[\lim _{k H \rightarrow \infty} \tanh k H=1. \nonumber \]
Substituting this into Equation 8.2.35 or Equation 8.2.36 gives
\[\omega=(g k)^{1 / 2}, \quad \text { or } \quad c=\left(\frac{g}{k}\right)^{1 / 2}. \nonumber \]
The group velocity is obtained from the first of these:
\[c_{g}=\frac{\partial \omega}{\partial k}=\frac{1}{2}(g k)^{-1 / 2} g=\frac{1}{2}\left(\frac{g}{k}\right)^{1 / 2}=\frac{1}{2} c. \nonumber \]
This relationship between group and phase velocities is often visible in the wake of a small boat or canoe. The wake propagates to either side of the boat’s trajectory as a wave pulse, or envelope. If you look carefully, the envelope is constantly changing its shape as individual wave crests appear at the back, propagate to the front, and disappear. This is because they propagate with the phase velocity while the pulse as a whole propagates at the group velocity, only half as fast (see Figure 8.3.2).
8.4.2 Shallow water (long) waves
We now consider the opposite limit, \(kH\rightarrow 0\). When \(kH \ll 1\), the \(\tanh\) function is approximated as
\[\tanh k H=k H. \nonumber \]
Using this approximation in Equation 8.2.36 leads to
\[c=(g H)^{1 / 2}.\label{eqn:1} \]
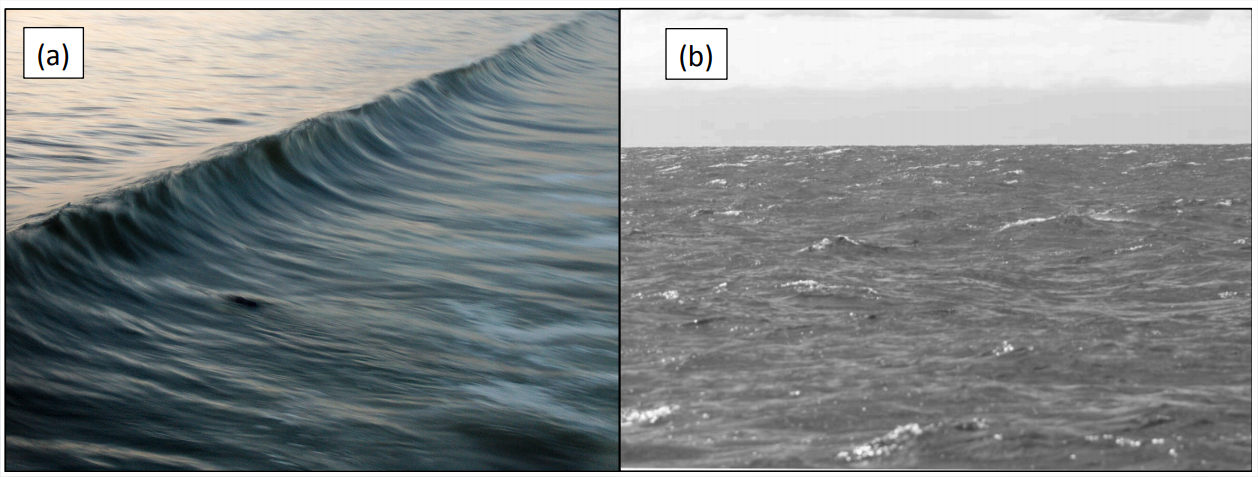
Note that this phase speed is the same for all frequencies. We therefore say that shallow water waves are nondispersive. An arbitrary wave shape made of many such waves will retain its shape rather than dispersing, because all of its Fourier components travel with the same speed (e.g., Figure \(\PageIndex{1}\)a). Bores and tsunamis are examples. Another indication of the nondispersive character of shallow water waves is the group velocity, computed from Equation \(\ref{eqn:1}\) as
\[c_{g}=\frac{\partial}{\partial k}(k c)=c, \nonumber \]
i.e., the phase and group velocities are the same. In this regime a wave superposition such as that shown in Figure 8.3.1 retains its shape.
In contrast, deep-water waves do not retain their shape but instead have an irregular, choppy appearance (Figure \(\PageIndex{1}\)b). This is because they are dispersive, i.e., their different Fourier components travel at different speeds.
Referring to Figure 8.2.2 we see that \(\left( gH\right)^{1/2}\) is the fastest speed possible for small-amplitude surface waves. In most of the ocean, \(H\) is about 4000 m, so \(\left( gH\right)^{1/2}\) = 200 m/s. This is the speed of a tsunami crossing the Pacific, for example. The shallow water limit applies, even though the depth is great, because the wavelength of a tsunami is greater. We will refer to this speed as the linear long wave speed.
The Froude number
Suppose that something disturbs the surface of a channel so as to radiate waves both upstream and downstream. The fastest waves will travel at \(c =\pm c_0\), where \(c_0=\left( gH\right)^{1/2}\) is the linear long wave speed. Now suppose that the water in the channel is flowing at speed \(u\). If \(u < c_0\), then long waves will still radiate both upstream and downstream, but the upstream waves will move more slowly (to a stationary observer). But if \(u = c_0\) the motion of the upstream wave will be arrested, and if \(u > c_0\) there will be no propagation upstream. This distinction is quantified by the Froude number1:
\[F=\frac{|u|}{(g H)^{1 / 2}}./label{eqn:2} \nonumber \]
The flow is called critical if \(F\) = 1, supercritical (no upstream propagation) if \(F\) > 1 and subcritical (propagation in both directions) if \(F\) < 1.
If you turn back to Figure 1.1.1 and look at the upper right frame, you will see a rather dramatic transition from smooth to turbulent flow in the Nile River. Upstream of the transition, the flow is smooth, almost glassy, apparently unaffected by the violent churning of the water only a short distance away. The upstream flow is supercritical, so that disturbances from the turbulent region downstream cannot reach it. At the transition, the flow changes from supercritical to subcritical. This happens because the flow slows down and gets deeper. (Verify by inspection of Equation \(\ref{eqn:2}\) that both of these changes act to reduce the Froude number.) This transition is called a hydraulic jump. In the downstream, subcritical region disturbances can propagate in all directions, and they clearly do. We will have much more to say about hydraulic jumps and the Froude number in chapter 9.
Vertical structure
We turn next to an examination of the vertical structure of the velocity and pressure fields in the shallow water regime. The vertical structure function for the horizontal velocity component is
\[U(z)=\omega \eta_{0} \frac{\cosh k(z+H)}{\sinh k H}, \nonumber \]
reproduced from Equation 8.2.39 . The limit of \(\cosh kH\) as \(kH\rightarrow 0\) is 1. The same is true of \(\cosh k(z+H)\) because \(|z+H| \leq H\). On the other hand, \(\sinh kH \approx kH\) for \(|kH| \ll 1\). Therefore, in the shallow water limit, we find that the horizontal velocity is independent of depth:
\[U=\frac{\omega \eta_{0}}{k H}=c \frac{\eta_{0}}{H}. \nonumber \]
For the vertical velocity, we begin with
\[W(z)=\omega \eta_{0} \frac{\sinh k(z+H)}{\sinh k H}, \nonumber \]
reproduced from 8.2.32. As \(kH\rightarrow 0\), \(\sinh kH \approx kH\), and also \(\sinh k(z+H) \approx k(z+H)\) because \(|z+H| \leq H\). Meanwhile, \(\cosh kH \approx 1\) as before. Therefore:
\[W(z)=\omega \eta_{0} \frac{k(z+H)}{k H}=\omega \eta_{0}\left(1+\frac{z}{H}\right). \nonumber \]
Vertical velocity is a linear function of depth with a nonzero surface value \(W(0) = \omega\eta_0\).
The vertical distribution of the nonhydrostatic pressure is
\[P=\rho_{0} g \eta_{0} \frac{\cosh k(z+H)}{\cosh k H}; \nonumber \]
(reproduced from Equation 8.2.31). Again, the limit of \(\cosh kH\) as \(kH \rightarrow 0\) is 1, and the same is true of \(\cosh k(z+H)\). The nonhydrostatic pressure is independent of depth:
\[P=\rho_{0} g \eta_{0}. \nonumber \]
With the \(x\) and \(t\) dependence restored,
\[p^{*}=\rho_{0} g \eta_{0} \cos (k x-\omega t)=\rho_{0} g \eta. \nonumber \]
This is just the weight of the water between \(z\) = 0 and \(z\) = \(\eta\) (negative if \(η\) < 0), i.e., what we have been calling the “nonhydrostatic pressure” actually becomes hydrostatic in the long-wave limit. This is because vertical accelerations are extremely small, so the vertical pressure gradient almost balances gravity. Adding this to the hydrostatic pressure for the motionless state \(−\rho_0 gz\), we obtain the total pressure
\[p=\rho_{0} g(\eta-z). \nonumber \]
The total pressure is therefore hydrostatic even in the presence of long waves.
1William Froude (1810-1870) was a British naval architect concerned with designing an efficient hull shape for naval vessels. As a ship moves, it radiates waves, and those waves rob the ship of its momentum. The resulting drag increases when the ship speed exceeds the maximum wave speed (i.e., the motion becomes supercritical), and it is therefore dependent on what we now call the Froude number.


