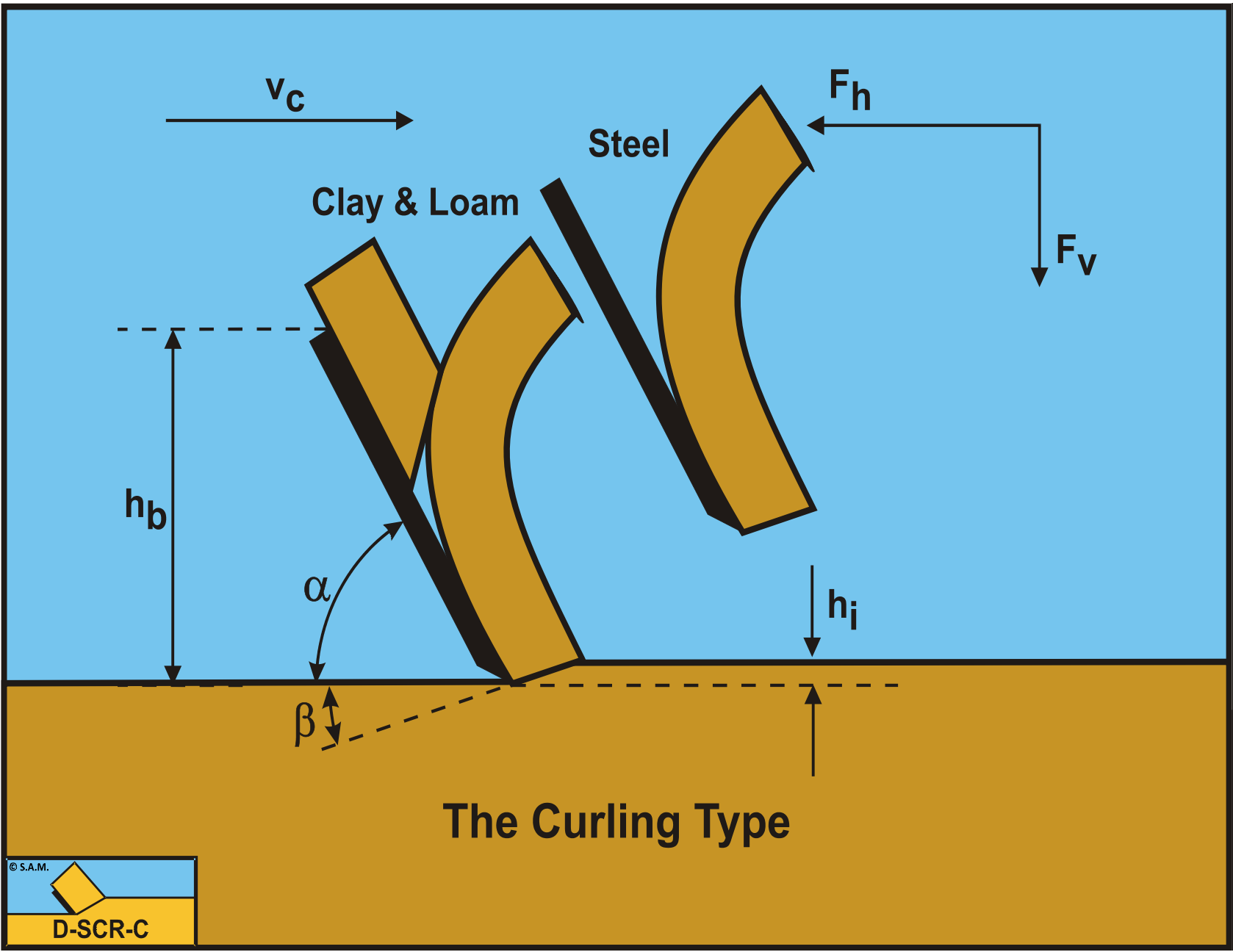
3.4: The Curling Type
- Page ID
- 29432
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
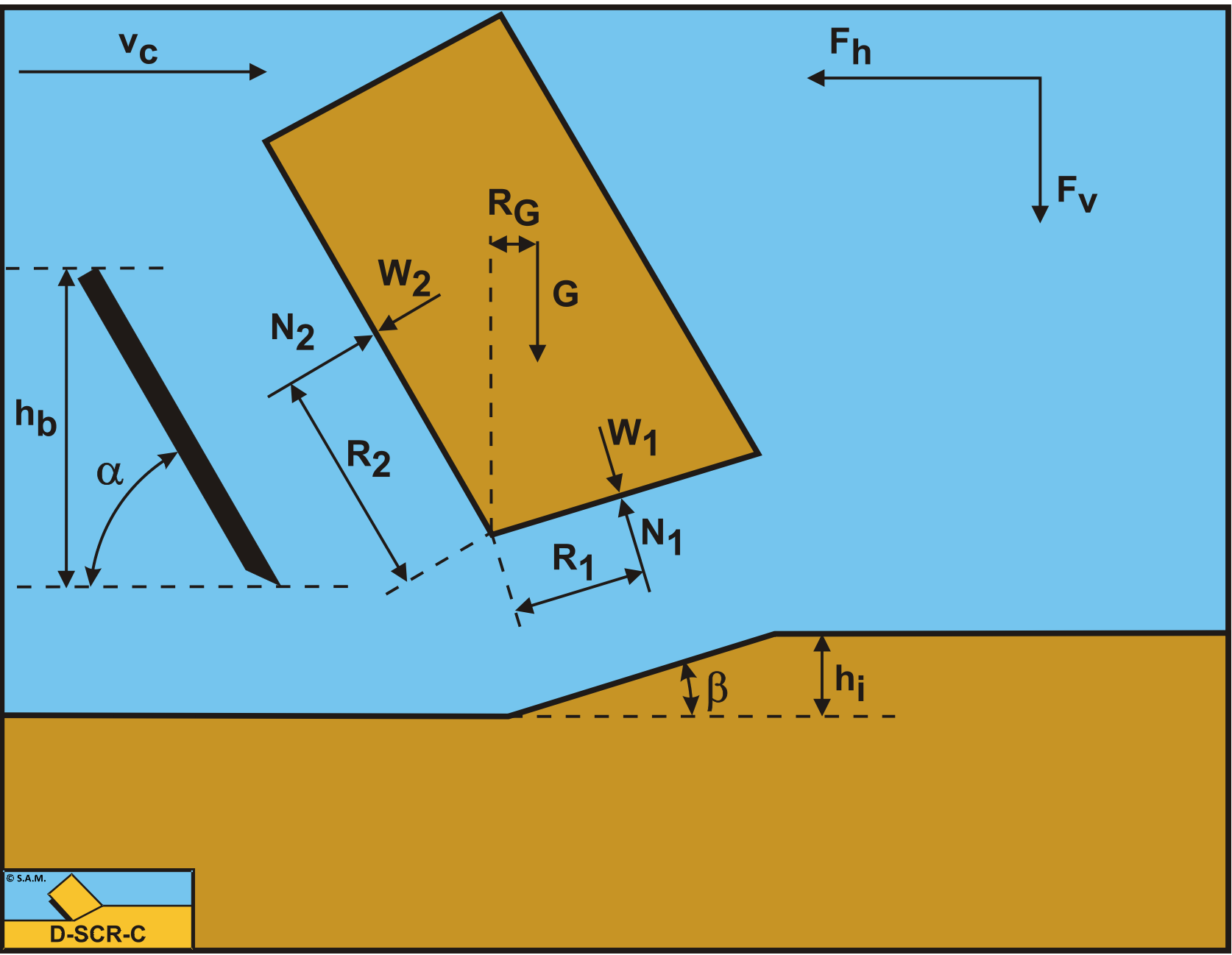
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)In some soils it is possible that the Curling Type of cutting mechanism occurs. This will happen when the layer cut is relatively thin and there is a force on the blade of which the magnitude depends on the blade height, like the adhesive force or the pore pressure force in the case of a cavitating cutting process. In soils like clay and loam, but also in rock under hyperbaric conditions this may occur. Figure 3-8 shows this Curling Type. The question now is, what is the effective blade height hb,m where the soil is in contact with the blade. To solve this problem, an additional equation is required. There is only one equation available and that is the equilibrium equation of moments on the layer cut. Figure 3-9 shows the moments acting on the layer cut. In the case of clay, loam or hyperbaric rock, the contribution of gravity can be neglected.
The equilibrium of moments when the gravity moment is neglected is:
\[\ \left(\mathrm{N}_{\mathrm{1}}-\mathrm{W}_{\mathrm{1}}\right) \cdot \mathrm{R}_{\mathrm{1}}=\left(\mathrm{N}_{\mathrm{2}}-\mathrm{W}_{\mathrm{2}}\right) \cdot \mathrm{R}_{\mathrm{2}}\tag{3-21}\]
The arms of the 2 moments are:
\[\ \mathrm{R_{1}=\frac{\lambda_{1} \cdot h_{\mathrm{i}}}{\sin (\beta)}, R_{2}=\frac{\lambda_{2} \cdot h_{\mathrm{b}, \mathrm{m}}}{\sin (\alpha)}}\tag{3-22}\]
This gives the equilibrium equation of moments on the layer cut:
\[\ \left(\begin{array}{c}\frac{\mathrm{W}_{2} \cdot \mathrm{s i n}(\delta)+\mathrm{W}_{1} \cdot \sin (\alpha+\beta+\delta)}{\sin (\alpha+\beta+\delta+\varphi)} \cdot \cos (\varphi) \\ +\frac{-\mathrm{C} \cdot \cos (\alpha+\beta+\delta)+\mathrm{A} \cdot \cos (\delta)}{\sin (\alpha+\beta+\delta+\varphi)} \cdot \cos (\varphi) \\ -\mathrm{W}_{1}\end{array}\right) \cdot \frac{\lambda_{1} \cdot \mathrm{h}_{\mathrm{i}}}{\sin (\beta)}=\left(\begin{array}{c}\frac{\mathrm{W}_{2} \cdot \sin (\alpha+\beta+\varphi)+\mathrm{W}_{1} \cdot \sin (\varphi)}{\sin (\alpha+\beta+\delta+\varphi)} \cdot \cos (\delta) \\ +\frac{\mathrm{C} \cdot \cos (\varphi)-\mathrm{A} \cdot \cos (\alpha+\beta+\varphi)}{\sin (\alpha+\beta+\delta+\varphi)} \cdot \cos (\delta) \\ -\mathrm{W}_{2}\end{array}\right) \cdot \frac{\lambda_{2} \cdot \mathrm{h}_{\mathrm{b}, \mathrm{m}}}{\sin (\alpha)}\tag{3-23}\]
When the equations for W1, W2, C and A as mentioned before are substituted, the resulting equation is a second degree equation with hb,m as the variable.
This can be solved using the following set of equations:
\[\ \begin{array}{left} \mathrm{A} &\cdot \mathrm{x}^{2}+\mathrm{B} \cdot \mathrm{x}+\mathrm{C}=\mathrm{0} \quad\text { and }\quad \mathrm{h}_{\mathrm{b}, \mathrm{m}}=\mathrm{x}=\frac{-\mathrm{B} \pm \sqrt{\mathrm{B}^{2}-\mathrm{4} \cdot \mathrm{A} \cdot \mathrm{C}}}{\mathrm{2} \cdot \mathrm{A}} \\
\mathrm{A}&=\frac{\lambda_{2} \cdot \mathrm{p}_{2 \mathrm{m}} \cdot \sin (\alpha+\beta+\delta+\varphi)-\lambda_{2} \cdot \mathrm{p}_{2 \mathrm{m}} \cdot \sin (\alpha+\beta+\varphi) \cdot \cos (\delta)}{\sin (\alpha) \cdot \sin (\alpha)} \\
&+\frac{+\mathrm{a} \cdot \lambda_{2} \cdot \cos (\alpha+\beta+\varphi) \cdot \cos (\delta)}{\sin (\alpha) \cdot \sin (\alpha)} \\
\mathrm{B}&=\frac{\lambda_{1} \cdot \mathrm{p}_{2 \mathrm{m}} \cdot \sin (\delta)\cdot \mathrm{cos(\varphi)}-\lambda_{2} \cdot \mathrm{p}_{1 \mathrm{m}} \cdot \cos (\delta) \cdot \sin (\varphi)}{\sin (\alpha) \cdot \sin (\beta)}\cdot \mathrm{h}_{\mathrm{i}}\\
&+\frac{-\mathrm{c} \cdot \lambda_{2} \cdot \cos (\delta) \cdot \cos (\varphi)+\mathrm{a} \cdot \lambda_{1} \cdot \cos (\varphi) \cdot \cos (\delta)}{\sin (\alpha) \cdot \sin (\beta)} \cdot \mathrm{h}_{\mathrm{i}} \\
\mathrm{C}&=\frac{\lambda_{1} \cdot \mathrm{p}_{1 \mathrm{m}} \cdot \sin (\alpha+\beta+\delta)\cdot \mathrm{cos(\varphi)}-\lambda_{1} \cdot \mathrm{p}_{1 \mathrm{m}} \cdot \sin (\alpha+\beta+\delta+\varphi) }{\sin (\beta) \cdot \sin (\beta)}\cdot \mathrm{h_i \cdot h_i}\\
&+\frac{-\mathrm{c} \cdot \lambda_{1} \cdot \cos (\alpha+\beta+\delta) \cdot \cos (\varphi)}{\sin (\beta) \cdot \sin (\beta)} \cdot \mathrm{h}_{\mathrm{i}} \cdot \mathrm{h}_{\mathrm{i}}\end{array}\tag{3-24}\]
The usage is now as follows:
\[\ \begin{array}{left}\text{if } \mathrm{h}_{\mathrm{b}, \mathrm{m}}<\mathrm{h}_{\mathrm{b}}\text{ then use } \mathrm{h}_{\mathrm{b}, \mathrm{m}}\\
\text{if }\mathrm{h}_{\mathrm{b}, \mathrm{m}} \geq \mathrm{h}_{\mathrm{b}}\text{ then use }\mathrm{h}_{\mathrm{b}}\end{array}\tag{3-25}\]


