4.1: Rate Equations

In section 2.5, we derived for the interaction of a two-level atom with a laser field propagating to the right the equations of motion (2.5.13) and (2.5.14), which are given here again:

\[\left ( \dfrac{\partial}{\partial z} + \dfrac{1}{v_g} \dfrac{\partial}{\partial t} \right ) A(z, t) = \dfrac{N\hbar}{4T_2 E_s} w (z, t) A(z, t), \label{4.1.1} \]

\[\dot{w} = -\dfrac{w - w_0}{T_1} + \dfrac{|A(z,t)|^2}{E_s} w(z, t), \label{4.1.2} \]

where \(T_1\) is the energy relaxation rate, \(v_g\) the group velocity in the host material where the two level atoms are embedded, \(E_s = I_sT_1\), the saturation fluence [\(J/cm^2\)] , of the medium.and \(I_s\) the saturation intensity according to Equation (2.4.7)

\[I_s = \left [\dfrac{2T_1T_2Z_F}{\hbar} \dfrac{|\vec{M} \hat{\vec{E}}|^2}{|\hat{\vec{E}}|^2} \right ]^{-1},\nonumber \]

which relates the saturation intensity to the microscopic parameters of the transition like longitudinal and transversal relaxation rates as well as the dipole moment of the transition.

Figure 4.1: Rate equations for the two-level atom

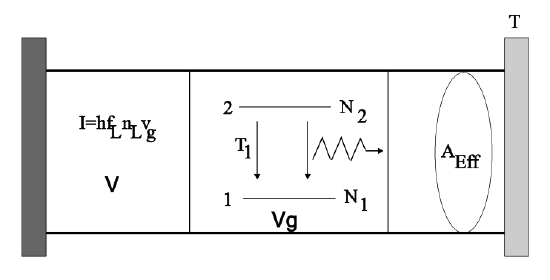
In many cases it is more convenient to normalize Equations \ref{4.1.1} and \ref{4.1.2} to the populations in level \(\text{e}\) and \(\text{g}\) or 2 and 1, respectively, \(N_2\) and \(N_1\), and the density of photons, \(n_L\), in the mode interacting with the atoms and traveling at the corresponding group velocity, vg, see Figure 4.1. The intensity \(I\) in a mode propagating at group velocity \(v_g\) with a mode volume \(V\) is related to the number of photons \(N_L\) stored in the mode with volume \(V\) by

\[I = hf_L \dfrac{N_L}{2^*V} v_g = \dfrac{1}{2^*} hf_Ln_Lv_g, \nonumber \]

where \(hf_L\) is the photon energy. \(2^* = 2\) for a linear laser resonator (then only half of the photons are going in one direction), and \(2^* = 1\) for a ring laser. In this first treatment we consider the case of space-independent rate equations, i.e. we assume that the laser is oscillating on a single mode and pumping and mode energy densities are uniform within the laser material. With the interaction cross section \(\sigma\) defined as

\[\sigma = \dfrac{hf_L}{2^*I_s T_1},\label{eq4.1.4} \]

and multiplying Equation (??) with the number of atoms in the mode, we obtain

\[\dfrac{d}{dt} (N_2 - N_1) = -\dfrac{(N_2 - N_1)}{T_1} - \sigma (N_2 - N_1) v_g n_L + R_p \nonumber \]

Note, \(v_gn_L\) is the photon flux, thus σ is the stimulated emission cross section between the atoms and the photons. \(R_p\) is the pumping rate into the upper laser level. A similar rate equation can be derived for the photon density

\[\dfrac{d}{dt} n_L = -\dfrac{n_L}{\tau_p} + \dfrac{l_g}{L} \dfrac{\sigma v_g}{V_g} [N_2 (n_L + 1) - N_1 n_L].\label{eq4.1.6} \]

Here, \(\tau_p\) is the photon lifetime in the cavity or cavity decay time and the one in Eq.(\(\ref{4.1.6}\)) accounts for spontaneous emission which is equivalent to stimulated emission by one photon occupying the mode. \(V_g\) is the volume of the active gain medium. For a laser cavity with a semi-transparent mirror with transmission \(T\), producing a small power loss \(2l = - \ln(1 - T) \approx T\) (for small \(T\)) per round-trip in the cavity, the cavity decay time is \(\tau_p = 2l/T_R\), if \(T_R = 2^*L/c_0\) is the roundtrip-time in linear cavity with optical length \(2L\) or a ring cavity with optical length \(L\). The optical length \(L\) is the sum of the optical length in the gain medium \(n_{group} l_g\) and the remaining free space cavity length \(l_a\). Internal losses can be treated in a similar way and contribute to the cavity decay time. Note, the decay rate for the inversion in the absence of a field, \(1/T_1\), is not only due to spontaneous emission, but is also a result of non radiative decay processes. See for example the four level system shown in Figure 4.2. In the limit, where the populations in the third and first level are zero, because of fast relaxation rates, i.e. \(T_{32}\), \(T_{10} \to 0\), we obtain

\[\dfrac{d}{dt} N_2 = -\dfrac{N_2}{\tau_L} - \sigma v_g N_2 n_L + R_p \nonumber \]

\[\dfrac{d}{dt} n_L = -\dfrac{n_L}{\tau_p} + \dfrac{l_g}{L} \dfrac{\sigma v_g}{V_g} N_2 (n_L + 1). \nonumber \]

Figure 4.2: Vier-Niveau-Laser

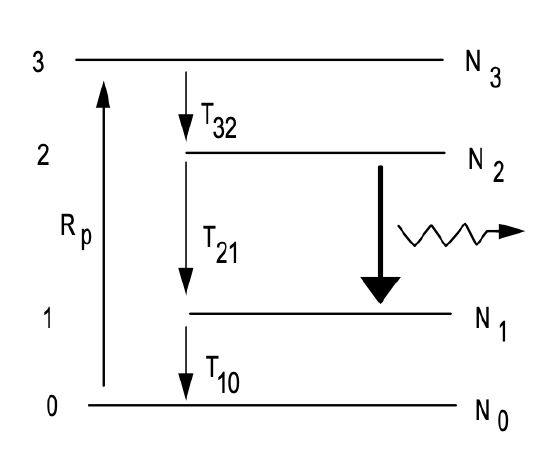
where \(\tau_L = T_{21}\) is the lifetime of the upper laser level. Experimentally, the photon number and the inversion in a laser resonator are not very convenient quantities, therefore, we normalize both equations to the round-trip amplitude gain \(g = \dfrac{l_g}{L} \dfrac{\sigma v_g}{2V_g} N_2 T_R\) experienced by the light and the circulating intracavity power \(P = I \cdot A_{eff}\)

\[\dfrac{d}{dt} g = -\dfrac{g-g_0}{\tau_L} - \dfrac{gP}{E_{sat}} \nonumber \]

\[\dfrac{d}{dt} P = -\dfrac{1}{\tau_p} P + \dfrac{2g}{T_R} (P + P_{vac}), \nonumber \]

with

\[E_s = I_s A_{eff} \tau_{L} = \dfrac{hf_L}{2^* \sigma} \nonumber \]

\[P_{sat} = E_{sat}/\tau_L \nonumber \]

\[P_{vac} = hf_L v_g/2^* L = hf_L/T_R \nonumber \]

\[g_0 = \dfrac{2^* v_g R_p}{2A_{eff} c_0} \sigma \tau_L,\label{eq4.1.14} \]

the small signal round-trip gain of the laser. Note, the factor of two in front of gain and loss is due to the fact, the \(g\) and \(l\) are gain and loss with respect to amplitude. Equation \ref{eq4.1.14} elucidates that the figure of merit that characterizes the small signal gain achievable with a certain laser material is the \(\sigma \tau_L\)-product.

Table 4.1: Wavelength range, cross-section for stimulated emission, upper- state lifetime, linewidth, typ of lineshape (H=homogeneously broadened, I=inhomogeneously broadened) and index for some often used solid-state laser materials, and in comparison with semiconductor and dye lasers.

Laser Medium	Wave-length \(\lambda_0\) (\(n\)m)	Cross Section \(\sigma\) (\(\text{cm}^2\))	Upper-St. Lifetime \(\tau_L\) (\(\mu\) s)	Linewidth \(\Delta f_{FWHM} = \tfrac{2}{T_2} (\text{THz})\)	Typ	Refr. index \(n\)
\(\text{Nd}^{3+}: \ce{YAG}\)	1,064	\(4.1 \cdot 10^{-19}\)	1,200	0.210	H	1.82
\(\text{Nd}^{3+}: \ce{LSB}\)	1,062	\(1.3 \cdot 10^{-19}\)	87	1.2	H	1.47 (ne)
\(\text{Nd}^{3+}: \ce{YLF}\)	1,047	\(1.8 \cdot 10^{-19}\)	450	0.390	H	1.82 (ne)
\(\text{Nd}^{3+}: \ce{YVO4}\)	1,064	\(2.5 \cdot 10^{-19}\)	50	0.300	H	2.19 (ne)
\(\text{Nd}^{3+}: \ce{glass}\)	1,054	\(4 \cdot 10^{-20}\)	350	3	H/I	1.5
\(\text{Er}^{3+}: \ce{glass}\)	1,55	\(6 \cdot 10^{-21}\)	10,000	4	H/I	1.46
Ruby	694.3	\(2 \cdot 10^{-20}\)	1,000	0.06	H	1.76
\(\text{Ti}^{3+}: \ce{Al2O3}\)	660-1180	\(3 \cdot 10^{-19}\)	3	100	H	1.76
\(\text{Cr}^{3+}: \ce{LiSAF}\)	760-960	\(4.8 \cdot 10^{-20}\)	67	80	H	1.4
\(\text{Cr}^{3+}: \ce{LiCAF}\)	710-840	\(1.3 \cdot 10^{-20}\)	170	65	H	1.4
\(\text{Cr}^{3+}: \ce{LiSGAF}\)	740-930	\(3.3 \cdot 10^{-20}\)	88	80	H	14
He-Ne	632.8	\(1 \cdot 10^{-13}\)	0.7	0.0015	I	~1
\(\ce{Ar+}\)	515	\(3 \cdot 10^{-12}\)	0.07	0.0035	I	~1
\(\ce{CO2}\)	10,600	\(3 \cdot 10^{-18}\)	2,900,000	0.000060	H	~1
Rhodamin-6G	560-640	\(3 \cdot 10^{-16}\)	0.0033	5	H	1.33
semiconductors	450-30,000	~ \(10^{-14}\)	~0.002	25	H/I	3-4

The larger this product the larger is the small signal gain (\(g_0\)) achievable with a certain laser material. Table 4.1

From Eq.(2.4.7) and (\(\ref{eq4.1.4}\)) we find the following relationship between the interaction cross section of a transition and its microscopic parameters like linewidth, dipole moment and energy relaxation rate

\[\sigma = \dfrac{hf_L}{I_{sat} T_1} = \dfrac{2T_2}{\hbar^2 Z_F} \dfrac{|\vec{M} \hat{\vec{E}}|^2}{|\hat{\tilde{E}}|^2}.\nonumber \]

This equation tells us that broadband laser materials naturally do show smaller gain cross sections, if the dipole moment is the same.