31.9: Thermodynamics

A SOFC is an electrochemical device that converts chemical energy of the fuel and oxidant directly and reversibly into electrical energy. They are not a better or improved heat engine, they are fundamentally different. Hydrogen and oxygen are going to be used to illustrate the thermodynamics of the fuel cell.

The ideal reversible SOFC – Basic derivation of potential and efficiency

The first and second law of thermodynamics describe the reversible SOFC. The reactants (fuel and air) deliver total enthalpy \( \sum n_iH_i \) and the total enthalpy \( \sum n_jH_j \) leaves the fuel cell, so the change in enthalpy, \( \Delta H = \sum n_jH_j - \sum n_iH_i\). The heat q_FC has to be extracted from the fuel cell and the reversible work w_FC delivered.

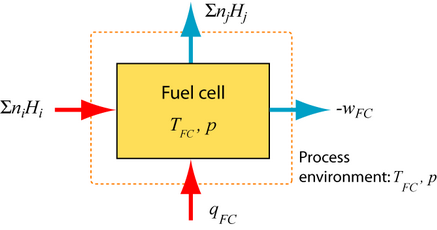
1^st Law of thermodynamics:

\[ q_{FC} + w_{FC} = \Delta H \]

2^nd Law of thermodynamics:

\[ \oint dS = 0 \]

The reaction entropy has to be compensated by the transport of heat to the environment:

\[ \Delta S - \frac{q_{FC}}{T_{FC}} \]

The reversible work is from the above equations:

\[ \omega_{FC} = \Delta H - T_{FC} \cdot \Delta S \]

The Gibbs enthalpy is equal to the reversible work of the reaction. The reversible efficiency is equal to the ratio of the Gibbs and the reaction enthalpy:

\[ \nu_{FC} = \frac{\Delta G}{\Delta H} = \frac{\Delta H - T_{FC} \cdot \Delta S}{\Delta H} \]

The fuel cell is an electrical device, for which the processes can be fully described by thermodynamic principles.

Hydrogen is absorbed at the anode, it is ionized and the electrons are conducted away to do useful work. Oxygen atoms that arrive at the cathode are ionized by the electrons coming from the anode. The protons and oxide ions react and form water.

Anode: H2 ↔ 2H^+ + 2e^– Cathode: ½O2 + 2e^–↔ O^2– The net reaction is: 2H^+ + O^2–↔ H2O

This shows that the molar flow of hydrogen is twice the molar flow of oxygen. The electric current is therefore:

\[ I = \dot n_{el} \cdot (-e) \cdot N_A = -2 \dot n_{H_2} \cdot F \]

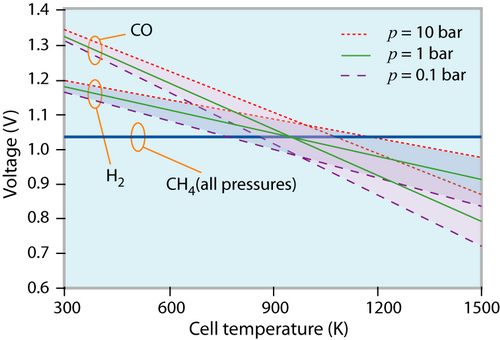
The reversible cell voltage of different fuels at different states (p, T) of the environment

The electric current is a measure of the rate at which fuel is spent. The electric and thermodynamic quantities are matched by considering reversible power:

\[ P = V \cdot I = \dot n_{H_2} \cdot \omega= \dot n_{H_2} \cdot \Delta G \]

Hence the reversible voltage:

\[ V = \frac{- \dot n_{H_2} \Delta G}{\dot n_{el} \cdot F} = \frac{- \Delta G}{\dot n_{el} \cdot F} \]

Using the assumption that we have (near) ideal gases, a more accurate equation for the Gibbs energy is:

\[ \Delta G(T,p) = \Delta H(T) - T \cdot \Delta S(T,p) \]

where

\[ S(T,p) = S^0 + \int{T_0}^{T} \frac{C_p(T)}{t}dt - R \cdot ln(\frac{p}{p_0}) \]

We get for the Gibbs energy:

\[ \Delta G(T,p) = \Delta G(T) + T \cdot R \cdot ln(K) \]

where K is the equilibrium constant.

We get from the above analysis the Nernst potential:

\[ V_N = \frac{- \Delta G(T)}{n_{el} \cdot F} - \frac{R \cdot T \cdot ln(K) }{n_{el} \cdot F}\]

Going further: The chemical thermodynamics of SOFCs.