Superconductivity

Superconductivity is a phenomena in certain metals and ceramics where the resistivity of the material drops to zero below a certain critical temperature known as T_C. These temperatures are low, with most industrial superconductors below 12 K and higher temperature ceramics just above 130K. Superconductors have widespread applications because of their properties, such as MRI machines, power lines, MagLev trains, and strong electromagnets for research.

Introduction

Due to its cryogenic nature, superconductivity was not discovered until Dutch physicist Heike Kamerlingh Onnes was able to liquefy Helium using the Hampson-Linde cycle in 1908. With a boiling point of 4K at ambient pressure, Liquid helium allowed Onnes to experiment with very cold temperatures. He began measuring resistivity at these low temperatures to see if resistivity decreased or increased at low temperatures. It was known that resistivity decreases with temperature, the behavior was unknown as it approached 0K. In April 1911, Onnes measured resistivity of Mercury wire and the resistance dropped to 0 suddenly at about 4K. He later won the 1913 Nobel Prize for his work.

There are two types of superconductors. Type I is usually metallic and tend to have a very low T_C, usually on the order of around 10 K or below. The physical mechanism of how these work is described by BCS theory. Type II include High Temperature Superconductors and can be ceramics or metals, and can have critical temperatures up to about 130 K. Up to this point, there is no successful theory for the mechanism behind Type II superconductivity, though there are some experimentally verified similarities. There are enough differences that they are almost certainly different.

Superconductors have the major advantage that their resistivity is zero, allowing for persistent, large current flow and without heating the wire. This can be used to create the very strong magnetic coils in MRI imaging machines (see fig 1), and the strong magnetic fields in scientific testing equipment, colliders, or any application that requires a high permanent magnetic field. They can also move electric power around with zero resistance, making them very desirable for power cables and efficient devices.

The main drawback to using superconductors is the very low operating temperatures at which they need to be held. Type I superconductors are typically used because they are metallic allowing them to be shaped into wires for coils, and because they have a high critical current density so they can carry more current for the cross sectional area of the wire. Unfortunately, liquid helium (a non-renewable, expensive resource) or newer, energy-heavy, cryogen-free techniques need to be used to keep these materials below their operating temperatures which are typically 8-12 K. Liquid Nitrogen can cool high temperature ceramic superconductors such as YBCO below T_C, but they are limited by how well they can be shaped and how much current they can carry.

Figure \(\PageIndex{1}\): An MRI machine.

BCS Theory Mechanism

To this day the most well received theory was published in 1957 by J. Bardeen, L.N. Cooper, and J. R. Schrieffer who received a Nobel Prize in 1972. On a purely conceptual level this theory explains superconductivity as a way that electrons move through a material. Essentially, in any material two electrons can pair up with the help of a phonon, or physical/vibration wave. This is a long distance interaction and in Type I superconductors this can be around 100nm also known as the coherence length. In high temperature superconductors, this is observed as well, albeit with much shorter interaction lengths. Above the critical temperature, these appear but are quickly broken by thermal vibrations in the material. At very low temperatures the thermal vibrations are suppressed to the point where these phononic interactions can be maintained for long periods of time, and through this quantum mechanical interaction, the two electrons and the phonon form what is called a Cooper Pair.

Physically speaking, it can be imagined that in a crystalline material, a leading electron can travel through a row of atoms and the positive nuclei are attracted to the passing electron. This causes a ripple in the crystal lattice where nuclei are pulled slightly closer together, leaving a slightly positive local charge. A second electron is attracted to this local positive charge and is attracted to this area exactly cancelling out the energy lost by the electron that created this positive area.

Electrons are fermions with half integer spins meaning that no two can occupy the same state at the same time, and thus interact with each other. When these two half integer spins combine in a Cooper Pair, they create an integer spin meaning that a Cooper Pair is a Boson. Bosons are allowed to occupy the same state as another boson, allowing them to pass through each other with no resistance. Another property of Cooper pairs is that the wave functions of the two electrons are phase locked, which is important to their formation.

The fact that Bosons can occupy the same state does a very interesting thing to the density of states. Based on Band Theory, in a normal metal electrons will fill up in an increasing density of state up to the Fermi level. In superconductors, the bosonic nature of the Cooper Pairs allow them to occupy lower states than before the material went through its superconducting transition temperature. The implication here is that there is an energy gap below the Fermi level in superconductors.

Known Superconductors

Chart of some known superconductors and Tc-Coming soon.

The most common engineering superconductors are Nb3Sn and Nb-Ti. They are both metallic so they are malleable, have high critical currents and high critical field densities. Their T_C is only about 9K however, so they need to be cooled with liquid nitrogen. The most talked about high temperature superconductor is YBa2Cu3O7-x or YBCO. It was the first superconductor discovered that had a T_C above liquid nitrogen (77K) with a T_C of 92K. It is commonly used to demonstrate the Meissner Effect in classrooms and a power cable was built out of it in New York.

Electric and Tunneling Properties

SQUIDs and Josephson Junctions

Beyond the zero resistance that superconductors offer, there are some additional properties that can be exploited for various purposes. One of the more common superconducting devices is known as a SQUID or Superconducting QUantum Interferance Device. A SQUID is capable of measuring magnetic moment of a sample down to one quanta of magnetic moment. This is only possible because superconductors have no resistance.

A SQUID works by taking a specifically shaped test coil of superconducting wire and attaching this coil to a Josephson junction which is essentially an interruption in the superconducting wire or thin insulating gap. This sets up an electron tunnel junction between two superconductors. Because Cooper pairs act as a single particle, these pairs will have to pass through the junction together while remaining phase locked. When there is a voltage across a Josephson junction, there is an oscillation that can be measured that is proportional to the voltage:

\[F_{Josephson} = \frac{2e\Delta V}{h}\]

Andreev Reflection

Since Cooper Pairs are made up of two electrons, it follows that when electrons pass from a normal conductor through an insulator into a superconductor (NIS junction) that a Cooper pair will appear on the superconducting side.

This has to occur because a single electron cannot exist in the aforementioned superconducting band gap. There are several things that can happen, but one process is known as Andreev Reflection. One electron approaches the junction from the metal and tunnels through, and a Cooper pair emerges on the superconducting side. To maintain both charge and charge carrier momentum a hole has to appear on the normal conducting side (see Fig 2) This effect can be measured with an experiment called Point Contact Spectroscopy, where voltage is varied across a NIS junction. This process is spin polarization dependent and can be used to measure the spin polarization of the metal.

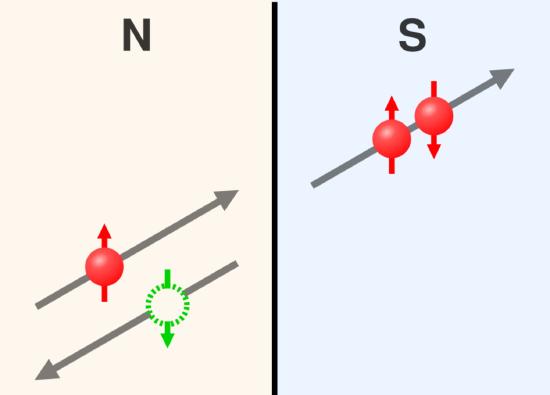
Fig. 2. Andreev Reflection, where a hole is ejected from a tunnel junction.

Magnetic Properties and Uses

Magnetic Coils

The most common demand of superconductors today is to use them for their magnetic properties. The most common use is in magnetic coils because the lack of resistance and large density of states allows us to pass a large current through a coil making a very strong electromagnet with relatively low energy. Once the current is up to speed it is simple to close the loop and the coil will maintain that magnetization as long as it remains cold. This can be used in an MRI machine where the spins of hydrogen atoms can be aligned in the body and then can be measured with radio frequencies to image the human body.

Superconductors do suffer a critical current and critical field density however, so if the field or the current goes too high a magnet can “quench.” This process is a rapid heating of the coil as the huge current is immediately converted into resistive heat, heating the liquid helium around it and usually causing a major pressure increase. This can be dangerous without a proper blow off valve, so care must be taken when setting up a superconducting magnet.

Meissner Effect

A very interesting property of superconductors has to do with the fact that Type I superconductors expel all magnetic field. This is known as the Meissner Effect. A magnetic field is produced by motion of electrons, and likewise a magnetic field can cause electrons to move. In normal metals we know that eddy currents occur around magnetic field lines and can cause local flows that quickly die out due to resistive heating. In a superconductor, there is no resistance so the Eddy Currents are allowed to perpetually flow. They happen to flow exactly opposite to the magnetic field lines cancelling them out in the superconductor. In Type II superconductors, some magnetic field lines can penetrate, however they are pinned to certain locations in the superconductor, and this effect is preserved.

Because these fields are exactly canceled out it actually allows a permanent magnet to be pinned in place by the superconductor as long as the magnetic flux remains the same. This is shown in figure 3. That is to say, it can float in a set position indefinitely. If a track is set up such that the magnetic flux remains the same at all points along the track, a traveling superconducting puck can move along this path at a set altitude. This is the basic concept for superconducting MagLev trains. Because there is no physical contact, this low resistance allows trains to be more efficient. There are also methods of propelling the train with magnetism which increases efficiency further.

Figure 3. Meissner effect above a high TC superconductor.

Questions

1. What are the most common uses for superconductors and why are they important?

2. What is a Cooper pair, and why is it important to superconductivity?

3. What is the voltage measured from a Josephson junction oscillating at 483.6 GHz?

Answers

1. Magnetic coils and research. Superconductors allow us to maintain a strong magnetic field with very little energy expenditure. In the future, high temperature superconductors could make trains, power lines and everyday devices much more efficient.

2. A Cooper pair, is a pair of electrons with a phononic interaction. The electrons are phase locked and it is a boson allowing it to occupy the same state as other cooper pairs. This creates a band gap below the Fermi energy and allows resistance-free flow through a material.

3. Exactly 1 volt. In fact, it is the standard for what 1 volt is.

Additional Links

Band Theory

Resistivity

Band Theory of Metals and Insulators

References

[1] Hummel, Rolf E. (2012). Electronic Properties of Materials. New York, NY: Springer, 2012.

[2] J. Bardeen, L. N. Cooper, and J. R. Schrieffer, "Theory of Superconductivity", Phys. Rev. 108, 1175 (1957)

[3] J. Bardeen. "Theory of the Meissner Effect in Superconductors". Physical Review 97 (6): 1724–1725.Bibcode:1955PhRv...97.1724B.

[4] R. Feynman “The Schrödinger Equation in a Classical Context: A Seminar on Superconductivity.” Feynman Lectures (2010)

Contributors and Attributions

Chris Hart, Chemical Engineering and Materials Science Department, UC Davis