6.2.5: Turbine

Last updated
Save as PDF

Page ID: 78142

\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \) \( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)\(\newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\) \( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\) \( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\) \( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\) \( \newcommand{\Span}{\mathrm{span}}\) \(\newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\) \( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\) \( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\) \( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\) \( \newcommand{\Span}{\mathrm{span}}\)\(\newcommand{\AA}{\unicode[.8,0]{x212B}}\)

The turbine is located downstream the combustor and transforms the energy from the hot flow into mechanical work to move the compressor (remember that turbine and compressor are linked by a shaft). The turbine is composed of two rows of small blades, one that rotates at very high speeds (the rotor) and the other that remains stationary (the stator). The blades experience flow temperatures of around \(1400^{\circ} K\) and must, therefore, be either made of special metals (typically titanium alloys) that can withstand the heat.

Depending on the engine type, there may be multiple turbine stages present in the engine. Turbofan and turboprop engines usually employ a separate turbine and shaft to power the fan and gearbox, respectively, and are referred to as two-spool engines. Three- spool configurations exist for some high-performance engines where an additional turbine and shaft power separate parts of the compressor.

The derivation of equations that govern the evolution of the air flow over the turbine are similar to those already exposed for the compressor. As the flow passes through the turbine, pressure and temperature decrease. The decrease in pressure through the turbine is quantified with the so-called turbine pressure ratio (TPR), i.e., the ratio of the exiting to the entering air pressure in the turbine. Using the station numbers of Figure 6.2, the TPR is equal to the stagnation pressure at point 5 (\(p_{5t}\)) divided by the stagnation pressure at point 4 (\(p_{4t}\)), i.e.,

\[TPR = \dfrac{p_{5t}}{p_{4t}}.\nonumber\]

截屏2022-01-21 下午8.40.34.png
Figure 6.8: Turbine and schematic blade.

Given that the process can be considered adiabatic, pressure and temperature are related as in Equation (6.2.1.9) so that:

\[TPR = \dfrac{p_{5t}}{p_{4t}} = \left (\dfrac{T_{5t}}{T_{4t}} \right )^{\tfrac{\gamma}{\gamma - 1}}.\]

Again, referring the reader to Section 6.2.1 and doing some algebraic operations, the turbine mechanical work \(W_{turb}\) can be expressed as follows:

\[W_{turb} = (\eta_t c T_{4t}) (1 - TPR^{\tfrac{(\gamma - 1)}{\gamma}}),\label{eq6.2.5.2}\]

where \(c\) is the specific heat of the gas and \(\eta_t\) is the turbine efficiency.

Compressor and turbine stages work attached one to the other. This relationship can be expressed by setting the work done by the compressor equal to the work done by the turbine, i.e., \(W_{turb} = W_{comp}\). Hence, the conservation of energy is ensured. Equating Equation (6.2.3.1) and Equation (\(\ref{eq6.2.5.2}\)) yields:

\[\dfrac{cT_{2t}}{\eta_c} (CPR^{\tfrac{(\gamma - 1)}{\gamma}} - 1) = (\eta_t c T_{4t}) (1 - TPR^{\tfrac{(\gamma - 1)}{\gamma}}).\]

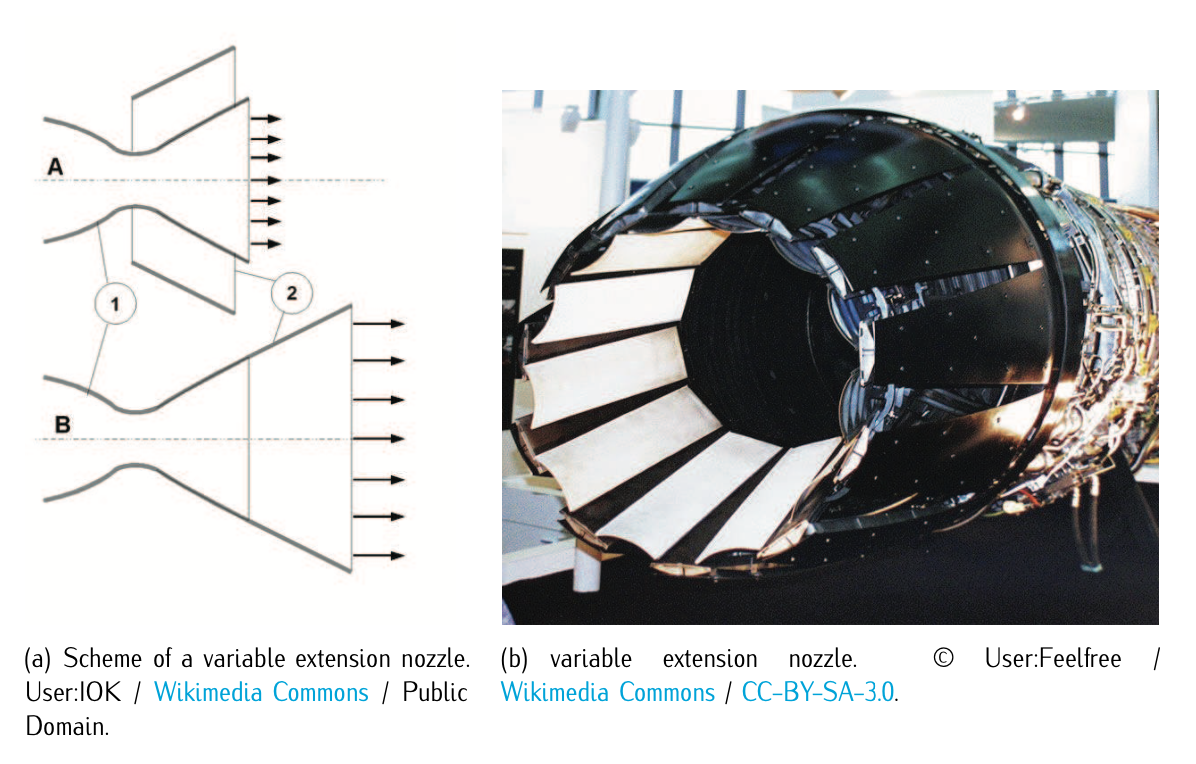
截屏2022-01-21 下午8.46.55.png
Figure 6.9: Variable extension nozzle.