Multi-junction Photovoltaics

Solar technology is becoming more ubiquitous in the modern world; as solar panels utilize the sun as an unlimited free energy source, they are being used on building roofs, car parks, homes, and there are even solar farms that produce energy like a common power plant. Traditional solar cells use silicon as the semiconducting material to form the pn junction that allows the cell to absorb light and turn it into electrical energy; these cells are known as single-junction photovoltaics. These achieve approximately 20% efficiency [1]. To increase this efficiency, multiple junction, or multi-junction, cells have been produced. These cells utilize multiple pn junctions, using a wide variety of materials, to more efficiently harvest a wider range of light, optimizing the electric potential of each photon that is absorbed. As shown in Figure 1, the photovoltaics that lead the industry in efficiency are multi-junction, while the standard single-junction cells achieve a little over half the multi-junction.

Figure \(\PageIndex{1}\): Efficiencies of various solar cells as they change over time and with new developments (wikicommons).

Operation and Band Gap Energy

To understand how a multi-junction cell operates, one must first understand the operation of a single-junction photovoltaic. The ability for a single-junction photovoltaic to absorb light comes from the pn junction created by the semiconductor. The semiconductor creates a pn junction by the combination of both a p-type and an n-type semiconducting layers. The n-type semiconductor has extra electrons, while the p-type has an absence of electrons, which creates holes [2]. Between these two layers, at the junction, there is a depletion zone which the elections cross, without an external source of energy. This external source comes in the form of photons, which, when absorbed by an electron, can excite the electron across the junction. To return the system to equilibrium, the electron will try to flow back across the junction, which can be done through an external current path where the electron's movement can be used to do work [1]. The energy needed to excite the electron across this junction, or gap, is the band gap energy, E_g.

Only certain frequencies of light have enough energy to excite electrons across the band gap. The energy of each photon is governed by the de Broglie equation

\(E=h\nu=hc/\lambda\)

Light that has a energy equal to or higher than Eg can excite the electrons across the junction; however, light that has lower energy, will pass through. Therefore, a material with a low band gap energy can utilize more light in the electromagnetic spectrum. Consequently, a photon with higher energy than E_g will not create a larger voltage than one with equal energy to the band gap. The size of the band gap dictates the energy generated by exciting an electron. A smaller band gap will result in lower energy; therefore, there is a balance between having a small band gap that will utilize many photons and having a large band gap that will utilize fewer photons, yet generate more energy from them. The optimum band gap is at approximately 1.4 eV and the theoretical limit of a singl e junction cell with this E_g is approximately 31% [3]. The actual efficiency and theoretical efficiency are greatly improved on with the addition of multiple pn ju nctions and therefore multiple band gaps. With various band gaps, the higher energy photons can be better utilized by materials with large band gaps while the lower energy photons can be utilized by those with smaller gaps. This is the basic principle that drove the creation of a multi-junction cell. A multi-junction cell layers the materials in descending order, with the largest band gaps on top and smallest on the bottom, which creates a "photon sorting" effect [4]. Photons with high energy are absorbed by the top layer and utilized more full y than if they were absorbed by the bottom layers, while lower energy photons pass through. Higher energy photons of the ones that are transmitted through the top layer are then absorbed by the middle layer, while the even lower energy photons continue to pass through. Through this process, the same amount of light that would have been absorbed by the lowest layer is still absorbed, yet higher energy photons are better utilized to generate energy. Figure 2 demonstrates this stacking order.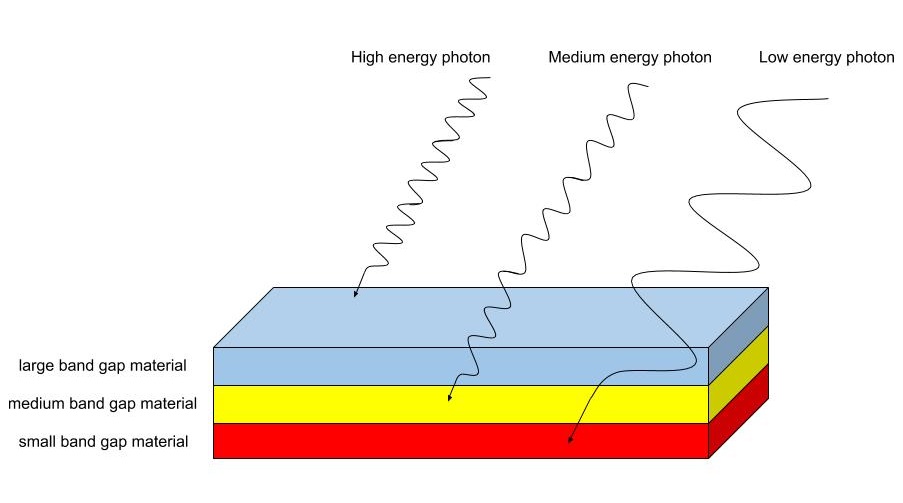

Figure 2: Light of lower energy passes through the thin film semiconductor, where it then can excite electrons across the bandgaps of smaller band gap materials. This image demonstrates how the stacking order of the material "sorts" the photons into different energy groups. (original image)

Materials

The materials that go into a photovoltaic cell make a large difference on the cell's efficiency, as the band gap varies based on the materials and the dopants within the material that make the pn junction. For traditional single-junction cells, monocrystaline silicon is used, as it is abundant and relatively cheap; in addition it has a gap of 1.11 eV, quite close to the optimal 1.4 eV [5, 1]. For multi-junction cells, he most common material used is Gallium Arsenide, GaAs, as it has a band gap of 1.43 eV, which is extremely close to the optimal band gap range. As the optimum material, GaAs generally is in the middle layers, commonly in between Indium Gallium Phosphide and Germanium, with band gaps of 1.85eV and 0.67eV respectively [4]. GaInP utilizes the high energy photons while Ge utilizes the much lower energy photons and GaAs utilizes those in between. The entire list of semiconducting materials and band gaps can be found here.

Addition of new materials:

Multi-junction cells increase their efficiency over single-junction cells with the addition of each new material, from which a new junction is added. The added material either increases the range of photons that can be absorbed or better absorbs the energy from photons of higher energy. Therefore, increasing the number of junctions increases the efficiency of the cell [4]. This does however have an upper limit. The increase in film thickness decreases the transmittance of light, and photons may not make it to the bottom layers of the cell. The inverse of this also has a limit; if the films are too thin, they will transmit too much light and will not absorb all the photons they can utilize. Most multi-junction cells utilize 3 materials [4, 6].

Efficiency:

Multi-junction cells were invented in the effort to produce more efficient solar cells, however, there are still many factors that effect the efficiency of the cell. Efficiency can vary with the amount of equivalent suns the cell is exposed to, the crystalline structure of the layers, and the structure of adjacent layers. Concentrators are a series of lenses and/or mirrors placed above and around the cell to focus the sun's light into the cell, which exposes the cell to much larger amounts of usable light; this can be up to "300 suns" which is 300 times the sun's radiation [7]. Multi-junction cells are primarily paired with concentrators, over silicon cells, as they can operate under the elevated temperatures that are produced from them [8]. The crystalline structure of each layer of material is also vital to efficiency, as monocrystalline structures are proven to be more efficient than multicrystalline, and even amorphous structures [9]. A final property of multi-jucntion cell efficiency is the lattice structure of adjacent layers. Layers can either be lattice-matched or lattice-mismatched, as shown in Figure 3. The mismatch of the lattices produces a lower band gap as opposed to that of the matched lattices [6]. The decrease in the band gap leads to an increase in photon absorption and increases efficiency of the photovoltaic cell.

Figure 3: This represents the a) lattice match, and b) lattice-mismatch between two semiconducting materials in a multi-junction photovoltaic. (original image)

Questions and Answers

Questions

1. Between these three materials: Gallium Arsenide, Germanium, and Boron Arsenide, , which can utilize yellow light to create energy in a solar cell? (hint, use this chart)
2. What is should the stacking order be of a multi-junction cell that is made from Indium Arsenide, Gallium Phosphide, Indium Phosphide, and Gallium Arsenide? Use the same chart from #1.
3. If a concentrator is added to the above cell from #2, but one material was removed, which material should be removed to still optimize the number of incident photons?

Answers

1. All of them! Yellow light averages at 570nm which corresponds to an energy of 1.63eV; this is above the E_g of all three materials and therefore can excite the electrons across the gap.
2. The stacking order should go in decreasing order in band gap energy; therefore it is: Gallium Phosphide, Gallium Arsenide, Indium Phosphide, Indium Arsenide.
3. Gallium phosphide should be removed. This material can better utilize high energy photons, yet lower band gap materials will be able to absorb more photons.

References

1. Toothman, Jessika, and Scott Aldous. "Anatomy of a Solar Cell1." HowStuffWorks. HowStuffWorks.com, 1 Apr. 2000. Web. 07 Dec. 2015.
2. Honsberg, Christina, and Stewart Bowden. "Doping." Doping. PVEducation, n.d. Web. 16 Oct. 2015.
3. Henry, C. H. "Limiting Efficiencies of Ideal Single and Multiple Energy Gap Terrestrial Solar Cells." AIP. Journal of Applied Physics, 18 Sept. 1980. Web. 7 Dec. 2015.
4. D. Friedman, “Progress and Challenges for Next-Generation High-Efficiency Multijunction Solar Cells," Elsevier, Vol. 14, no. , pp. 131-138, 2010.
5. "Band Gap." Wikipedia. Wikimedia Foundation, 16 Nov. 2015. Web. 08 Dec. 2015.
6. H. Cotal, C. Fetzer, 1st Initial. Et al, "III-V Multijunction Solar Cells for Concentrating Photovoltaics," Energy Environ. Sci., Vol. 2, no. , pp. 174-192, 2009.
7. F. Akarslan, "Photovoltaic Systems and Applications," Modeling and Optimization of Renewable Energy Systems, Vol., no. , pp. 32-33, 2012.
8. "Concentrator Photovoltaics." Wikipedia. Wikimedia Foundation, 30 Nov. 2015. Web. 8 Dec. 2015.
9. Hans-Wemer Schock, " Prospects of Thin Film Solar Cells: Towards Competitive Efficiencies," Helmholtz-Zentrum-Berlin für Materialien und Energie GmbH, pp. 1-8, 2012.